Change of temperature/thermal energy in a collision between two objects in an ideal condition

Question

As far as I know thermal energy is a part of internal energy which includes kinetic energy of particles of a body with respect to its center of mass. And temperature is related to this thermal energy.

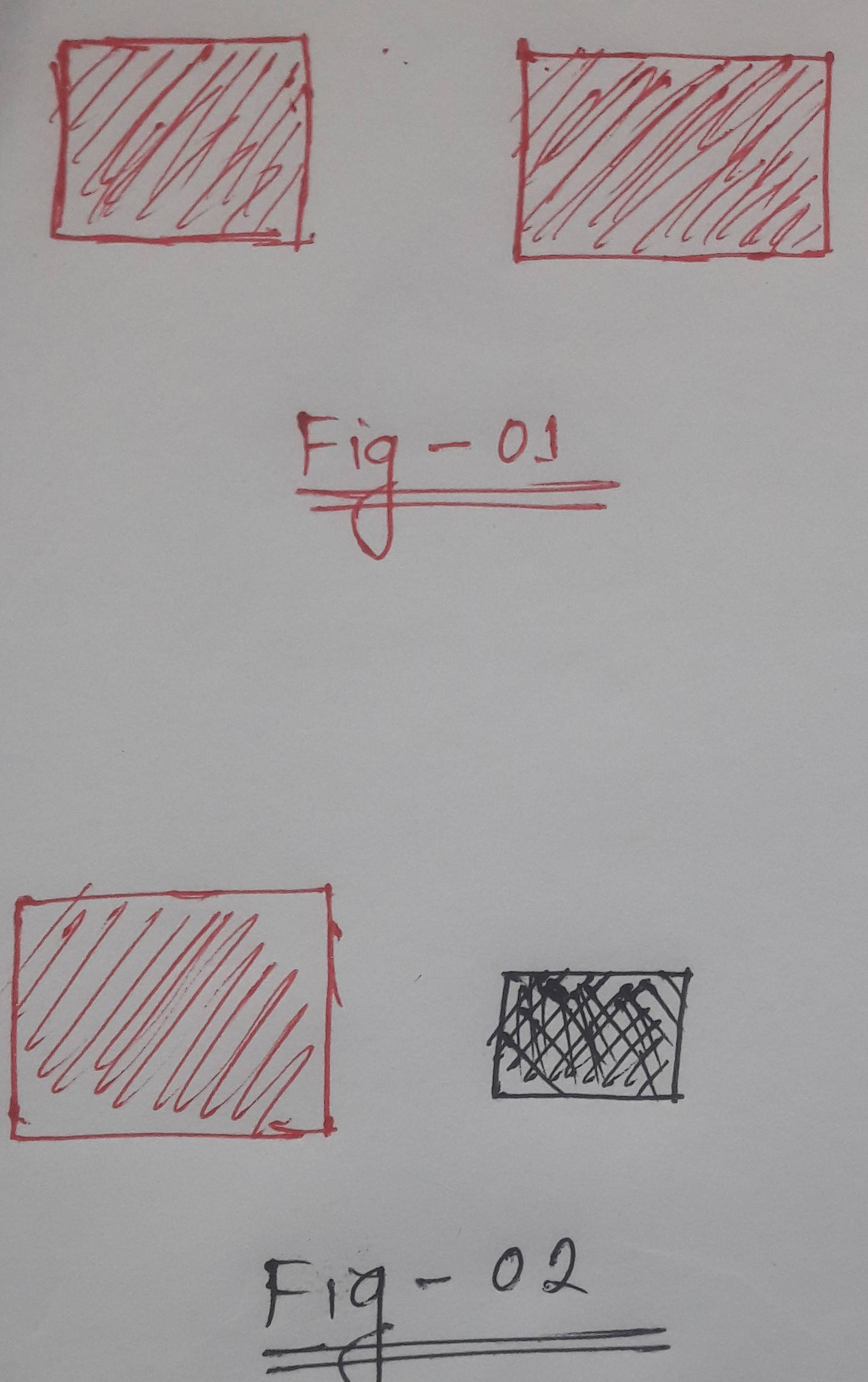
If two solid bodies(one at rest) of equal size collides in an ideal condition(no friction or dissipation of energy) such that two surfaces of two bodies which collide are exactly of same shape, then that collision should be elastic. Considering that the surfaces of collision are of similar shape and that is why the force is unformly distributed all over the surface which does not cause any change in internal microscopic motion of particles.

But what if the two objects are of different sizes as shown in the image(fig-02) below? If we consider that the smaller one is in motion and it collides with the larger one, will the force applied on the surface of larger one be uniform? Or will it be for some moment non uniform such that a small part of gets slighlty dispaced in the direction of force and causes internal particles in that portion to dissipate energy due to collision with the surrounding particles? If the later case occurs, will it change the temperature ot total thermal energy of the larger body?

I am refering to such a condition as if the collision is taking place in space where ther is no air resistance, no friction.... That is no means of energy loss.

Answer 1

If two solid bodies(one at rest) of equal size collides in an ideal condition(no friction or dissipation of energy) such that two surfaces of two bodies which collide are exactly of same shape, then that collision should be elastic.

The fact that the two surfaces of the bodies which collide are the same shape or not the same shape doesn't necessarily mean the collision is elastic or inelastic. For example a collision between two identically shaped objects made of putty that stick together will not be elastic. The elasticity of the collision depends more on the elastic behavior of the material or object involved, in addition to its shape.

In this regard, perfectly elastic behavior of an object is often modeled by an ideal spring. The force required to extend or compress the spring is proportional to the amount of extension or compression, the proportionality constant being the spring constant. An ideal spring undergoes deformation with no dissipation of energy. A body exhibiting such behavior would not experience a temperature increase during a collision.

Completely inelastic behavior is often modeled by an ideal dashpot. The force required to extend or compress the dashpot is proportional to the rate of deformation, where the proportionality constant is the damping coefficient and is due to viscous friction. The kinetic energy of colliding bodies exhibiting this behavior is completely converted into internal kinetic energy, with resulting temperature increase.

In between the two extremes we have partially inelastic, or viscoelastic, behavior. This behavior is modeled by various combinations of ideal springs and ideal dashpots

Of course the shapes of the colliding objects of identical materials can lessen or increase the degree of inelastic deformation. The greater the force per unit area, all else being equal, the greater the stress and degree of deformation.

The above said, it should be realized that, at the macroscopic scale, there is no such thing as a perfectly elastic collision. There will alway be some inelastic deformation resulting in kinetic energy being dissipated as friction, accompanied by some increase in temperature.

Hope this helps.