NEWTON'S LAWS OF MOTION:
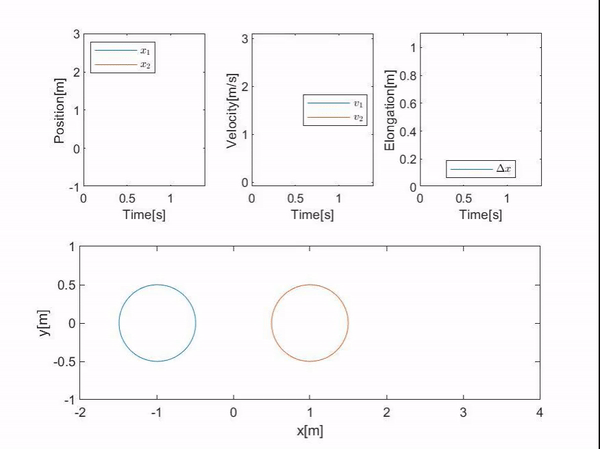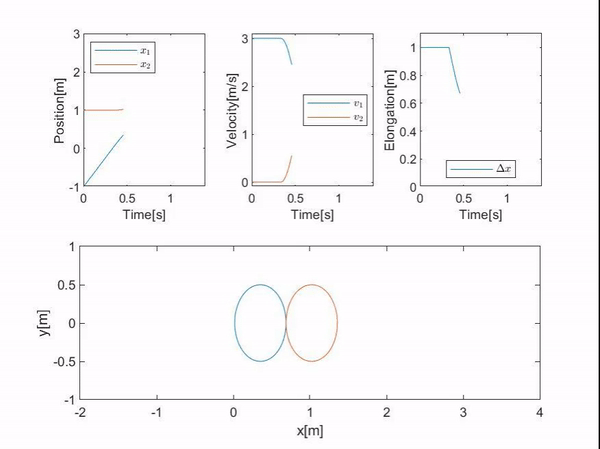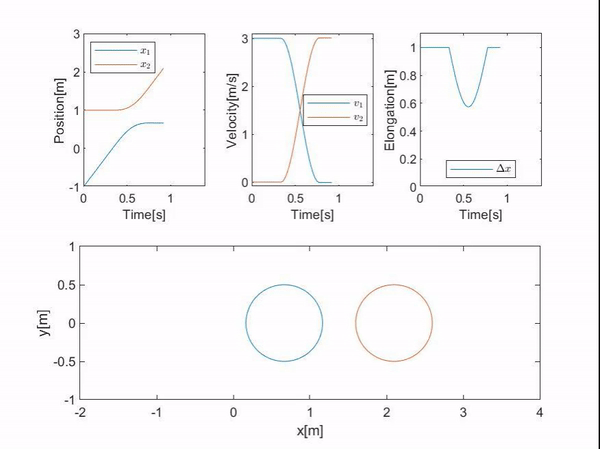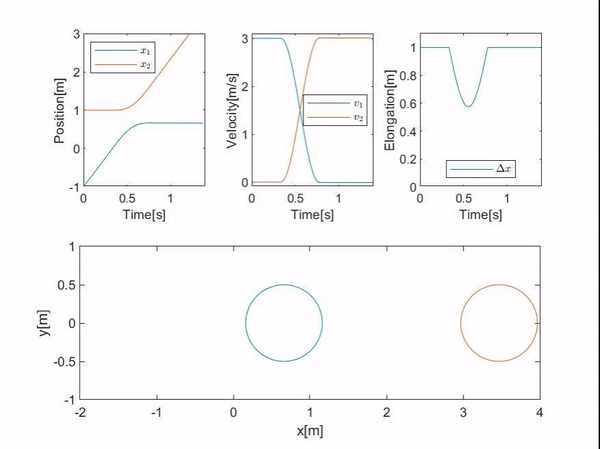
It can be shown for a perfectly elastic head on collision involving identical objects, strictly using Newton's laws of motion, Object 1 will come to a stop and Object 2 will continue with the initial velocity of Object 1.
We can consider the two colliding objects as a system. Given no friction forces, and the fact that the gravitational force on each object is balanced by the normal reaction force of the surface, then from Newton's second law the net external force $F_{net}$ acting on the COM of the system is
$$F_{net}=MA=0$$
Where
$M=m_{1}+m_{2}=2m$ = the center of mass
$A=0$ = the acceleration of the center of mass
and therefore
$V_{COM}$ = the velocity of the COM of the system (average of the velocities of the two objects) and is constant. Before the collision, we know that
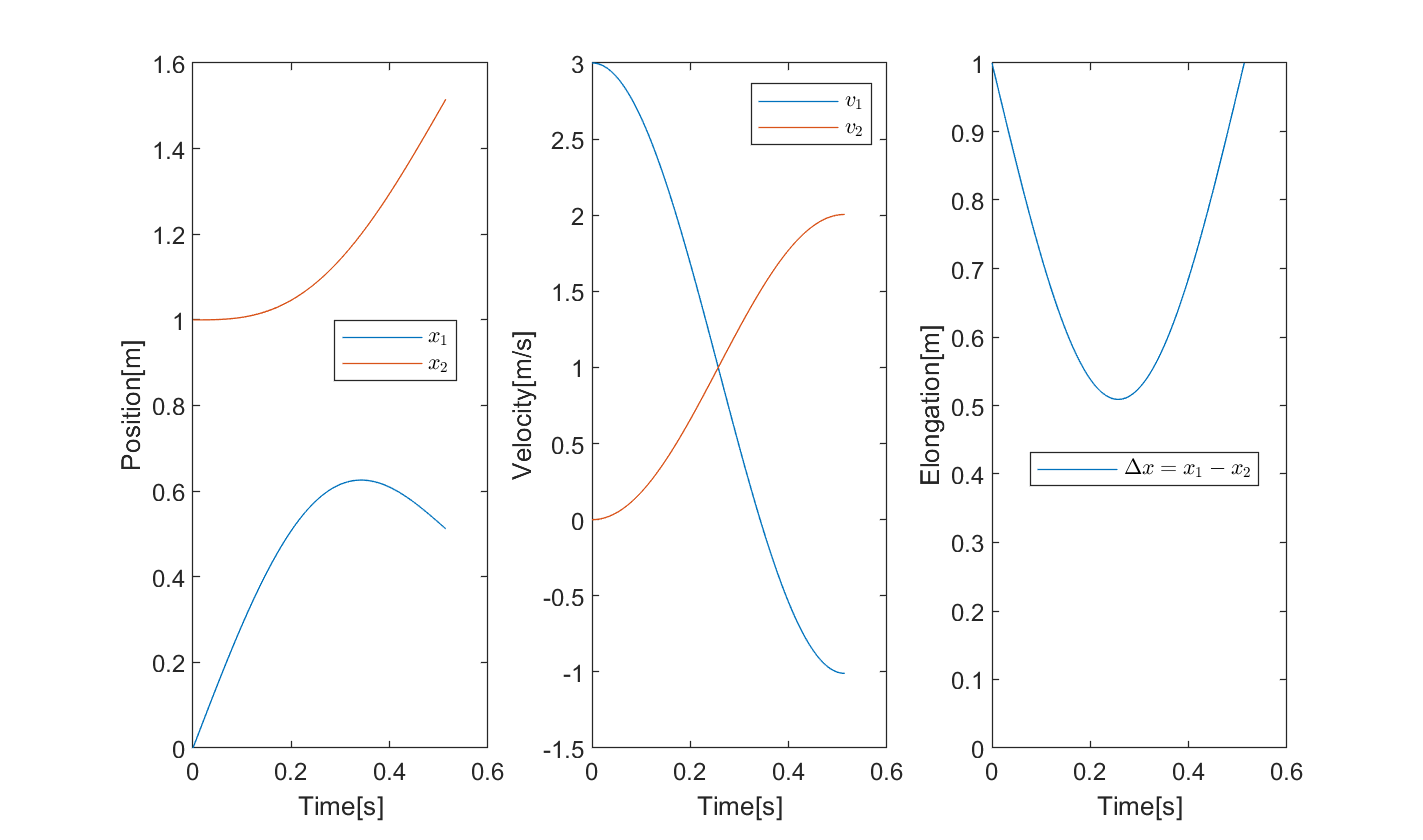
$$V_{COM}= \frac{v_{1}+v_2}{2}=\frac{v_1}{2}$$
And that this velocity remains constant during and after the collision.
While the objects are in contact during the collision, elastic deformation of each occurs. During this time forces are exerted on each object due to the compression of the objects. However, these forces are internal to the system and therefore should have no affect on the center of mass, i.e., the velocity of the COM remains constant.
We now look at what is happening during the collision using Newton's laws.
DURING THE COLLISION:
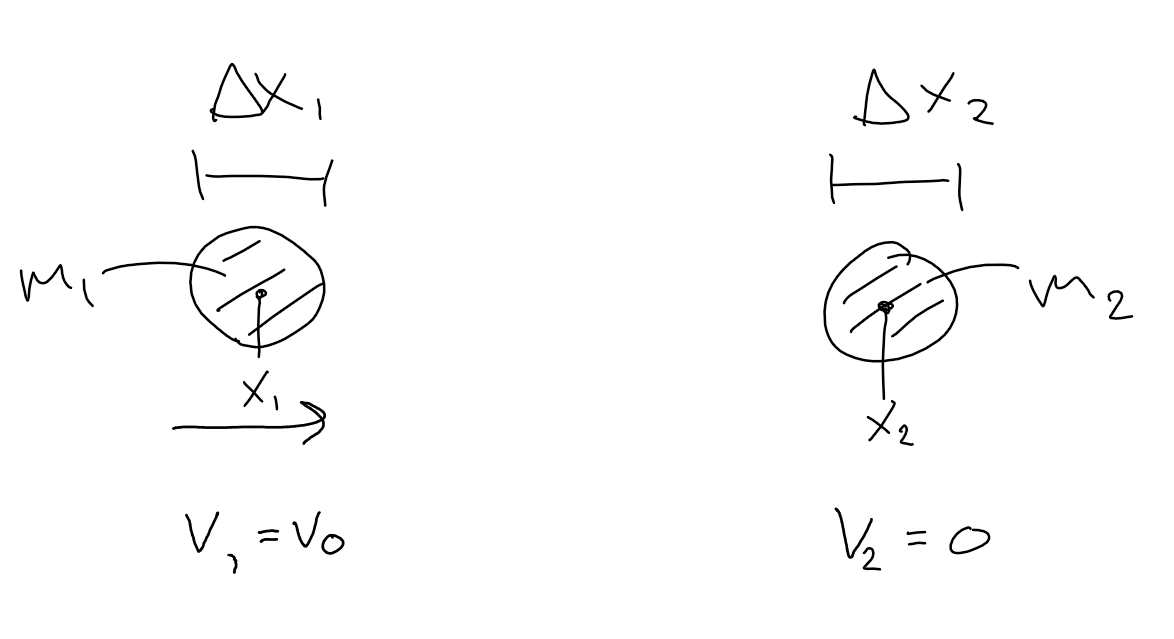
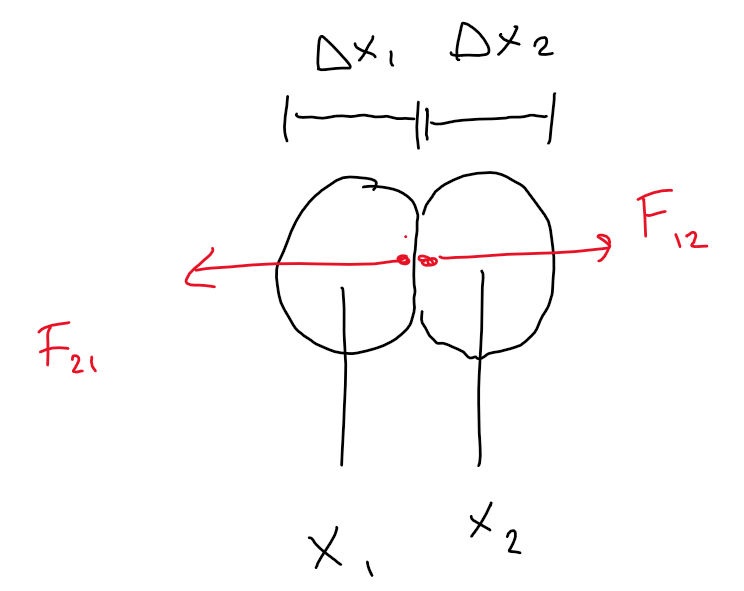
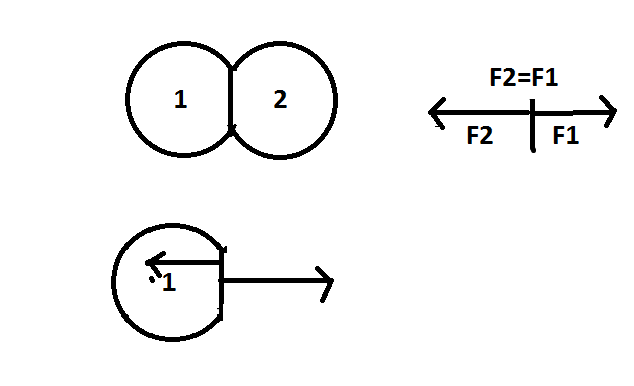
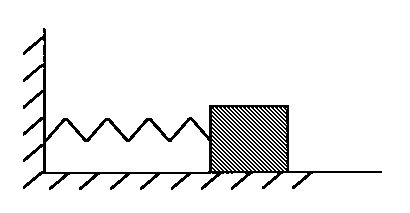
Modeling each object as a combination of an identical rigid body and ideal massless spring, where the spring represents the elasticity of each object, the collision of the springs results in a force acting upon each object. Per Newton's 3rd law
$$F_{1}=-F_{2}$$
Where
$F_1$ = the net force that Object 2's spring exerts on Object 1
$F_2$ = the net force that Object 1's spring exerts on Object 2
Since the masses of the two objects are identical, the accelerations are equal and opposite, or
$a_{1}=-a_{2}$
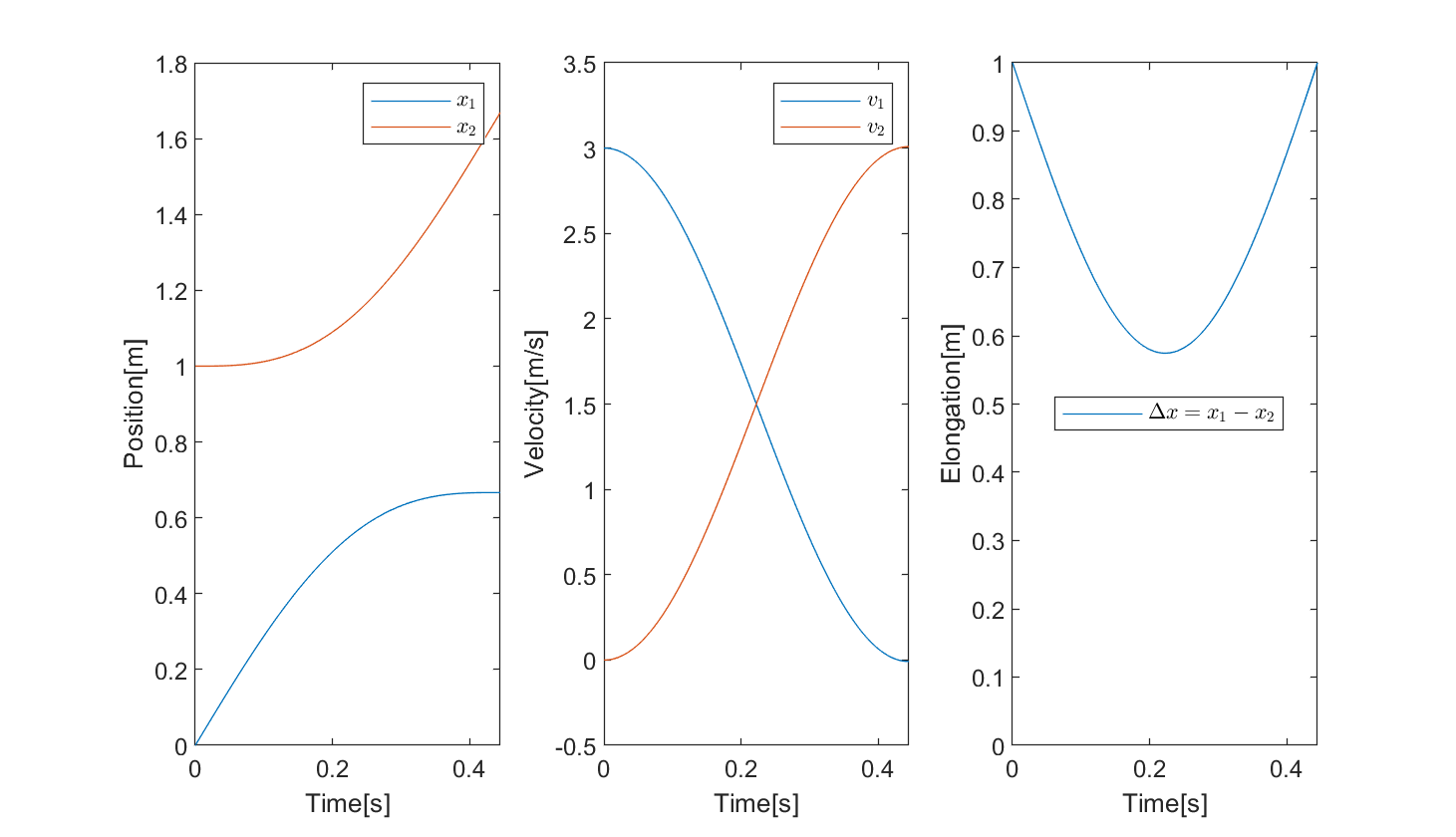
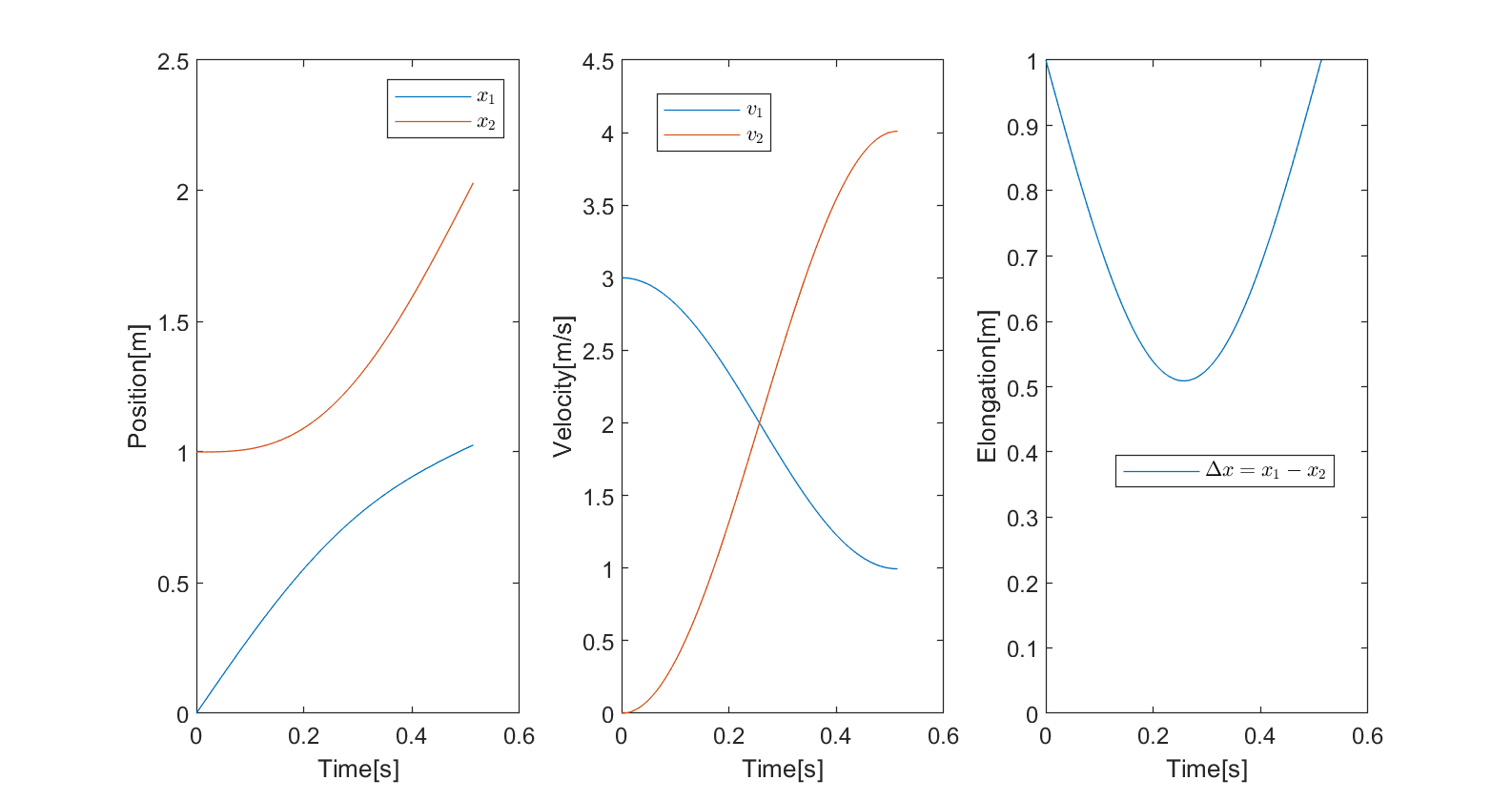
Since the duration of each acceleration is the same (the objects are in contact with one another for the same time), the change in velocity of Object 1 equals minus the change in velocity of Object 2,
$v^{'}_{1}-v_{1}=-(v^{'}_{2}-v_{2})$
Where the prime values are the velocities upon separation. Since Object 2 was initially stationary, $v_{2}=0$ and
(1) $v^{'}_{1}-v_{1}=-v^{'}_{2}$
We couple this with the velocity of the COM;
$$V_{COM}=\frac{v_1}{2}=\frac{v^{'}_{1}+v^{'}_2}{2}$$
(2) $v_{1}=v^{'}_{1}+v^{'}_2$
Solving equations (1) and (2) simultaneously gives
$$v^{'}_{2}=v_1$$
Since the final velocity of Object 2 equals the initial velocity of Object 1, and there is no change in total kinetic energy, the final velocity of Object 1 must be zero.
As a final comment, if Object 1 continued to move forward after the collision, this would indicate that the collision was inelastic. This can be shown in the simulation from the following link:
https://www.myphysicslab.com/springs/collide-spring-en.html
Although the simulation does not show both objects with a spring, it wouldn't matter on the behavior of the colliding objects since two identical springs in series is equivalent to one spring with a spring constant equal to one half that of each spring.
To see the effect of an inelastic collision, change the setting for the "spring damping" from zero, which is the default for an elastic collision, so say, 0.5 which damps the spring making it an inelastic collision. You will then observe that the first object will continue moving after the collision.
Hope this helps.