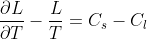0
$\begingroup$

Derive the equation.

enter image description here

Here L refers to latent heat, T for temperature, C_s for specific heat capacity of saturated vapour and C_l for specific heat capacity of liquid.

I tried using Clausius Clapeyron's equation but couldn't obtain the result. Please help.

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ An important realization in deriving the Clausius-Clapeyron equation is that $\Delta G=0$ during a phase change and that $G\equiv H-TS$ by definition; therefore, $L\equiv\Delta H = T\Delta S=T(S_s-S_l)$. Now (1) take the derivative with respect to temperature and (2) recall the definition of the heat capacity. $\endgroup$
    – Chemomechanics
    Commented Apr 5, 2021 at 6:31
  • $\begingroup$ Got it, thanks @Chemomechanics $\endgroup$
    – sheshin
    Commented Apr 6, 2021 at 3:04
  • $\begingroup$ @Chemomechanics thanks for the answer. Are L and H equal. They do not match dimensionally as L(latent heat) is energy per unit mass and H(enthalpy) is energy. Rectify me if I am getting the meanings or dimensions wrong. $\endgroup$
    – sheshin
    Commented Apr 6, 2021 at 3:19
  • 1
    $\begingroup$ Either everything should be normalized to unit mass or moles or it shouldn't. As you note, the units wouldn't match up correctly otherwise. (Normalized enthalpy and entropy are sometimes written as $h$ and $s$, respectively, for clarity, in which case we would write $L\equiv\Delta h=T\Delta s$.) $\endgroup$
    – Chemomechanics
    Commented Apr 6, 2021 at 3:28
  • $\begingroup$ Thanks @Chemomechanics $\endgroup$
    – sheshin
    Commented Apr 6, 2021 at 3:30

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.