1
$\begingroup$

IF we take small element in the circular loop then it must experience a magnetic force in a direction coming out or going into the plane of coil(depends on both the direction of magnetic field and the direction of current). if upper semicircular part experiences force coming out of the plane then lower semicircular part will experience force going into the plane and vice-versa. So this will definitely produce a torque (couple). If I suppose a small element making angle A with magnetic field then it experience force dF = iBdlsinA. i is current, B is magnetic field , dl is infinitesmal length of small element. Now upper semicircular part has many such small elements , each of them experiencing a varying force because angle A is also changing. The same applies to lower semicircular part. We know (arc/radius) = angle (subtended by small element at centre). This small angle subtended by dl is dA. So we can write dl = rdA(r is radius of circular loop). So dF = ibrsin(A)dA. Now on integrating this small force over angle A ,varying from 0 to (Pie), F = 2iBr. This is the force experienced by upper semicircular part, similarly same force i.e. 2iBr will be experienced by lower semicircular part and they together will produce a couple . Since this circular loop will rotate about diameter of circle ,and the distance between fulcrum and force is r(radius of circular loop) therefore net TORQUE = 2 * (2iBr * r) = 4iBr*r .But i know that it is wrong because by formula TORQUE= I *(Areaofloop)*B, It comes out to be i * pie * r * r * B. So please explain me what is wrong with my logic? 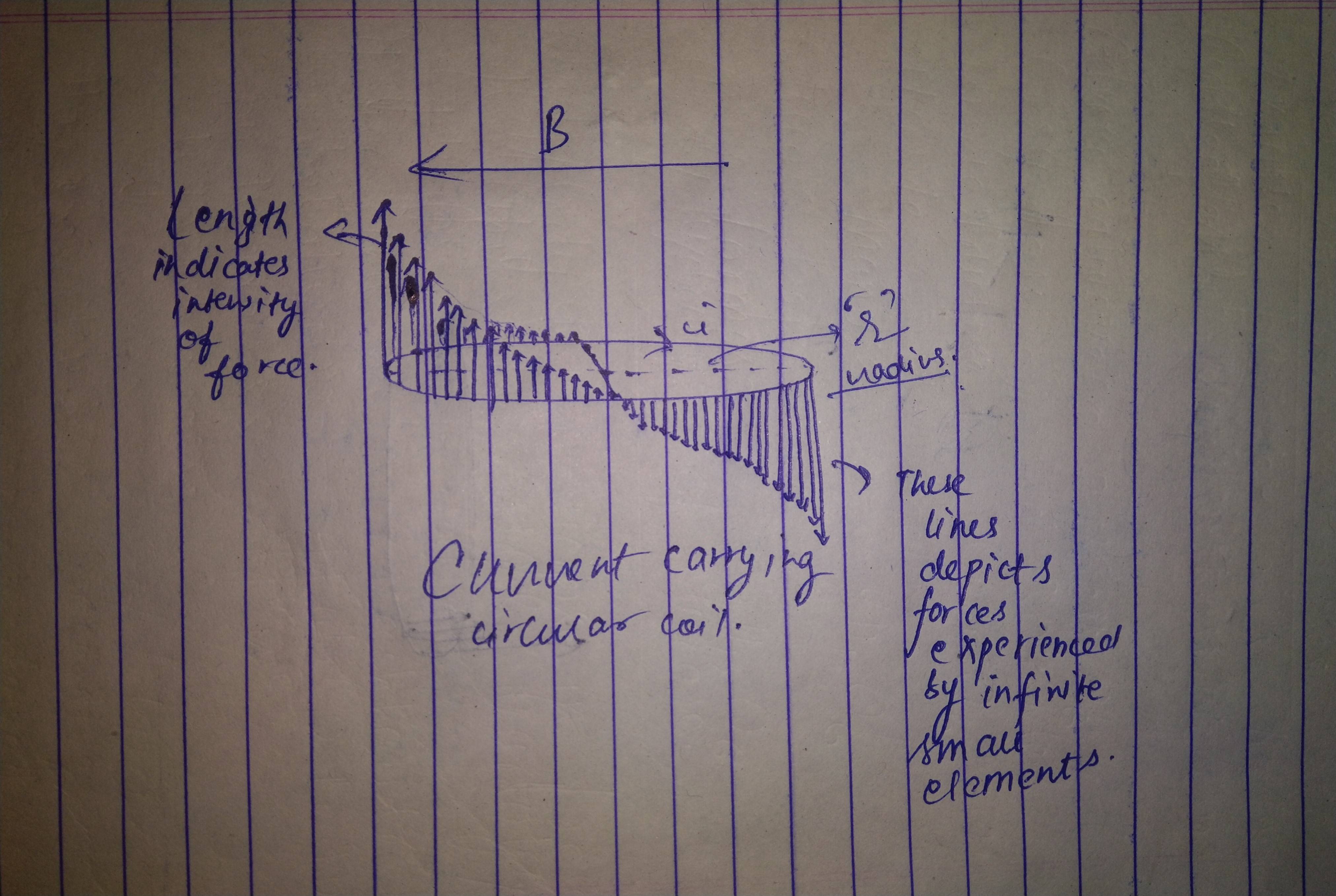enter image description here

As per my imagination I have attached a diagram above, those lines are forces experienced by small current element , the longer the line the stronger is the force. So finally they seem to produce a rotating couple with diameter of circle as its axle.Please tell me all wrong I have done. ThankYou.

Improve this question
$\endgroup$
1
  • $\begingroup$ You need to format the mathematics in your post using MathJaX. $\endgroup$
    – Triatticus
    Commented Apr 1, 2021 at 17:44

1 Answer 1

Reset to default
0
$\begingroup$

Each element, $r\,d\theta$, is not $r$ away from the central axis. As you move from the central axis not only does each element have a greater force exerted on it, $B\,i\,r\,d\theta\,\sin \theta$, the element is also a greater distance from the axis, $r\,\sin \theta$ where $\theta$ is the angle subtended between the central axis and the element.
Now find the contribution to the torque for each element and then integrate over the whole loop.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Farcher , I just want to know that is it possible that if I integrate small-small forces to find total force on upper semicircular and lower semicircular part and then find torque by multiplying by r(radius of circular loop ) then is it correct or not? What is the reason? $\endgroup$
    – Prajjwal XII A
    Commented Apr 2, 2021 at 19:10
  • $\begingroup$ No, $\vec{R}$ is not independant of the integral, meaning it cannot be brought out. $\endgroup$
    – jensen paull
    Commented Mar 27, 2022 at 19:16
  • $\begingroup$ Use polar coordinates instead of integrating the 2 cartesian equations that represent a circle $\endgroup$
    – jensen paull
    Commented Mar 27, 2022 at 19:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.