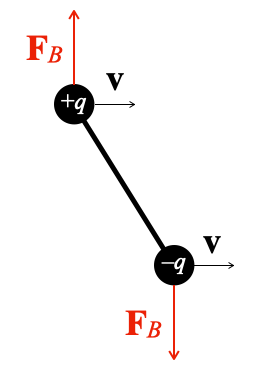
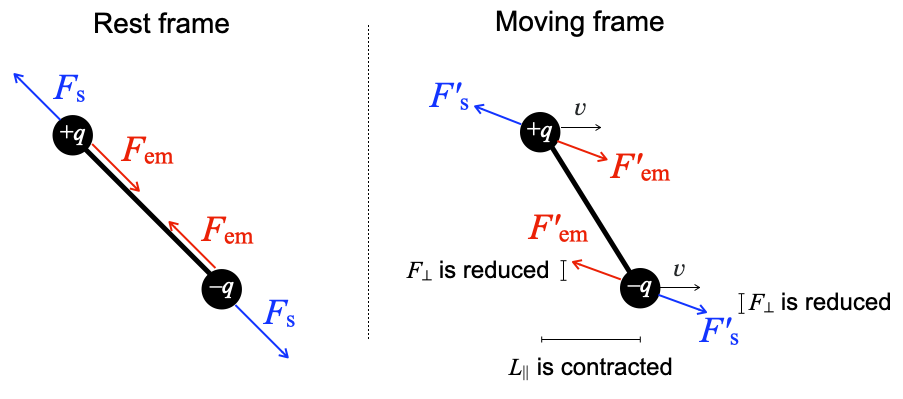
Ricky Tensor has beaten me to the punch, and his excellent answer provides the key insight: in the frame where the stick is moving, the force of the stick on the charges is not parallel to the stick itself. In my answer I'll just provide some intuition for why this should be the case.
Simpler setup, no angles
The necessity of this result can be seen in a much simpler setup. Consider a stretchable string connecting two equal masses, which has a constant tension $T$ in its rest frame. If the masses start off at rest, they will come together and collide due to the string.
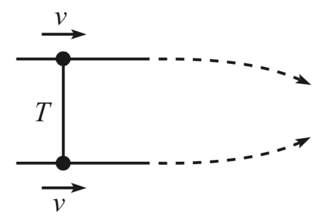
Now consider the reference frame where the masses are initially moving with velocity $v$ to the right. As the string accelerates the masses towards each other, the rightward velocity $v_x$ must stay the same, so the speed goes up, and the Lorentz factor $\gamma$ increases. But the rightward momentum is $p_x = \gamma m v_x$, which means $p_x$ must increase, which means there must be a force in the rightward direction. This force must be applied by the string itself, meaning that the force it applies is not parallel to itself.
(There is a second subtlety in this particular setup: if the string is exerting rightward forces on both particles, doesn't that violate conservation of momentum? No, because the string itself carries rightward momentum; it must carry an energy per length of $T$, which means it has a mass per length of $T/c^2$. As the string's length decreases, it gives up its momentum. But this is irrelevant to OP's question, since OP's stick has constant length.)
Defining shear stress
Here's a second way to see that this result is plausible. The reason the non-parallel force looks weird is because it suggests that there is a shear stress in the stick, while no such shear stress exists in the stick's rest frame. This would be a contradiction, since, e.g. we could replace OP's stick with a cylindrical fluid-filled balloon, which can't support shear stress at all.
The problem here is in the definition of shear stress. Demanding the force be along the stick is equivalent to demanding that the off-diagonal elements of the stress tensor vanish, $T_{ij} = 0$ if $i \neq j$. But this condition doesn't remain true under a Lorentz transformation, which mixes up diagonal and off-diagonal elements! The real definition of vanishing shear stress, from the solid's point of view, is that the off-diagonal elements are zero in the solid's rest frame. The constitutive relations of a solid only apply in the rest frame. In a general frame, all bets are off.
A microscopic model
The second explanation above might feel like a cop-out; it just says that there's no reason the stick's force has to be parallel to itself. But you might want a concrete, microscopic model that explains how the stick's force could be non-parallel to itself.
Of course, this would be rather difficult to do for a real solid, but here's a toy model. Let's replace the stick with a hollow column, within which is a single neutral particle. In the stick's rest frame, this particle elastically bounces back and forth, reflecting its velocity every time it hits one of the charges, leading to a time-averaged force along the stick.
Now let's consider the same situation in OP's reference frame. The particle still bounces back and forth, reversing its velocity every time it hits one of the charges. But because the stick itself is moving, the particle's velocity is not along the stick. It needs an additional rightward component to stay inside the stick as the stick moves to the right. This is the origin of the non-parallel force.
In principle, something similar is happening at the microscopic level in a real solid, though this would be very hard to show explicitly.