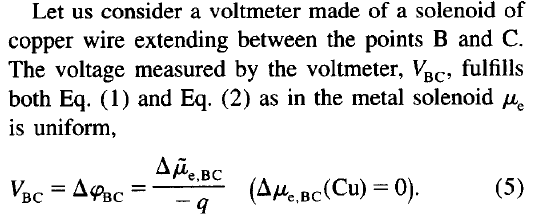2
$\begingroup$

I recently learned, that a voltmeter doesn't measure difference of electrical potential but difference of electrochemical potential $\tilde{\mu} = \mu/e + \Phi$. My original question was about measuring the voltage drop on an open p-n junction.

In the meantime I read the short paper of Riess "What does a voltmeter measure" and found that quite interesting. I feel free to show a small part of the paper here - hopefully it is not wrong to do this...

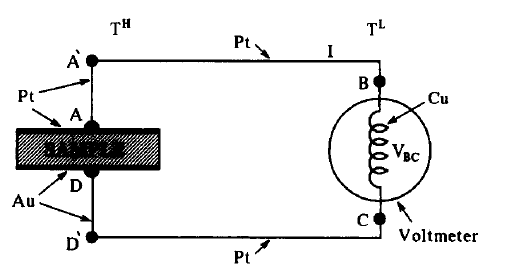
enter image description here enter image description here

It is argued, that in the measuring solenoid $\mu$ is uniform and I can understand this. However, a real voltmeter is more complicated and the measuring path includes non linear compounds of different materials, including semiconductors junctions as transistors, diodes and so on. I guess, instead of saying that $\mu$ is uniform, it is better to say, that $\mu$ is the same for both ports B and C, because the are usually of the same material. Right? But what if they are not of the same material, e.g. one is of Au and the other of Cu ? Then I have a contact voltage at the Au-Port, when I plug in a Cu-Strip. Assuming, the whole instrument is at the same temperature, again I will not "notify" this contact voltage, because it is again compensated by the other contact of the port, which is internally in the meter. I have a long chain of such contacts, but at some place the paths coming from the Port B and the Port C will meet at the same component. Therefore all contact voltages sum up to zero - is that right?

The original key question however is:

There is an electrical potential difference between the p and n far ends of a pn-junction. Can I physically say, there is a voltage between the two ends?? Or doesn't it make sense to attribute a voltage to that potential difference, because there is no net driving force behind?

If there is such a voltage, I'm not able to measure it, because whenever I attach probes of whatever kind, it will be compensated by contact voltages. So for me it gives more sense to say, that there is not a voltage between its ends. However this is in contradiction to electrodynamics, where we define $\Delta U=\int\Phi dx $ What is the right approach? At some point there is a (conceptual) problem...

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ Hi, it would be helpful to provide definition of every term in the equations, so there is no ambiguity. Now, a voltmeter most certainly can measure a voltage drop in situations where there is no electrochemical potential involved. It can only measure voltage across some pair of contact points, and only do so accuratly if the meter's internal impedance is at least 10 times the impedance of the item being measured. $\endgroup$
    – Carl Witthoft
    Commented Mar 23, 2021 at 12:43
  • $\begingroup$ $\tilde{\mu}$ is the electrochemical potential, while $\varphi$ is the electrical potential and $\mu$ the chemical potential. I think that sometimes $\tilde{\mu}$ is referred to as "fermi level". When the concept "voltmeter" ends at the connectors, I agree with your definition. However, when I measure something, I must place tips on two points and what I get is the voltage between those points. In the case of an open pn junction I will not measure any voltage, although there is a potential difference between its ends. If the junction is contacted by wires, this voltage is cancelled out. $\endgroup$
    – MichaelW
    Commented Mar 23, 2021 at 13:08
  • 2
    $\begingroup$ There is no potential difference between the ends of a diode. There is a barrier to moving charges across the depletion regions. The two are different concepts. The whole point of forming the depletion layer is to bring the two sides into equilibrium. $\endgroup$
    – Jon Custer
    Commented Mar 23, 2021 at 13:20
  • 1
    $\begingroup$ A pn junction is a diode. The field and total built-in potential difference in the depletion region is what is needed to bring the Fermi levels of the $n$ and $p$ regions into alignment. Once established, there is no potential difference across the diode. $\endgroup$
    – Jon Custer
    Commented Mar 23, 2021 at 14:06
  • 1
    $\begingroup$ The ends don’t have to be connected for the carriers to be in equilibrium - the junction already connects them. $\endgroup$
    – Jon Custer
    Commented Mar 24, 2021 at 15:41

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.