1
$\begingroup$

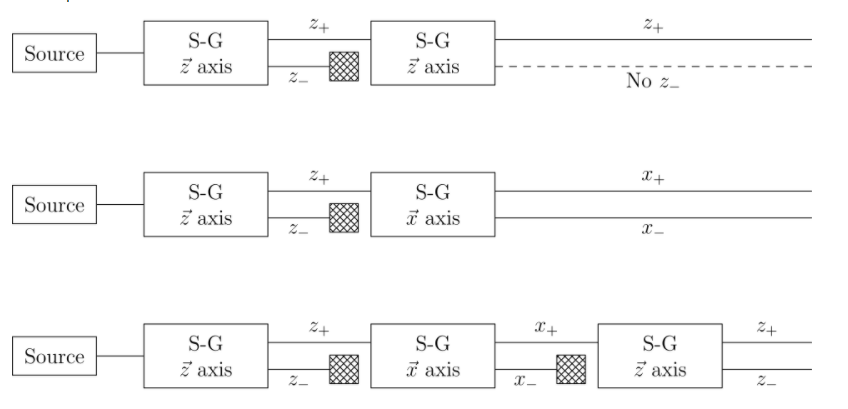
The Stern Gerlach experiment that established the quantization of spin in a particular direction, according to my understanding, does so while inevitably affecting the particle. To conduct a measurement on the spin, a particle has to be subjected to an inhomogeneous magnetic field in a particular direction.

enter image description here

Here SG z axis represents a set up that subjects the atoms to a magnetic field along the z axis, causing Larmor precession about the z axis such that z component of the spin remains unaffected. It is similarly done for the x direction, hence explaining the first two diagrams.

The third diagram is explained using quantum uncertainty and how the x and z components of the spin cannot be known simultaneously due to quantum measurements. However, my question is that could this not be explained using our understanding of physical laws itself? When particles are subjected to SG x after SG z, they would physically start Larmor precession about the x axis due to magnetic field along the x axis. This would lead to a loss of information in the z component of the spin. Hence isn't the process of measurement itself affecting the state, and explaining the third diagram, without the need to bring in Quantum uncertainty?

Improve this question
$\endgroup$
3
  • $\begingroup$ After you measure the spin along the $x$-axis, the spin points along the $x$-axis, so how would it precess about the $x$-axis? $\endgroup$
    – Sandejo
    Commented Mar 16, 2021 at 6:13
  • $\begingroup$ When we measure spin along x axis, we get information about the x component of the spin. The entire spin would not point along the x axis. The y and z components of the spin would cause Larmor precession if magnetic field is pointing in the x direction, (which is done to measure spin along x direction). $\endgroup$
    – UVcatastrophe
    Commented Mar 16, 2021 at 11:29
  • $\begingroup$ After you measure the sin along the $x$-axis, the state changes (or “collapses”) to an eigenstate of the $S_x$ operator. Therefore, the $y$ and $z$ components of the spin are no longer well defined. $\endgroup$
    – Sandejo
    Commented Mar 16, 2021 at 22:03

2 Answers 2

Reset to default
2
$\begingroup$

Your story is not completely correct. Let's remember about Larmor precession classically. It says that if you have a magnetic moment vector $\vec{\mu}$ in an external magnetic field $\vec{B}$, that $\vec{\mu}$ will rotate around $\vec{B}$ with a constant frequency. If a $\vec{\mu}$ is aligned or anti-aligned with $\vec{B}$, then there will be no precession. If $\vec{B}$ is constant in space then there is no net force on the particle.

If $\vec{B}$ is changing in space, then another effect kicks in. Namely, there is a net force of $$ \vec{F} = -\vec{\nabla} (\vec{\mu} \cdot \vec{B}). $$ In the Stern Gerlach apparatus, there is indeed a magnetic field which is changing in space. If the particle is spin up, and $\vec{\mu}$ is aligned with $\vec{B}$, then the force accelerated the a particle in the direction of decreasing $\vec{B}$. If the particle is spin down, it is accelerated the other way. This is how the beams are separated depending on if they are spin up or spin down.

Note that Larmor precession actually does not play a role here. If the spin is completely aligned or anti aligned with the field, it plays no role. Because no actual Larmor precession is occuring in this experiment, it is not fair to say it is the reason that the information about the original state is lost is that the Larmor precession somehow washes it out.

Edit: Quantum spin should not be thought of as a three dimensional vector, like $(v_x, v_y, v_z)$. Instead it is described by a two dimensional vector with complex components. In particular, $$ | \uparrow_x \rangle = \frac{1}{\sqrt{2}}\begin{pmatrix} 1 \\ 1\end{pmatrix} \hspace{1 cm}| \downarrow_x \rangle = \frac{1}{\sqrt{2}}\begin{pmatrix} 1 \\ -1\end{pmatrix} $$ $$ | \uparrow_z \rangle = \begin{pmatrix} 1 \\ 0\end{pmatrix} \hspace{1 cm}| \downarrow_z \rangle = \begin{pmatrix} 0 \\ 1\end{pmatrix}. $$ Note that, while in three dimensions the vector $(1, 0, 0)$ is orthogonal to $(0, 0, 1)$, when it comes to the quantum state we have $$ | \uparrow_x \rangle = \frac{1}{\sqrt{2}} | \uparrow_z \rangle + \frac{1}{\sqrt{2}} | \downarrow_z \rangle $$ so the up state in the $x$ direction is one half the up state in the $z$ direction and the down state in the $z$ direction. Thus if you measure the spin of $|\uparrow_x\rangle$ in the $z$ direction, one half of the time you find it spin up and the other half of the time you find it spin down.

Improve this answer
$\endgroup$
10
  • $\begingroup$ I understand your explanation for how we observe two beams, however, my question still remains. If I'm not wrong, it should never be possible for the spins to completely align or misalign with the magnetic field (say one that points in the z direction) as the direction of spin is quantized. If spin completely points in the angle of measurement (z), we would have the information about its x and y components too, them being zero. So the case that you describe should not be possible. $\endgroup$
    – UVcatastrophe
    Commented Mar 16, 2021 at 11:24
  • $\begingroup$ What you describe is really the crux of quantum spin. You can't think of it as a three dimensional vector $(v_x, v_y, v_z)$ like you might classically. Please see my recent edit to the answer $\endgroup$
    – user1379857
    Commented Mar 16, 2021 at 15:29
  • $\begingroup$ To be able to define $\mu$, don't we need to consider spin as a three dimensional vector in space? Since $\mu=g_s\frac{q}{2m}\vec S$. We need to consider the three dimensional magnetic dipole moment to describe Larmor precession using the equation $$\tau=\mu \times \vec B$$. So why is it that we can write the equation involving $\mu$ you wrote to describe deviation but not this one that describes precession? $\endgroup$
    – UVcatastrophe
    Commented Mar 17, 2021 at 15:39
  • $\begingroup$ There is a difference between the classical story and the quantum story. The classical story can be used as a useful mental model to explain some features of the Stern Gerlach experiment, but not all of them. For instance, there isn't really a well defined notion of "force" or "torque" in quantum mechanics, everything just evolves according the the Schordinger equation. In fact, the energy $U = - \vec{\mu} \cdot \vec{B}$ is not even a number, but rather a $2 \times 2$ matrix in QM! Here, $\vec{\mu} = - g \frac{q}{2m} \vec{L}$... $\endgroup$
    – user1379857
    Commented Mar 17, 2021 at 15:45
  • $\begingroup$ ... where $\vec L = \frac{\hbar}{2}( \sigma_x, \sigma_y, \sigma_z)$ and $\sigma_x, \sigma_y, \sigma_z$ are the $2 \times 2$ Pauli matrices. The average energy of the state $| \psi \rangle$ is $\langle \psi | U | \psi \rangle$. So the energy $U$ is a $2 \times 2$ matrix, but its expectation value is indeed a single number. Schroinger's equation says that the state $| \psi \rangle$ evolves in time according to $$ i \hbar \frac{d}{dt} | \psi \rangle = U | \psi \rangle.$$ Or anyway, this is how the spin degree of freedom works. If you want to describe the position there's more to it. $\endgroup$
    – user1379857
    Commented Mar 17, 2021 at 15:47
0
$\begingroup$

I revisited this question recently. After thinking about it again, I realized that it is the formation of discrete lines on the output screen that points to quantization of spin. According to the classical model I described in the question, the output would be a random distribution of atoms on the screen. Depending on the different phases in the precession of some classically imagined spin vectors, $\vec\mu.\vec B$ will take continuous values over whole spectrum as opposed to just two. Hence the appeal to quantum mechanics.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.