Stern-Gerlach experiment is often seen as idealization of measurement. Using strong magnetic field, it makes magnetic dipoles (of e.g. atoms) align in parallel or anti-parallel way. Additionally, gradient of magnetic field bends trajectories depending on this choice.
Magnetic dipoles in magnetic field undergo e.g. Larmor precession due to $\tau=\mu \times B$ torque, unless $\mu \times B =0$ what means parallel or anti-parallel alignment.
Precession means magnetic dipole becomes kind of antenna, should radiate this additional kinetic energy. Thanks to duality between electric and magnetic field, we can use formula for precessing electric dipole, e.g. from this article:
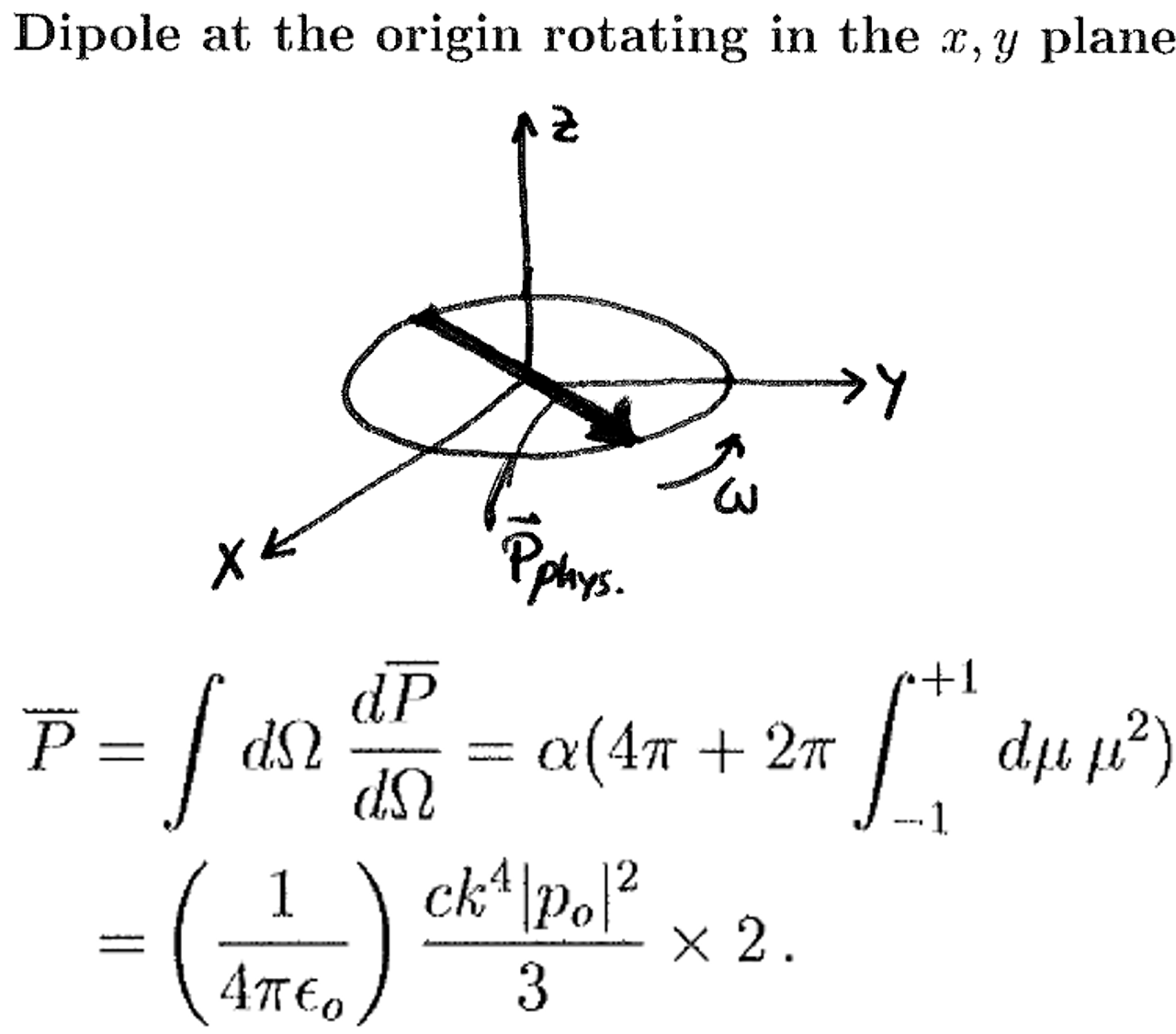
Using which I get power like $10^{-3} W$, suggesting radiation of atomic scale energies ($\sim 10^{-18}J$) in e.g. femtoseconds (to $\mu \times B=0$ parallel or anti-parallel).
So can we see spin alignment in Stern-Gerlach as a result of EM radiation of precessing magnetic dipole?
Beside photons, can we interpret other spin measurement experiments this way?
Update: Connor Behan below has found very nice article "Phenomenological theory of the Stern-Gerlach experimen" by Sergey A. Rashkovskiy with detailed calculations - getting $\sim 10^{-10}$s times for such alignment of atoms in Stern-Gerlach: https://www.preprints.org/manuscript/202210.0478/v1
Instead of energy radiation (are they equivalent?), it directly uses below formula ((3) in article) for dynamics of magnetic dipole $v$ of gyromagnetic ratio $\gamma_e=-e/m_e c$ in external magnetic field $H$, $b$ is semi-classical calculated parameter : $$ \dot v = \gamma_e\, v \times H - 2\alpha\, v \times \dot{v} +\frac{b}{c}\, v\times \ddot{v} $$
Update: Analysis of EM waves radiated by rotating dipole: http://web.hep.uiuc.edu/home/serrede/P436/Lecture_Notes/P436_Lect_13p75.pdf
