Emissivity and thermal equilibrium

Question

Lets say I enter a closed room with the walls and everything in it (including me and my eyes) at thermal equilibrium. Its a very hot room, but my super-eyes still work at 5000 degrees Kelvin.

I have two propositions which are in conflict.

The first is that I should be effectively blind when I am in the room. For me to see anything at all, the pigments in my retina would have to absorb some of the thermal radiation and convert it into electrical impulses for my brain. (The same applies for anything which detects radiation). But we can't extract energy from the heat without a cooler object, and there is none, so we can't use the energy in the black-body radiation for vision. There is no available free heat energy; everything is at thermal equilibrium. What is happening is that the pigments in my retina are generating black body radiation at the same rate as they are absorbing black body radiation from the object being looked at, so no net chemical change can occur and hence no vision.

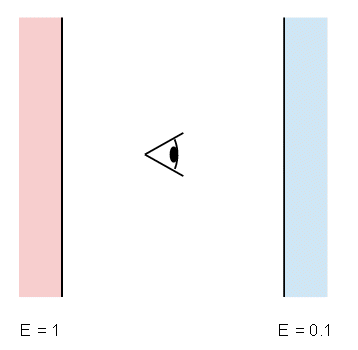
However, I also know that objects have different emissivity, which means some objects in the room will be brighter than others. If I am looking at an object of lower emissivity than the pigments in my eyes, my eyes should radiate more power out than they absorb from the object, and vice versa for an object of higher emissivity. This means that if I look at different things, the pigments in my eyes will react differently to different objects, meaning vision is possible. But this contradicts the first proposition.

Can somebody explain this "paradox" ?

Answer 1

To simplify the situation let's imagine one wall of the room has an emissivity of 1 and the other has an emissivity of 0.1.

The question you're asking is whether you'll be able to see that the wall with emissivity of 0.1 looks darker. If so this does seem to be a paradox.

The resolution is that the emissivity and reflectivity are related by $E + R = 1$. Assuming everything is in thermal equilibrium the room and everything in is bathed in light with a colour temperature of 5000K. When you look at the wall with emissivity of 1 you see only emitted light because the reflectivity is zero. When you look at the wall with emissivity of 0.1 you see emission at only 10% of the other wall, but the other 90% is made up of reflected light. So the answer is that both walls will look equally bright.

Answer 2

The question basically boils down to the idea that if two objects are in radiative thermal equilibrium with different emissivities, there will be a net flow of energy from one to the other. However, Kirchoff's law of thermal radiation states that this is not true: in radiative equilibrium, the absorptivity and emissivity of any body must be equal. This follows directly from the second law, which prohibits such a net flow of energy in thermal equilibrium.

In general, emissivity is not equal to absorptivity, so this might seem a little paradoxical. But the reason they can be different is that they're wavelength-dependent. The emissivity must equal the absorptivity at any given wavelength, but away from equilibrium the wavelengths of the emitted radiation can be different from those of the absorbed radiation.

For example, sunlight (which is approximately black body radiation at around 6000K) consists mostly of higher-frequency light than the IR emitted by bodies at everyday temperatures. Therefore if a body has a low absorptivity (and hence also a low emissivity) at short wavelengths and a high emissivity (and high absorptivity) and long wavelengths, then under everyday conditions we'll measure its emissivity to be higher than its absorptivity. But if you put it in a hot closed room and let it come to equilibrium, the frequencies it emits will be the same as the frequencies it absorbs, and so there will be no net flow. So you would indeed be blind (as well as dead) if you were in thermal equilibrium at 5000K.