How do we distinguish between systems and bodies when dealing with forces and moments?

Question

In my preperation for an exam I came across the following question:

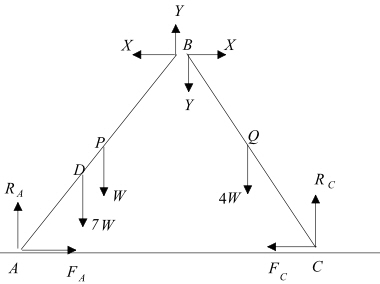
In this question I treated the hinged laders as one body and thereby resulted forces and took moments. However, this does not yield the correct answer, and moreover, doesn't tell me which ladder slips first. The markscheme presents the following diagram

My question is, when can we treat a system as a single object? Does this only work only in the context of resolving forces but not with moments? If anybody could assist me in intituively thinking about forces, moments and such systems I would gretly appriciate it.

Answer 1

In the context of statics, you can treat multiple objects as one if you are not interested in any internal details. For example when analyzing a bridge, all the parts of the bridge can be treated as one body if only the support forces are needed.

In the context of dynamics, then you cannot treat multiple objects as one, unless they are kinematically linked to move together. Bodies that have different degrees of freedom, cannot be clumped together.

This particular problem is a quasi-static problem (slow moving painter) but because the hinge between the ladders cannot transmit torque special care must be taken. The torque to balance the ladders comes from the ground friction and so if you treat it as single body, you must assume the pin can resist rotation, and convert the measured torque on the pin into an equivalent friction force.

It would be much simpler, to treat this with each body on their own (FBD diagrams), with each having their own balance equations. Two bodies, and three equations per body = 6 equations. Count your unknown forces and find they are 6 also. Two per contact, and 2 at the pin.

Answer 2

Here is my intuitive answer. The vectors denoted X in the image must be in balance because the hinge itself doesn't come apart. Since the outward pointing vectors are equal the "victor" is determined by their frictional potential. $μ$ is equal on both sides thus it all depends on the downwards force on $A$ and $C$ respectively. Because she is significantly closer to $A$ she will contribute the vast majority of her weight to that side and consequently $C$ will be the side that is slipping.