Waveguides (in the ocean?)

Question

The speed of sound in the ocean is given by

$$c_s(\theta,z) = 1450 + 4.6\theta - 0.055\theta^2 + 0.016z$$

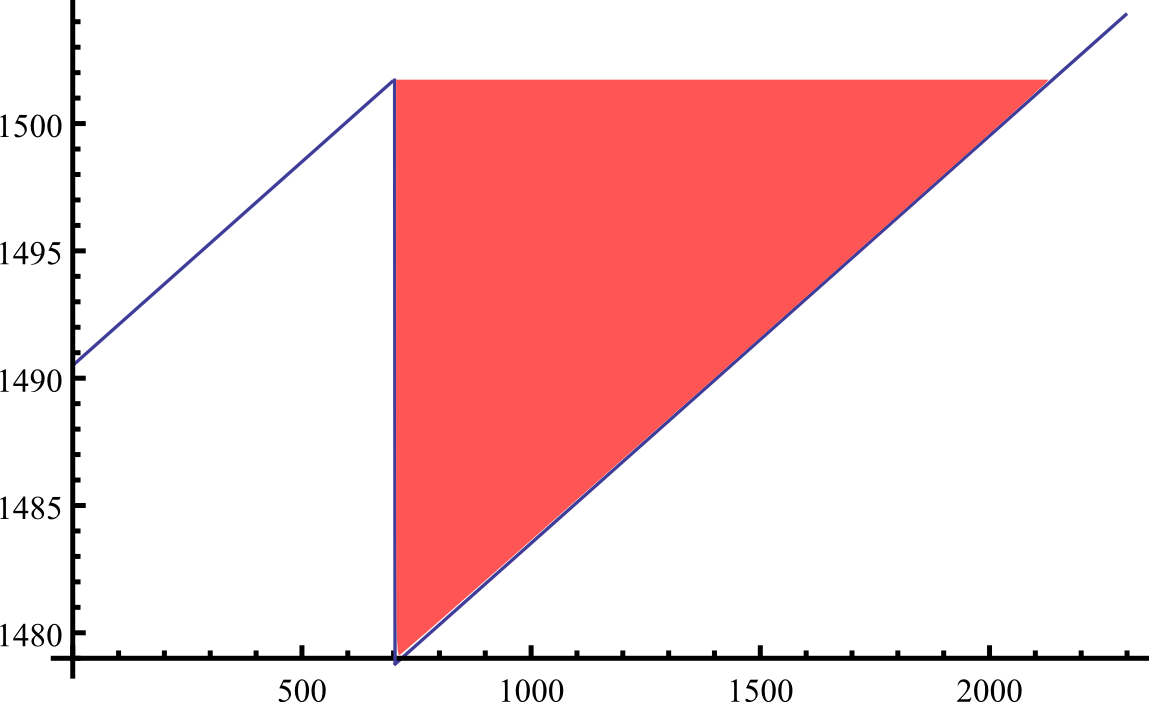
$\theta$ is the temperature in degrees celcius, and $z$ is the depth. In a simplified model, $\theta$ is constant at 10$\,^\circ $C for the part of the ocean above the "themocline". The thermocline is an interface at depth 700 m over which the temperature drops to 4$\,^\circ $C almost instantly.

The question: It claimed that the water below the thermocline can act as a waveguide. Why and what is the extent (in depth) of this waveguide?

My thoughts: Evaluating some relevant speeds: $c_s(10,700) = 1501.7 m\,s^{-1}$ and $c_s(4,700) = 1478.7 m\,s^{-1}$. As the speed changes at the thermocline, there will be refraction and reflection of incident waves from both sides (above and below).

So waves incident from below will be reflected back. However I don't understand what makes the waves reflect on the lower side of this "waveguide". As far as I can see, the speed of sound will increase with depth. If there is no interface with a sudden discontinuity like the thermocline, how does this situation work?

Answer 1

You don't need a sharp discontinuity in the speed of sound to guide the waves. Remember that reflection does not occur right at the interface; rather, the wave always penetrates outside the waveguide to "see" what's going on there. A gradual increase in the speed of sound enforces the wave to reflect as well.

Reflection occurs from above due to the thermocline (700 m), and from below due to the linear increase in the speed of sound, see the red shaded area in the following figure:

Guiding from below is possible down to a depth where the speed of sound equals $c_s(10,700)$ which yields 2136.25 m. The reason for this is explained in the following.

A wave which is guided has different properties than a freely propagating wave. In particular, one can show that a guided wave has a larger speed (please note that a guided wave propagates with one single speed throughout the waveguide, it is incorrect to imagine it as a particle bouncing off, e.g., two mirrors) than the minimum speed in the waveguide (in this case $c_s(4,700)$) yet smaller than the maximum speed above or below the waveguide, whichever is smallest (in this case $c_s(10,700)$). The reason for this is because a guided wave in a slab waveguide can be conceptually decomposed into two WAVES (not particles!) bouncing off the "walls" of the waveguide thereby preventing them from leaking out. This interference between the two waves creates a standing wave pattern inside the waveguide perpendicular to the propagation direction. Hence, a wave with a speed larger than $c_s(10,700)$ would immediately leak out of the waveguide and propagate to the upper infinity.

Answer 2

I wonder if you're mixing up the thermocline and the SOFAR channel. The speed of sound is a minimum at the SOFAR depth, so water at this depth acts as a waveguide.

I imagine sound will reflect off the thermocline, but I don't see how this would act as a waveguide unless the sea bottom acts as the lower reflector.

Answer 3

You don't need a sharp discontinuity in the speed of sound to guide the waves. Remember that reflection does not occur right at the interface; rather, the wave always penetrates outside the waveguide to "see" what's going on there. A gradual increase in the speed of sound enforces the wave to reflect as well.

Reflection occurs from above due to the thermocline (700 m), and from below due to the linear increase in the speed of sound, see the red shaded area in the following figure: Guiding from below is possible down to a depth where the speed of sound equals $c_s(10,700)$ which yields 2136.25 m.