Need help with the position of a particle in a velocity-versus-time graph [closed]

Question

I am having trouble with a velocity-versus-time graph. I recently took a Physics test that asked this question: The graph shows the velocity versus time for a particle moving along the $x$ axis. The $x$ position of the particle at $t$=0 seconds was 8 meters. What was the $x$ position of the particle at $t$=2 seconds What was the $x$ position of the particle at $t$=4 seconds?

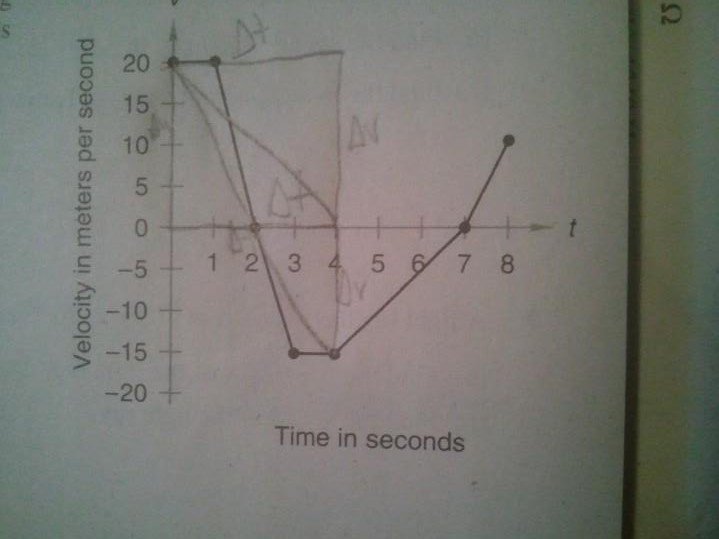
So, in order to solve this problem, I used the position equation: $x(t)=x_o + v_ot + \frac{1}{2}at^2$. Starting with the first position, I plugged 2 seconds into $t$, the time; 8 meters into $x_o$, the initial position; 20 meters per second into $v_o$, the initial velocity; and -10 meters per second squared into $a$, the acceleration. I derived the acceleration from the graph using $\frac{\Delta v}{\Delta t}$.

$a = \frac{\Delta v}{\Delta t} = \frac{-20}{2} = -10$

The position equation, with everything in place, reads:

$x(2)=8 + 20(2) + \frac{1}{2}(-10)(2^2)$

This math works out to 28 meters, which is the answer I gave on the test. I did the same thing for the second part of the question--the position of the particle at 4 seconds, as well as the acceleration based on the change in velocity and the change in time.

$a = \frac{\Delta v}{\Delta t} = \frac{-35}{4} = -8.75$

The equation comes out to this:

$x(4)=8+20(4)+\frac{1}{2}(-8.75)(4^2)$

The math comes out to 18 meters. However, according to the curriculum I used, the answer to the first part is 38 meters and the answer to the second part is 16 meters. How can this be the case? Did I fail to apply the position equation correctly? Did I make a mistake when computing the acceleration of the particle? I can't figure out what I did wrong. Is it possible that I used the wrong equation?

I really have no idea. I would appreciate any insight you might have to offer. Thank you in advance for you consideration.

Answer 1

I'm not sure why you are using the displacement-time formula if you have the shape of the graph.

The distance covered by the particle is given by the area under the graph from point 0.

After t = 2s, the distance the particle would have covered is the area under the trapezium, that is: $$\dfrac12 (1+2)(20) = 30$$

The position of the particle becomes $8 m + 30m = 38m$.

Maybe you can work out the second part now.

Explanation:

Your formula assumes constant acceleration, which is not the case! For the first 1 s, the acceleration is $0ms^{-2}$, the next 1s, the acceleration is $-20 ms^{-2}$! If you used your formula for $0<t<1$ and then $1<t<2$, then you would have obtained the desired answer. But it's just making the problem more complicated than it actually is at that point :)