Let $\phi$ be a real scalar field and
$$\mathcal L = \frac{1}{2}(\partial_\mu \phi)^2 + \frac{1}{2}m^2\phi^2 - \frac{\lambda}{4!}\phi^4.$$
Given the standard Feynman diagrams and Feynman rules for $\phi^4$ -theory, let us consider the one particle irreducible (1PI) 4-point correlation function up to $O(\lambda^2)$, that is
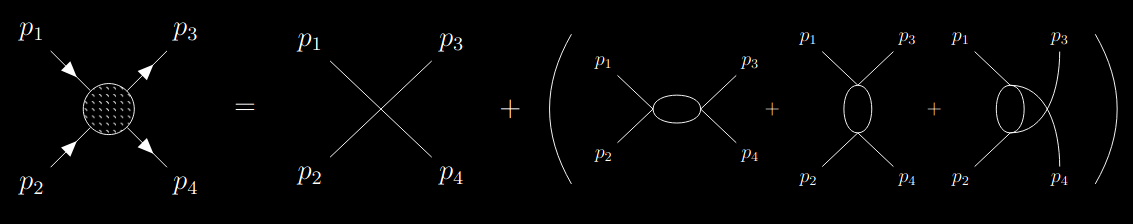 In my lecture notes it is written that the complete correlation function is then given by ($s,t,u$ are Mandelstamm variables)
$$i \mathcal{M}_{12 \rightarrow 34}(s, t, u)=-i \lambda+(-i \lambda)^{2}[i V(s)+i V(t)+i V(u)],$$
where
$$iV(p^2)= \frac{1}{2}\int \frac{d^{4} k}{(2 \pi)^{4}} \frac{i}{k^{2}-m^{2}+i \epsilon} \frac{i}{(k+p)^{2}-m^{2}+i \epsilon}.$$
I'm confused by this expression because of another set of lecture notes (same uni, the previous year) that state the amplitude of the first diagram in the parenthesis is
$$\begin{aligned}
F=& (-i \lambda)^{2}(2 \pi)^{4} \delta^{4}\left(p_{1}+p_{2}+p_{3}+p_{4}\right) \prod_{j=1}^{4} \frac{i}{p_{j}+m^{2}-i \epsilon} iV(s),
\end{aligned}$$
i.e. they included the momentum conservation and propagators.
In my lecture notes it is written that the complete correlation function is then given by ($s,t,u$ are Mandelstamm variables)
$$i \mathcal{M}_{12 \rightarrow 34}(s, t, u)=-i \lambda+(-i \lambda)^{2}[i V(s)+i V(t)+i V(u)],$$
where
$$iV(p^2)= \frac{1}{2}\int \frac{d^{4} k}{(2 \pi)^{4}} \frac{i}{k^{2}-m^{2}+i \epsilon} \frac{i}{(k+p)^{2}-m^{2}+i \epsilon}.$$
I'm confused by this expression because of another set of lecture notes (same uni, the previous year) that state the amplitude of the first diagram in the parenthesis is
$$\begin{aligned}
F=& (-i \lambda)^{2}(2 \pi)^{4} \delta^{4}\left(p_{1}+p_{2}+p_{3}+p_{4}\right) \prod_{j=1}^{4} \frac{i}{p_{j}+m^{2}-i \epsilon} iV(s),
\end{aligned}$$
i.e. they included the momentum conservation and propagators.
I'm struggling to get the terminology right here. $F$ and $\mathcal{M}_{12 \rightarrow 34}$ (I think at least) are essentially the same thing, but what do people usually call "amplitudes"? Do we include momentum conservation and propagators when we write amplitudes down or is it assumed that everybody can fill the gap?
