Interfering alternatives and identical particles in Feynman and Hibbs

Question

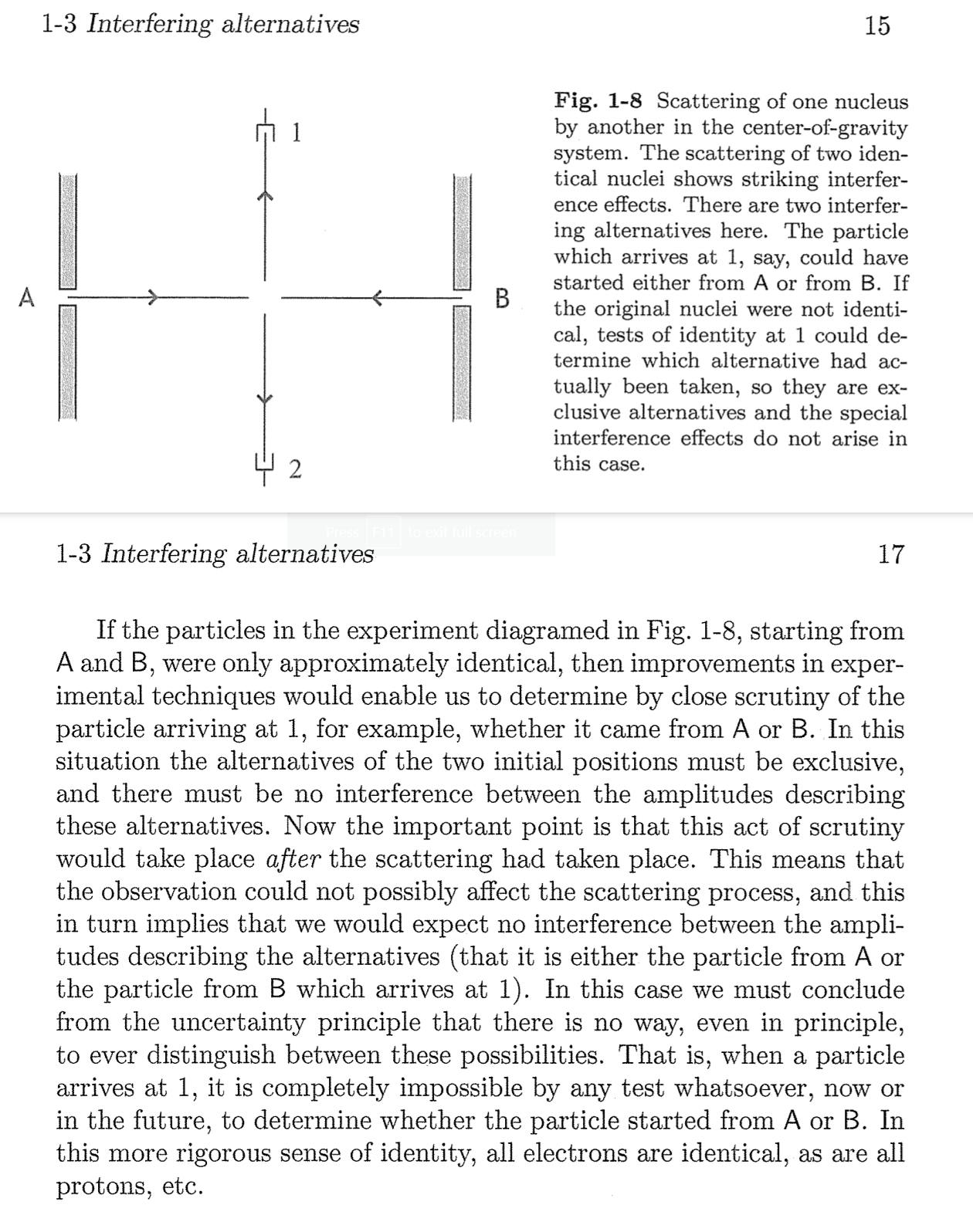
I am currently self-studying Feynman and Hibbs, and in his first chapter, Feynman talked about 'alternatives' like the various possibilities or paths an experiment can take. He defined two different types of 'alternatives'- interfering alternatives and exclusive alternatives. He then used this concept to explain Identical Particles in Quantum Mechanics (page 17), but I can't seem to understand his explanation for identical electrons using scattering experiments. I have linked the screenshot at the end of the page.

In summary, I'm confused with the following. Feynman argues that if two particles are "approximately identical", we can do a scattering experiment, starting from positions A and B, and then determine by close scrutiny if the particle reaching the position 1 was from A or B. Since this act of scrutiny occurs after the scattering event, our measurement can't affect the scattering process. If we can possibly resolve the difference between the two particles, and if the measurement to determine the particle doesn't affect the scattering process, that means that these alternatives are exclusive (particle from A reaches 1, and particle from B reaches 1) and there should be no interference between the probability amplitudes of these alternatives. I understand his arguments till now, but I start to get confused after this.

Now he says, that we can conclude from the uncertainty relation that there is no way to distinguish these possibilities. (particle from A reaches 1, and particle from B reaches 1), which sounds like a contradiction since we just discussed that they can be distinguished. And thus he concludes that identical electrons can't be distinguished.

I don't really understand the leap that he took from the inference of non-interfering amplitudes, to uncertainty relation and then to identity of electrons, neither was I able to find any other explanation on the internet about this. If someone could clarify it, that would make it a whole lot easier.

Answer 1

Even if you knew the energy the measurement gave the electron you cannot predict direction of electron not to mention you cannot even say anything about the photon you used to carry that measurement so we cannot measure before the particle reaches 1 or 2. As of present or in the future, However even without uncertainty principle the electron can be two places at the same time there is probability of it exchanging places if u repeat this experiment Even if you somehow(ideally) take a measurement at the last second before electron arrives at 1 it could simply there will still be some probablilty for it to arrive at because the electron wavefunction is also near 2. I can only say at best "I did this measurment and the electron will arrive likely at 1 after 1 second. " And after that one second I can at best say "This is most likely the elecron that started from A" (I can never know with certainty thanks to electron probability distribution.) Actually he does this prove the point that even if I somehow knew the trajectory it can be anywhere once once interference occurs and even if it doesn't occur probability distribution still applies. And that is why they are indistinguishable. Hope this helps.