Clarification in definitions of physical quantities

Question

What does "per unit" mean?

For example is defined as mass per unit volume. Mathematically we can write this as:$$ρ= \frac{dm}{dV}$$ The same goes for velocity and power. I can't understand what per unit volume or per unit time means. When we say that $Z$ is defined as $X$ per unit $Y$ (e.g. time) does it mean that we should take $X$ and divided by $Y$ or by the unit of $Y$. Also when stating such a definition should we include also the phrase $X$ "in unit of $Y$" per unit of $Y$?

When are differentials used for definitions?

As stated above we can define density with differentials of mass and volume. Does this mean that every physical quantity that is expressed as a ratio should be following the "differential" definition? For example could we define resistance as: $$R=\frac{dI}{dV}$$

How do physicists decide when to give a "differential" definition $\frac{dX}{dY}$ versus a definition like $\frac{X}{Y}$?

Answer 1

At first I want to say that your formula is incorrect. Density is mass per unit volume $\rho = m/V$, and not mass change (differential or delta) per unit volume. Your formula as it is stated shows that environment can be in homogenous, where density can change in some direction. Suppose you measure $dm/dV$ of air going upwards into higher atmosphere altitudes. You will get different values depending on altitude. Thus $\rho = \rho (h) $. However if you divide total mass of atmosphere by total it's volume, you will get an average density of atmosphere. So mass change over elementary volume lets us to evaluate local density or density dynamics, while total values ratio - average quantities.

As about what "per unit" means. Density is mass per unit volume. It means that if you take a $1~ m^3$ of steel and weight it's mass - what you will get is steel density, aka. "how much unit volume of something weights". Or you can take any volume of steel and divide by it it's total mass and you will get the same density thing. That's why density has meaning of mass per unit volume.

As about when to use differentials/deltas over absolute quantities - not the physicists decides but rather a nature law itself. Differentials are useful when law talks about relationship between changes in quantities. For example body acceleration is defined as it's speed change over time period, thus the formula is $a=\frac {dv} {dt} $ Hope that helps.

Answer 2

Descriptions such as "per unit time" are a way of generalising the units to broadly apply to any given physical situation, whether you are dealing with seconds, or perhaps a different unit of time.

In many situations in physics, particularly in theoretical or computational physics, idealised environments are considered where variables are dimensionless, or perhaps environments are considered where units are totally different, such as in particle physics where natural units are used that consider everything in terms of energy, the speed of light and other fundamental constants. Physics can even consider environments where fundamental physical constants are different and so the relations between what is a "second" or a "metre" with other units can completely break down.

By being general about the units used, the physics stays generalised and holds in various more abstract scenarios. This is often good practice.

As for differentials, a simple answer is that a differential will represent a scenario where the change in two variables is more important than their absolute values. For example, when considering velocity, distance and time, the velocity may be constantly changing (think of a polynomial plot of distance against time, with velocity as the gradient) and so simply dividing total distance by total time will not necessarily give the correct value for the velocity. Instead the current velocity must be found by finding the exact gradient at a given point. This can be thought of as the instantaneous change in distance over the instantaneous change in time, which analytically corresponds to a differential of a given (perhaps polynomial) equation relating distance and time.

Answer 3

When a quantity is function of another, it is meaningful to define a derivative. If $x = x(t)$ then we can talk of $v(t) = \frac{dx}{dt}$.

It is not the case of $\rho = \frac{m}{V}$. Here $m$ is not a function of $V$, and $\rho = \frac{dm}{dV}$ doesn't make sense. $\rho = \rho(x,y,z)$. It is the limit of the amount of mass contained in a small volume around a point, when this volume tends to zero.

Answer 4

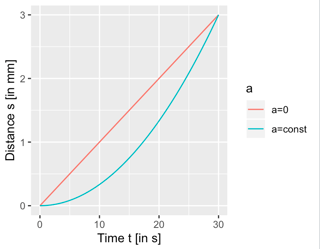
Let's consider an example: Velocity is displacement per unit time, $v = ds/dt$. If the word "unit" bothers you, you can simply omit it. So, if I measure a displacement of $\Delta s=3mm$ in the time interval $\Delta t = 0.5min$ the average velocity is $\bar v = 3mm/(0.5min)$, which is equal to $3mm/30s$ or $10^{-4}m/s$. However, we do not know the velocity at time $t=30s$, because we did not specify whether the acceleration was zero or non-zero. This is shown in the following plot where both motions travel the distance fo $3mm$ in the time interval $30s$. Hence, bot motion have the same average velocity (in this particular time interval), but the final velocity at time $t=30s$ differs.

Why do we use the phrase "per unit xyz"? In my opinion this phrase emphasises that it does not matter whether we wish to use seconds, minutes or weeks as our reference time interval. What ever we choose the corresponding quantity will be a velocity. Although the same is true for the nominator, we could choose to measure the distance in units of meters, foot, or light years, I have never read "velocity is a unit of displacement per unit time". In my opinion, stuffing unnecessary words into sentences does not help to improve the readability.

The second question is about the derivatives. As you have probably learned in math the first derivative of a curve is its slope (=tangent). The slope indicates a rate of change. Hence, if we are interested in this rate of change of a continuous quantity, we will define the quantity by the derivative. However, note that above I discussed the average velocity and used $\bar v = \Delta s / \Delta t$. It would be wrong to replace the ratio by the derivative. Hence, the definition is chosen according to our needs/interest. However, luckily, we are interested most often in the velocity at a given time, $$v(t_0) = \frac{ds}{dt}\big|_{t=t_0} =\frac{ds(t_0)}{dt} $$