0
$\begingroup$

I'm working my way through the paper Observable quantum entanglement due to gravity

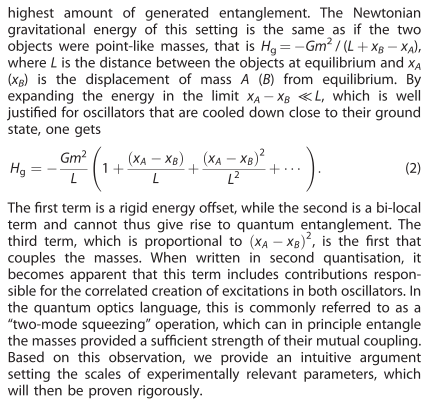
Equation 2 is a Taylor expansion of Newtonian gravity potential. They say the second term is bi-local and so cannot lead to quantum entanglement, and the third term couples the two test masses/leads to entanglement between them. Here's a snapshot of the equation, where $L$ is the separation between test masses and XA and XB their displacements from equilibrium:

equation 2

  1. Can you please tell me what bi-local means here? Maybe more importantly why can it not cause entanglement? Because it does not include a product of the form XA * XB?

  2. The reason the 3rd term entangles the two masses is because when written out, it includes a XA * XB term. Correct?

Any further insight into why product terms are associated with entanglement between the masses will be much appreciated.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ Please put a more conventional link to the (ideally) abstract page of the paper. As it stands it's some sort of chrome extension link which won't work for most people (if it works for anyone). Also note we actively discourage images of text or math and these should be typed in using text and Mathjax. $\endgroup$
    – StephenG - Help Ukraine
    Commented Dec 5, 2020 at 3:52
  • 1
    $\begingroup$ Okay thanks. I corrected the link. $\endgroup$
    – Bashir
    Commented Dec 5, 2020 at 3:58

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.