0
$\begingroup$

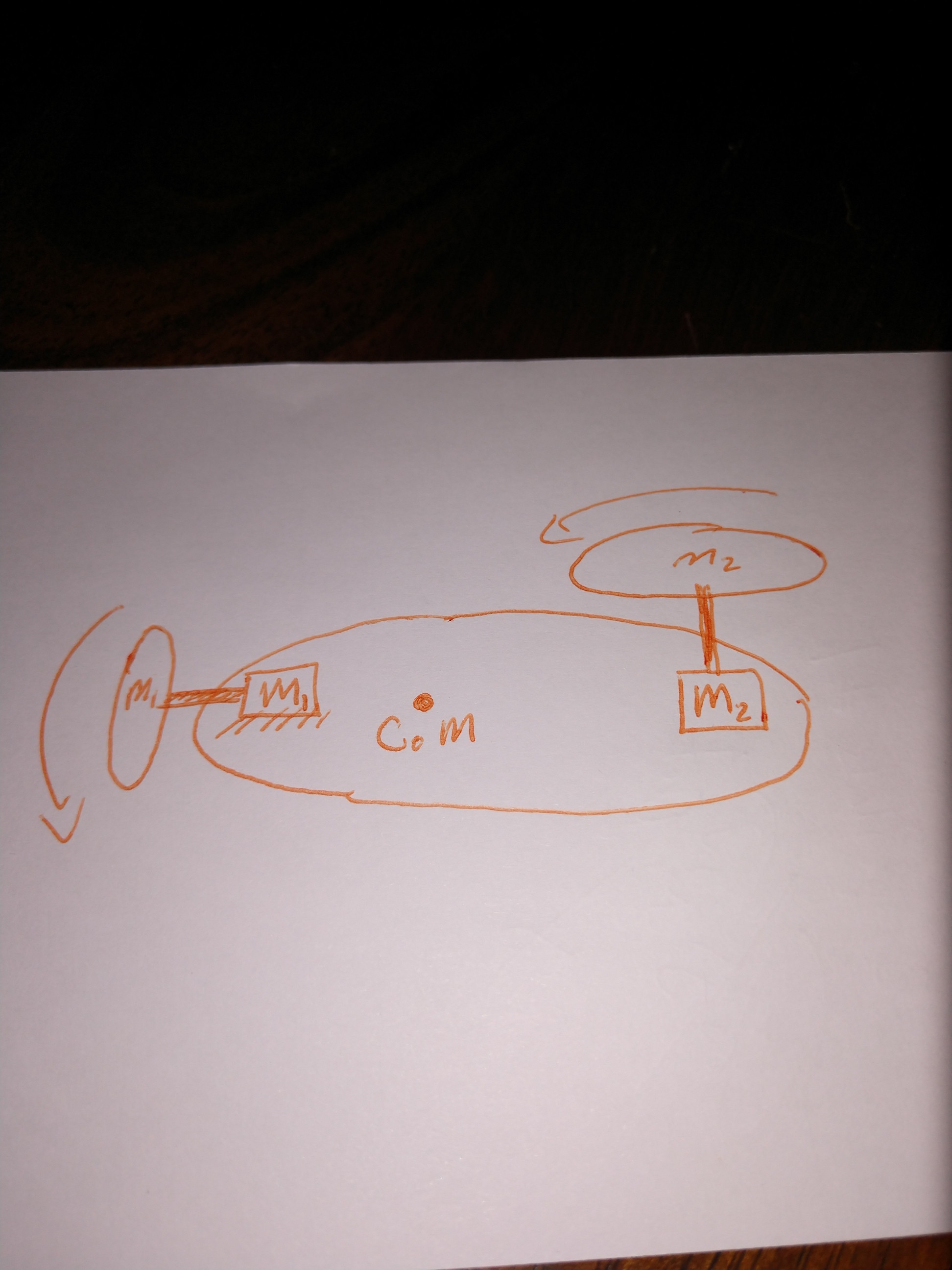
Imagine a turn-table in space, therefore there are no external forces on the system.

This turntable has two motors, turning various masses at different radius. Imagine one motor is at the edge of the turntable, spinning parallel to the turntable. Other motor is the opposite side of the turntable but spinning in a vertical/perpendicular direction.

Ang Momentum has to be conserved, but what axis would you use? And how would you calculate it? Would the overall system rotate about an axis that is a blend of both rotations?

I dont specifically need a math answer. Just conceptually trying to understand how you would even work a problem like this out, and how would the overall system would look and/or rotate in space given rotation about 2 different axis.

I get ang. momentum in our physics books, but it always about 1 axis, and very simplified systems. Examples are like a bullet and door, or two rotating disks falling ontop of a common axis. I know that ang. momentum doesn't apply where external forces exist, so many times it doesn't apply. But this has my brain wondering. Thank you

Here is a picture. Image a disk, with two motors, spinning objects. How is Ang. Momentum conserved? Is it still conserved about each motor axis as well as the full system? enter image description here

Improve this question
$\endgroup$
11
  • $\begingroup$ parallel axis theorem en.wikipedia.org/wiki/Parallel_axis_theorem which uses the concept of center of mass of the system $\endgroup$
    – Daddy Kropotkin
    Commented Nov 28, 2020 at 16:07
  • $\begingroup$ So for that system, are you saying you wouldn't calculate the angular momentum about the individual axis but rather center of mass and find the equivalent rotations and via the parallel axis therom? That way Even though the system rotates about the center of mass, therefore would still be conservation of Ang. Mom? $\endgroup$
    – Kevin C Speltz
    Commented Nov 28, 2020 at 17:06
  • $\begingroup$ School 15 years ago did teach an example with parallel axis therom, but only a system with even masses, nothing complicated like this. I wish they would do more examples. Been looking for one $\endgroup$
    – Kevin C Speltz
    Commented Nov 28, 2020 at 17:08
  • $\begingroup$ I don't really understand how your system is setup. Your description is unclear... maybe make a picture to specify the locations and relative orientations of things. Otherwise I can't give more info. If the axes of rotation in your system are parallel, then use the parallel axis theorem. If not, then it's more complicated and you'd need to set up a lagrangian. $\endgroup$
    – Daddy Kropotkin
    Commented Nov 28, 2020 at 17:59
  • $\begingroup$ I added a picture and a description of what I imagine. From what your saying, I'm guessing and assuming Ang momentum is still conserved, but gets much more complex. In general do we not worry about Ang momentum in most systems(like a crane or other engineering porblesms) becuase they are tied to the earth and therefore there is an external force, so it isn't conserved? Plus any changes to earth are so negligible/ canceled out so we can ignore it. I do engineering and most time we never consider Ang momentum of our systems. Thks $\endgroup$
    – Kevin C Speltz
    Commented Nov 28, 2020 at 20:05

1 Answer 1

Reset to default
2
$\begingroup$

Angular momentum has to be conserved along all possible axis of rotation. You can show that if it is conserved along three linearly independent axes, then it is conserved for all axis. So it is common to use a cartesian coordinate system and formulate angular momentum as a vector with components along all the directions of the coordinate system.

The most complex part of this process, is formulating the 3×3 mass moment of inertia tensor $\mathbf{I}$ for each separate body to be used such that

$$ \boldsymbol{L}_{\rm total} = (\mathbf{I}_1 \boldsymbol{\omega}_1 + \boldsymbol{r}_1 \times \boldsymbol{p}_1) + (\mathbf{I}_2 \boldsymbol{\omega}_2+ \boldsymbol{r}_2 \times \boldsymbol{p}_2) + \ldots $$

where $ \boldsymbol{L}_{\rm total}$ is a vector, as well as each rotational velocity $\boldsymbol{\omega}_i$. Also the location of each center of mass $\boldsymbol{r}_i$ and the momentum vector $\boldsymbol{p}_i$ has to be considered.

Improve this answer
$\endgroup$
2
  • $\begingroup$ One question I have is that over the years I have solved many engineering problems and essentially ignored Ang. Momentum. For example sizing hydraulics or motor torques to get winch performance ratings for a crane. Why is it when designing say the winch, we don't have to be concerned with how/where Ang. Momentum is concerned? Is it becuase in that case there are external forces such that Ang. Momentum isnt conserved in our system( winch itself). Plus, if the winch is connected to the crane and then earth, any gains in those are so negligible due to size we can safely ignore? $\endgroup$
    – Kevin C Speltz
    Commented Nov 28, 2020 at 23:34
  • $\begingroup$ Angular momentum is not conserved for things attached to the earth, but the time derivative of angular momentum is torque, which is crucial for calculating motor requirements. But you are right, momentum isn't that useful on its own unless there are impacts involved. $\endgroup$
    – John Alexiou
    Commented Nov 29, 2020 at 2:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.