Suppose that 2 observers are sending light signals to each other.If each of them are stationary in relation to a nearby galaxy, but each of these are separated by so great distance, they will be moving apart at speed which is higher than light: 
How's the type of violation of causality seen in the Krasnikov tube avoided?
Causality is violated if spacetime isn't time-orientable (you can't consistently identify one half of the light cone as the future half) or if it's time-orientable but has closed causal loops (spacetime paths that are future-directed everywhere but end up where they started).
FLRW cosmologies manifestly don't violate causality, because at every point, one half of the light cone points toward increasing cosmological time, and you can consistently take that to be the future direction; and then any spacetime path that is always future-directed is also monotonic in cosmological time, so it can't return to its starting point, which is at an earlier cosmological time.
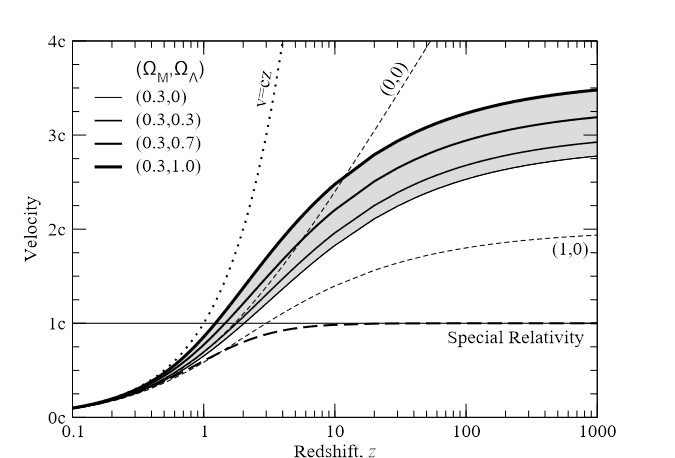
The greater-than-$c$ velocities that show up in cosmology have little practical meaning. As one example, in the graph in the question there is a curve labeled "$(0,0)$" which increases without bound, and another one labeled "Special Relativity" which tops out at $c$. Those curves actually describe the same cosmological model: the special case of FLRW cosmology in which the matter density is zero and so the spacetime is just Minkowski space. The reason the curves are different is that they're using two different definitions of velocity. The "Special Relativity" velocity is $dx/dt$ where $x$ and $t$ are Minkowski inertial coordinates, while the "$(0,0)$" velocity is $d(aχ)/dτ$ where $χ$ and $τ$ are FLRW coordinates and $a$ is the scale factor. These are simply not the same quantity, even though they both, in this case, describe the motion of the same object.
The FLRW coordinate that I called $τ$ actually coincides with the proper time of objects moving with the Hubble flow, and one way of understanding "superluminal" recession in cosmology is to recognize that there's no limit on recession speed relative to proper time, even in special relativity. See this answer.
