Are these conservation laws always true?

Question

Imagine a system of particles with the internal force on $i^{th}$ particle due to $j^{th}$ particle being given as $f_{ij}$

From the derivation of law of conservation of momentum and law conservation of angular momentum, I know that

Momentum would be conserved only if internal forces obey third law
$f_{ij} = - f_{ji}$ ...(1)

Similarly, for angular momentum to be conserved, internal forces should be central.
$\vec f_{ij}\times \left( \vec r_i - \vec r_j \right)= 0$ ... (2)

But later we study about lorentz forces, where sometimes (mentioned below) these conditions are not met, we still hold on to momentum conservation and assign momentum to fields. I don't understand how we can use law of conservation of momentum here when the assumption 1 itself breaks down.

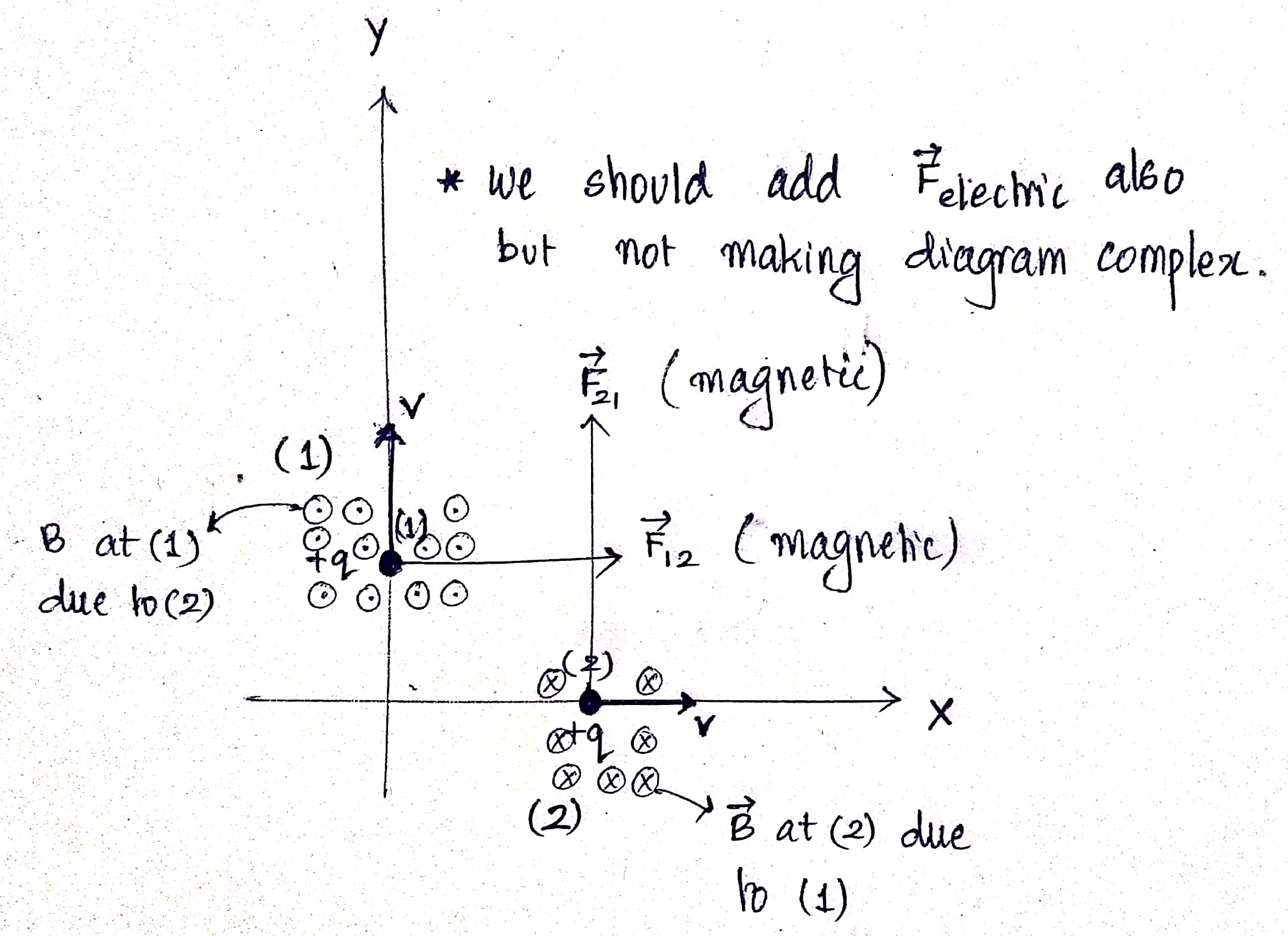
I see that assumption 1 is newton's third law which should be always true...but can we prove third law without assuming momentum conservation?

It seems to be a cycle where if we believe that momentum conservation is always true, everything goes fine.

Now similarly in another question, It was answered that forces on charge are central because law of conservation of angular momentum has to be valid. But shouldn't it be in the opposite way?

In general why do we believe these conservation laws to be always true?

Answer 1

The conservation of mechanical momentum $\mathbf P_\mathrm{mech} = \sum_i m_i \mathbf v_i$ is guaranteed if the internal forces between the particles obey Newton's third law, $\mathbf F_{i\to j} = - \mathbf F_{j\to i}$.

As you've noted, for the Lorentz force between two moving charges, Newton's third law does not hold, and therefore neither does the conservation of mechanical momentum. This is handled by including the electromagnetic field itself as a dynamical entity, and in particular one which can hold momentum in its own right. This electromagnetic momentum $\mathbf P_\mathrm{EM}$ then combines with $\mathbf P_\mathrm{mech}$ to make the total momentum $$\mathbf P_\mathrm{total} = \mathbf P_\mathrm{mech} + \mathbf P_\mathrm{EM},$$ and it is this total momentum that's conserved.

However, saying

It seems to be a cycle where if we believe that momentum conservation is always true, everything goes fine.

is inaccurate. We don't "believe" that momentum conservation is true: instead, there is a rigorous calculation within the laws of electrodynamics that proves that it holds, once a suitable electromagnetic momentum density has been identified.

---

There is also another ingredient at play, in terms of when you ask

In general why do we believe these conservation laws to be always true?

or, more generally, why do we keep looking for additional places where momentum might be "leaking away" to. This is because of a central result in physics known as Noether's theorem, which guarantees that for a certain broad class of systems (those describable by lagrangian or hamiltonian mechanics), if the laws of motion of the system have a symmetry, then there must be a conservation law that corresponds to that symmetry. (There is also a weak converse in the other direction, explained here.)

In the case of momentum, the symmetry at play is translation invariance: experimental results should not depend on where the experiment is run. Noether's theorem then gives you a precise recipe of how to construct the conserved quantity (momentum) from the action of the symmetry transformation and the laws that govern the system.

In other words, if for some reason in a given system the total momentum is not conserved, then the laws that govern it are not translationally invariant. This would not be wrong, per se, but it has never been observed (below cosmological scales), and as such this would require some exceptionally strong experimental evidence before it was accepted.

Answer 2

Conservation laws may be proven from space and time symmetry. If all directions in space are equivalent this leads to angular momentum conservation law. This is more general than electromagnetism and applies to other situations as well Check Noether theorem for this

Answer 3

Conservation depends on what model are using. If EM is not part of the dynamics, so particles affect each other directly, momentum conservation is OK. If you use EM field as part of the dynamics and carrier of particle-particle interactions, the system will radiate momentum and energy into the infinity. It's not cleat for me how you handle EM interactions, but you should take these into account.