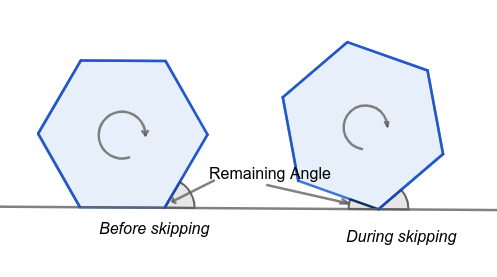
Recently I was viewing this video which discusses Galileo's solution to Aristotle's wheel paradox and it got me thinking about when rolling turns into skidding. Why do geometric shapes with a finite number of edges skip and when an object has its several sides going to infinity, it skids.
Is there a precise number of sides to for which skips turn into skids in rolling motion? Or is this a consequence of the limiting behavior? How?
Reference:
Around 3:00 of this video
The skipping happens because the angle between the sides of a polygon is $<180^\circ$ and to proceed with rotation it has to rotate the remaining angle on its vertex. For it to be a smooth rolling motion that angle should be $180^\circ$ on a flat surface because that's the angle made by a straight line. That only happens when you have a smooth surface like a circle or ellipse, not pointy polygons. Theoretically, any $n$-sided polygon no matter how large $n$ is will always skip. You might not be able to tell the difference between a 50 sided coin but it's still there. If the coin was way bigger then you would notice the skip.
A Pentacontagon 50-sided polygon
However, that does not mean that polygons can not roll smoothly. See this, square wheels!
Their conclusion was that a circle can be considered as a polygon with an infinite number of sides. If there is no material deformation, there is no number of straight sides less than infinite that will not result in the skipping effect in the video.
