The motion of an object moving up and down through an infinite plane

Question

Consider an infinitely large plane which exerts gravity onto a point mass with mass $m$ and located at height $h_0$. The point mass moves perpendicularly through the plane without undergoing any friction.

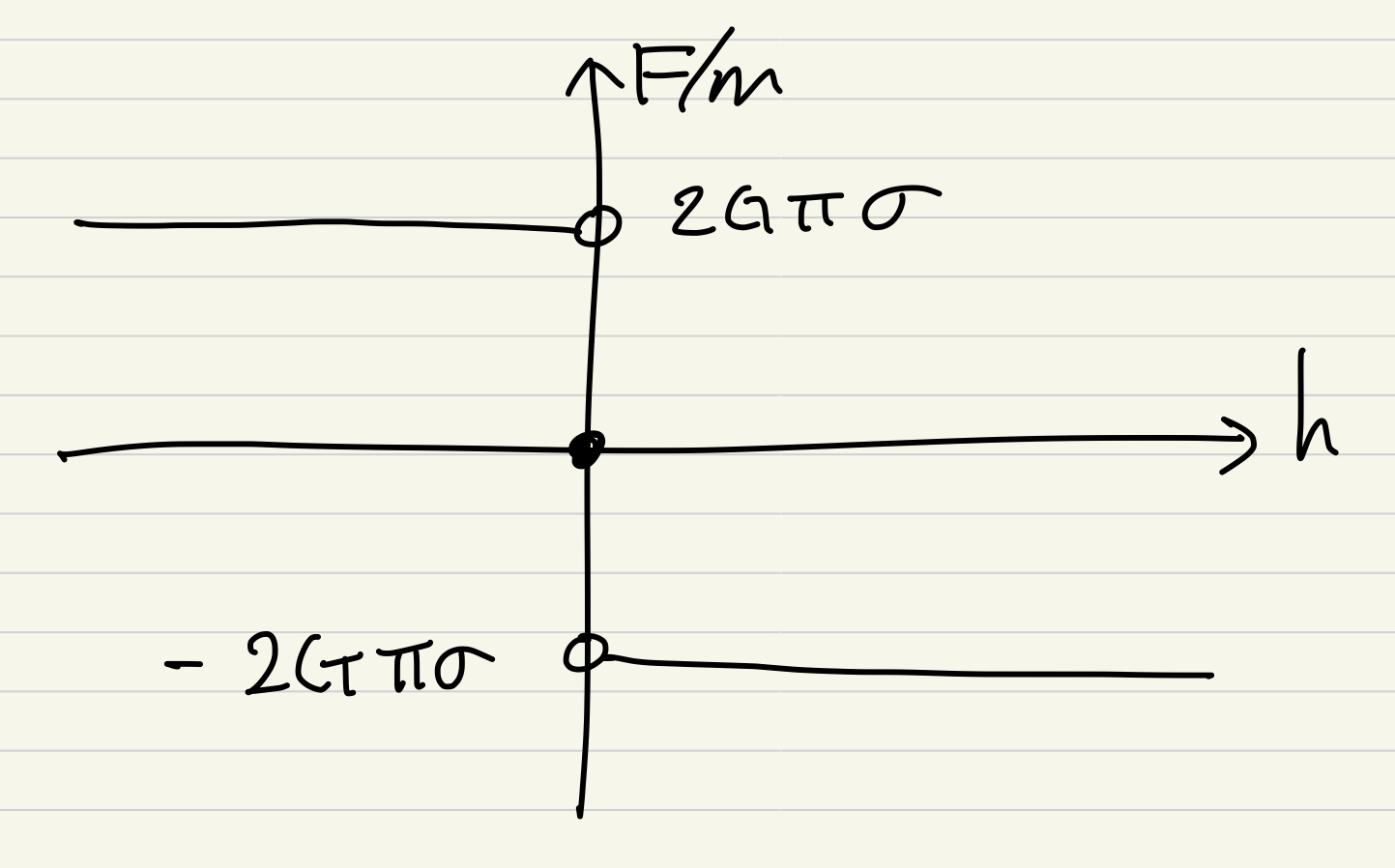
It can be easily derived that the force exerted by the plane is a constant and thus independent of the height at which the point mass is located. I am going to take for granted the result of the calculation, which is $|\vec{F}| = 2Gm\pi\sigma$.

Then it is an obvious conclusion that the $a-h$ graph will look as follows:

Now to specify the problem I will provide the initial conditions: at $t = 0$ $h=-h_0 \ (h_0 > 0)$ and $v = 0$.

I want to find the relationship between $h$ and $t$, i.e. draw the graph of $h(t)$.

What I thought was that initially, while the object is at $-h_0 \leq h < 0$, $$\frac{1}{2}gt^2=0-(-h_0) \Rightarrow t = \sqrt{\frac{2h_0}{g}}$$

and when the object becomes located at $0 \leq h < h_0$ for the first time, the following equations will be valid:

(calling the magnitude of velocity at $h=0$ as $v_0$) $$\frac{1}{2}mv_0^2 = mgh \Rightarrow v_0 = \sqrt{2gh}$$

and as the motion is of uniform acceleration,

$$v(t) = v_0 - gt \ (g = 2G\pi\sigma)$$ $$\Rightarrow -v_0 = v_0 - gt \Rightarrow t = \frac{2v_0}{g}$$

Substituting the expression for $v_0$ into the above, $$t = 2\frac{\sqrt{2gh}}{g} = 2\sqrt{\frac{2h_0}{g}},$$ which is exactly the twice of the time taken for the first interval ($-h_0 \leq h < 0$). So we can deduce the following graph.

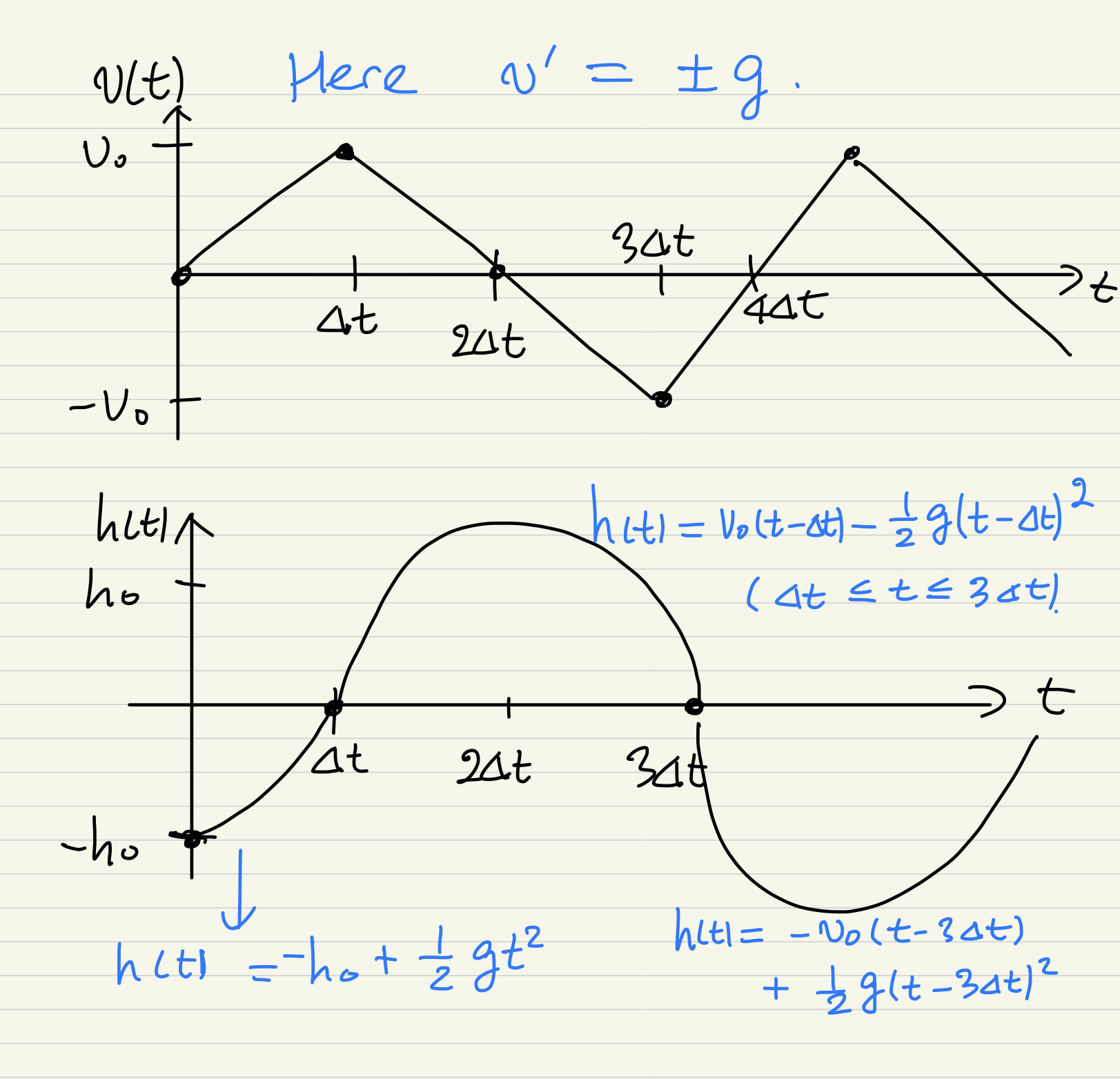
Let's then perform simple integrations to find $v(t)$ and thus $h(t)$. The main problems here would be to find appropriate integration constants so that at the end both $v(t)$ and $h(t)$ are a continuous function. With a bit of physical intuition about the initial conditions, we can conclude that the graphs will look as follows:

Thus the generalisation would be

$$h(t) = -h_0 + \frac{1}{2}gt^2, \ if \ 0 \leq t \leq \Delta t$$ $$h(t) = (-1)^{n+1} v_0 (t-(2n-1)\Delta t) + \frac{(-1)^n g}{2}(t-(2n-1)\Delta t)^2 \ for \ n \in N, \ if \ t \geq \Delta t$$

This is what I came up by myself, and it wasn't even a proper problem in textbooks. I would like you to check if there is any error in my analysis. Also please feel free to tell me if there is any better or simpler (perhaps less algebraic and more physical?) way to reach the same conclusion.

Answer 1

You are obtaining non-harmonic oscillations in potential of type $V(x) = \alpha |x|$. This is correct, and the problem makes perfect sense. I do not want to go through the details of your calculations though - I think it is against the rules of this site.

Answer 2

There is a much and much more simple way to solve your problem. Clearly as you say the acceleration of the point mass is given by $$\vec{a}=\begin{cases} -2\pi\sigma G\;\hat{j} & \forall\;h>0 \\ 2\pi\sigma G\;\hat{j} & \forall\; h<0 \\ \end{cases} $$ Where $\hat{j}$ represents the unit vector in the positive y direction of the usual right hand coordinate system or simply in your diagram the upward direction perpendicular to the infinite plane. For reference to the coordinate system that I have used, refer the following diagram:

Since the acceleration is a piece-wise defined function, lets analyse the first case where $h>0$. The acceleration is constant hence the following equation becomes valid:

$$\vec{S}\;=\;\vec{u}t + \frac{1}{2}\vec{a}t^2$$ where $\vec{S}$ represents the displacement vector and $\vec{u}$ represents the initial velocity.The equation can be derived via integration. If we take $t=0$ at $\vec{h}=h_o\hat{j}$ and $\vec{u}=0$ we get the following., $$\vec{h}\;=\;h_o\hat{j}-\pi\sigma Gt^2\;\hat{j}$$ Also note that to obtain the above after the substitution of values, you would need the following definition of the displacement vector: $$\vec{S_t}=\vec{r_t}-\vec{r_{i}}$$ where the subscript $i$ denotes the initial condition and the subscript $t$ denotes being function of time and the vector $\vec{r_t}$ denotes the position vector with respect to the origin as shown in the diagram above.

A similar analysis can be used to obtain a relation for the second case of acceleration, i.e., when $h<0$.

Hope this answers your question! Use the comment box if something still concerns you.