On conservation of angular momentum and energy

Question

The problem is: Two particles with mass $m$ are attached to a spring of negligible mass, with lengh $l_0$ without streching. The spring is streched until it reaches twice it's initial lenght and it's released after a velocity perpendicular to the sprinc of $(v_0, - v_0)$ is transmited to the particles, such that $kl_0^2 = mv_0^2$, where $k$ is the spring constant. Calculate the components $(v_r, v_\theta)$ of the velocity of the particle when the spring passes through it's non-streched position, where $v_r$ is the radial velocity and $v_\theta$ is the tangencial velocity

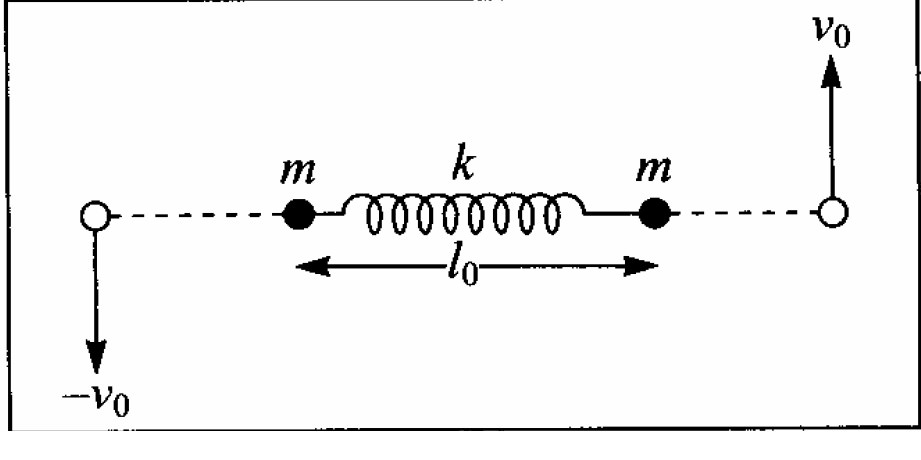
My attempt:

Since the force that act onthe masses is radial, the angular momentum is conserved such that:

$$\frac{I_i v_i}{R_i} = \frac{I_f v_f}{R_f}$$

Where $I$ is the moment of inertia of the system, $v$ the velocity and $R$ the radius of gyration. The initial moment being the moment of releasing and the final, the moment where the spring is in it's non-streched position. This implies that:

$$v_\theta = 2v_0$$

By conservation of energy, we thus have:

$$U_i + K_i = U_f + K_f$$

Hence:

$$\frac{kl_0^2}{2} + \frac{mv_0^2}{2} = \frac{mv^2}{2}$$ $$kl_0^2 + mv_0^2 = mv^2$$ $$7mv_\theta^2 = mv^2 = m(v_r^2 + v_\theta^2)$$

We thus have:

$$v_r = v_0 \sqrt{3}$$

The books answer says that $v_r = 0$, but I think it's wrong.

I would be glad if you help me, thanks in advance.

Answer 1

The book is right. The centripetal force needed to keep the masses spinning at a constant radius is given by $F_{cent}=\frac{mv_0^2}{r}=\frac{kl_0^2}{l_0}=kl_0$. This is exactly the force exerted by the spring.

Answer 2

The book answer is wrong (or maybe you have made a mistake in understanding). I will treat only one particle below as the problem is symmetric under exchange of particles. This means the spring constant has to be multiplied by 2. Energy conservation gives

$$ v_r^2 + v_\theta^2 + \frac{2kl_0^2}{m} (\frac{r}{l_0} - \frac{1}{2})^2 = const $$

Using your relation $k l_0^2 = m v_0^2$ and angular momentum $L=m l_0 v_0$ we can write this as

$$ v_r^2 + \frac{v_0^2}{(\frac{r}{l_0})^2} + 2 v_0^2 (\frac{r}{l_0} - \frac{1}{2})^2 = const $$

We can write the scaled radial position $\rho = \frac{r}{l_0}$ and get

$$ \frac{3}{2} v_0^2 $$

for the constant by putting $v_r=0$ and $\rho=1$. So we get the solution for the problem

$$ \frac{v_r}{v_0} = \sqrt{ \frac{3}{2} - \frac{1}{\rho^2} - 2(\rho- \frac{1}{2})^2} \\ = \frac{\rho-1}{\rho} \sqrt{ - (2 \rho^2 +2 \rho +1)} $$

Since the quantity in the square root is always negative, the only solution is $\rho=1$.

An interesting thing to look at is if we say $\frac{kl_0^2}{mv_0^2}=\gamma$. Then we have

$$ \frac{v_r}{v_0} = \sqrt{ (1+ \frac{\gamma}{2}) - \frac{1}{\rho^2} - 2 \gamma(\rho-\frac{1}{2})^2} \\ $$

and I plot below the allowed regions in solid and disallowed in dashed for three values of $\gamma$.

We see that when $\gamma<1$ (the spring is lax) the allowed region is on the outside of $r=l_0$ while when $\gamma>1$ (spring is tighter) the allowed region is on the inside of $r=l_0$. Of course when $\gamma=1$ (the case in point) we get the allowed region is $r=l_0$.