What is the general formula for time-dilation due to velocity and gravity together?

Question

I see a formula in the Wikipedia entry on time dilation: $$ \sqrt{1-v^2-v_e^2-\frac{v_r^2\cdot v_e^2}{1-v_e^2}} $$ where $v$, $v_e$, $v_r$ are the actual velocity, the escape velocity from gravity, and the radial component of the actual velocity (all normalized against c). Descending into a black hole with $v=v_e=v_r=-\sqrt {r_s/r}$, the result quickly drops to zero at $r/r_s = 3/2 + \sqrt {5/4}$, i.e. well before I get to the event horizon. I assume I am misusing the formula or interpreting it incorrectly?

Answer 1

You have misunderstood the equation that Wikipedia gives:

$$ \frac{d\tau}{dt} = \sqrt{1 - \beta^2 - \beta_e^2 - \frac{\beta_{||}^2 \beta_e^2}{1-\beta_e^2}} \tag{1} $$

In this equation $\beta$ is the radial velocity and $\beta_{||}$ is the tangential velocity (both in units of $c$). So if you are considering an object falling radially inwards it would have a zero tangential velocity and equation (1) simplifies to:

$$ \frac{d\tau}{dt} = \sqrt{1 - \beta^2 - \beta_e^2 } \tag{2} $$

Also Wikipedia is being very misleading in calling $\beta_e$ the escape velocity. It is numerically equal to the Newtonian escape velocity, but this is not equal to the actual escape velocity of the object. It is just a convenient way of referring to the quantity:

$$ \beta_e = \sqrt{\frac{2GM}{r}} = \sqrt{\frac{r_s}{r}} $$

So equation (2) becomes:

$$ \frac{d\tau}{dt} = \sqrt{1 - \beta^2 - \frac{r_s}{r} } \tag{3} $$

The velocity of the object falling in from infinity is given by:

$$ \frac{v}{c} = \left(1 - \frac{r_s}{r}\right)\sqrt{\frac{r_s}{r}} \tag{4} $$

See for example my answer to Will an object always fall at an infinite speed in a black hole?. Deriving equation (4) is somewhat involved for the beginner so I'm leave that as an exercise for the reader.

Anyhow, for your example of an object falling freely into the black hole that will give us:

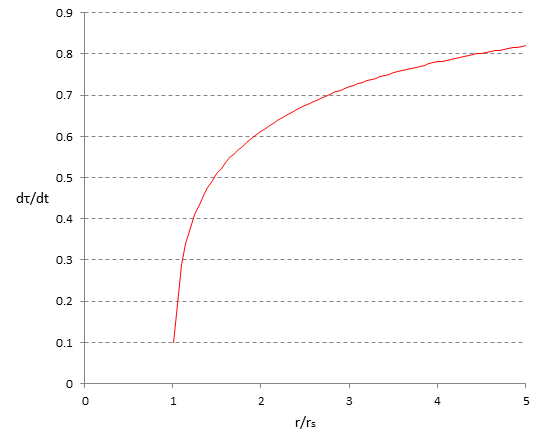
$$ \frac{d\tau}{dt} = \sqrt{1 - \left(1 - \frac{r_s}{r}\right)^2\frac{r_s}{r} - \frac{r_s}{r} } \tag{5} $$

I graphed this to see what it looks like and I got:

So the ratio $d\tau/dt$ goes smoothly to zero at the event horizon as expected.