I recently asked my physics teacher this question, and he could not give me a clear answer: How gravity acts on a streched rope?
Consider example like this one below: if we strech a rope of mass m between points distance d apart, how low will the rope hang? And what will be the tension on the rope? Is rope a perfect parabola in this scenario? I just find it extremely quaint and I couldn't find the answer anywhere.
The shape of a freely hanging massive rope in gravity is a catenary. Parabola comes as a natural guess to early learners. Even Galileo once thought it to be a parabola.
Tension is varying at every points but calculation of tension at end points can be done by force balance equations. $$Derivation:$$ Consider a freely hanging massive rope with uniform linear mass density $ {\lambda}$. Let $x$ and $y$ axes represent horizontal and vertical co - ordinate axes. Point$(0,0)$ be the lowermost point i.e. the point where $\frac{dy}{dx} = 0$
Observe : By horizontal force balance horizontal component of tension is same everywhere. Assign it a variable $T_H$ which can be calculated easily by vertical force balance at the end points and torque balance equation about center of mass (You will need this equation in calculation of $T_H$ in asymmetrical case.)
Between any arbitrary $x$ and $x+dx$: $$T_H \left(\frac{dy}{dx}_{x+dx} - \frac{dy}{dx}_x\right) = {\lambda}dsg$$ Since, $$ds^2= dx^2+dy^2$$ $$or , ds = dx \sqrt{1+\left(\frac{dy}{dx}\right)^2}$$ The above given equation becomes - $$T_H \left(\frac{dy}{dx}_{x+dx} -\frac{dy}{dx}_x\right) = {\lambda}gdx\sqrt{1+\left(\frac{dy}{dx}\right)^2}$$ $$or, \frac{d^2y}{dx^2}=\frac{{\lambda}g}{T_H}\sqrt{1+\left(\frac{dy}{dx}\right)^2}$$
Now to further progress from here , making use of $u$ substitution , put $\frac{dy}{dx} = u$. $$\frac{du}{dx} = \frac{{\lambda}g}{T_H} \sqrt{1+u^2}$$
The solution of this differential equation is - $$u = \sinh \left(\frac{{\lambda}g}{T_H}x\right)$$ and thus applying proper boundary conditions- $$y=\frac{T_H}{{\lambda}g} \cosh \left(\frac{{\lambda}g}{T_H}x\right) - \frac{T_H}{{\lambda}g}$$
See , the given equation is for symmetrical case . By making the situation asymmetric there will only be difference in boundary conditions.
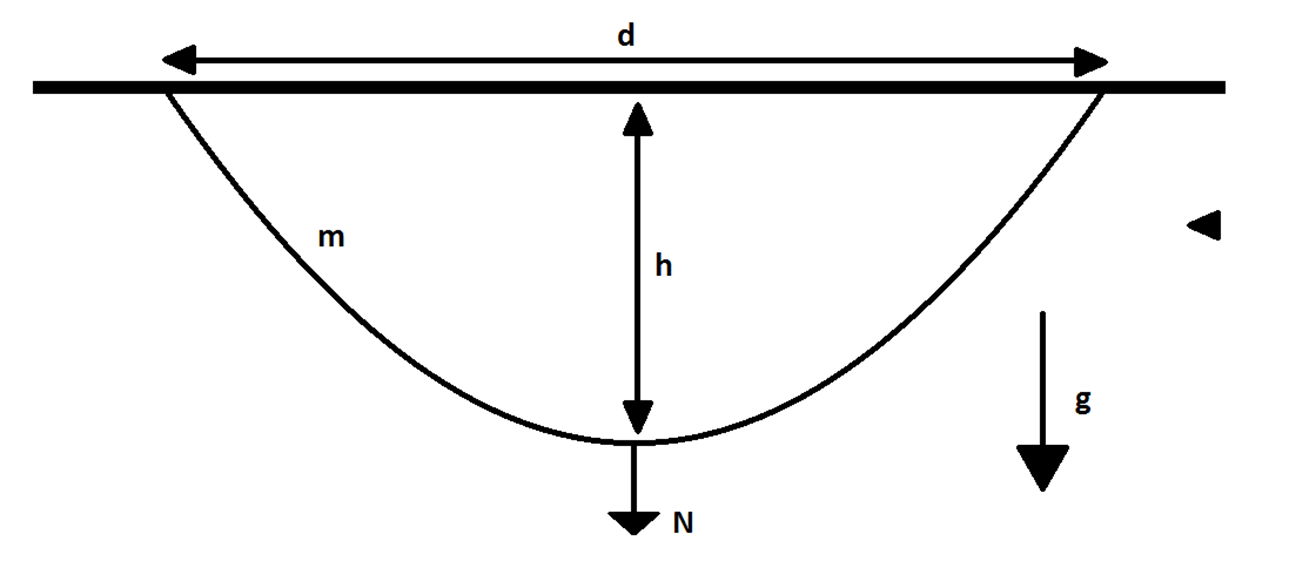
The shape is the catenary.
To derive it take a small section of the rope and balance the forces. The shape of the rope follows the curve $y = y(x)$.
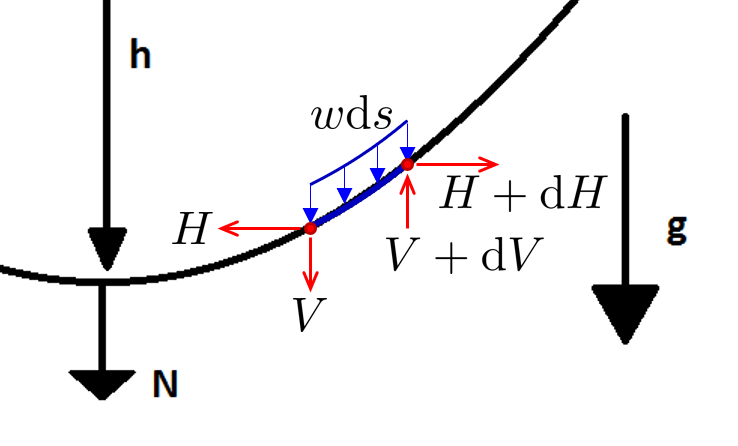
Here the segment with length ${\rm d}s = \sqrt{{\rm d}x^2 + {\rm d}y^2} = (\sqrt{1 + y'^2}) {\rm d}x$ has weight per length of $w$ and thus the total vertical external force applied is $w {\rm d}s$.
The segment is pulled to the left by tension with horizontal component $H$, and to the right by the horizontal component $H+{\rm d}H$. But since there are not external forces along the horizontal, it means that $${\rm d}H = 0 \tag{1}$$, or that the horizontal component of the tension is always constant along the rope.
Similarly, the left side is pulled down by the cable by the vertical component $V$ and pulled up by $V+{\rm d}V$. The balance of forces in the vertical direction is $(V + {\rm d}V) - V - w{\rm d}s =0$ or $$ {\rm d}V = w {\rm d}s \tag{2}$$
The tension is also always tangent to the rope which means that at any point
$$ (\text{slope}) = y' = \tfrac{V}{H} \tag{3} $$
Next re-arrange the above as $V = H y'$ and take the derivative with respect to $x$ on both sides
$$ \require{cancel} \tfrac{ {\rm d}V}{{\rm d}x} = H y'' + \cancel{\tfrac{ {\rm d}H}{{\rm d}x}} y' $$ or
$$ w \tfrac{ {\rm d}s}{{\rm d}x} = w \sqrt{1+y'} = H y'' \tag{4}$$
The solution to the above is given by an equation of the form $$y(x) = y_0 + a \left( \cosh \left( \frac{x-x_0}{a} \right) -1 \right) \tag{5}$$
The $\cosh$ function is what gives it the catenary shape. I leave it up to the reader to evaluate $y'$ and $y''$ and prove that (5) solves (4).
Note that the point $(x_0,\,y_0)$ is the lowest point on the curve, and the parameter $a$ is called the catenary constant (with length units) describes the radius of curvature of the rope at the lowest point.
It is interesting to note that the questioner’s intuition about the curve being a parabola is correct if the suspended weight varies linearly with $x$ rather than with the curve length $s$. A similar analysis to those in other answers then gives
$\displaystyle \frac{d^2y}{dx^2}= \text{constant}$
A practical example of this is a suspension bridge where the weight of the cables is much smaller than the weight of the horizontal suspended roadway.
You are looking for this word: catenary
Deriving this from Newton's laws is not trivial. Fortunately, there's a derivation on Wikipedia.
