1
$\begingroup$

My question is the following:

What is the shape of the rope which holds a kite flying? (Steady state.)

I am not a physicist (I am a mathematician), so I can not work the physics part of the question.

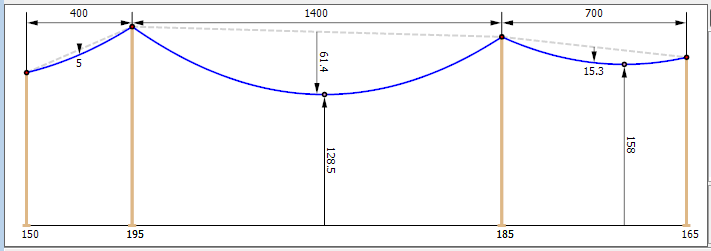
My guess is that it should be the same as catenary (hyperbolic cosine)

Improve this question
$\endgroup$
4
  • $\begingroup$ Possible duplicates: physics.stackexchange.com/q/51485/2451 and links therein. $\endgroup$
    – Qmechanic ♦
    Commented Mar 3, 2014 at 20:52
  • 3
    $\begingroup$ Your guess is correct $\endgroup$
    – pho
    Commented Mar 3, 2014 at 20:56
  • 2
    $\begingroup$ ""it should be the same as catenary (hyperbolic cosine) "" It is not the same, it is a catenary, but only in math. In physics You have to know the drag of the wind on every piece of the rope. Because this drag is a rather nonlinear function of wind velocity, things are complicated. $\endgroup$
    – Georg
    Commented Mar 3, 2014 at 20:58
  • $\begingroup$ @Georg but you know that in physics, all ropes are massless, frictionless, 1-dimensional objects. :-) . Tho' in this case I guess we have to give it mass (so the catenary doesn't collapse to a line segment), but let's keep it frictionless and inextensible. $\endgroup$
    – Carl Witthoft
    Commented Mar 3, 2014 at 21:05

1 Answer 1

Reset to default
2
$\begingroup$

The catenary shape between two points with coordinates $A=(0,0)$ and $B=(S,h)$ is

$$ y(x) = y_C + a \left( \cosh \left( \frac{x-x_C}{a} \right)-1 \right) $$

where

$$ \begin{gather} a = \frac{H}{w} & \mbox{Catenary Constant} \\ H : & \mbox{Horizontal Tension} \\ w : & \mbox{Weight per Unit Length} \\ x_C = \frac{S}{2} + a \sinh \left( \frac{h \exp(\eta)}{a (1-\exp(2\eta))} \right) & \mbox{Lowest Point x coordinate} \\ y_C = -a \left( \cosh\left( -\frac{x_C}{a}\right)-1\right) & \mbox{Lowest Point y coordinate} \\ \eta = \frac{S}{2 a} & \mbox{strain factor} \end{gather} $$

The results look like this: Catenary

Improve this answer
$\endgroup$
2
  • $\begingroup$ I've been (idly) wondering about an extension of these solutions: what is the value of $h$ ( the relative height of the second anchor point) at which the catenary's lowest point equals $h$ -- now all I have to do is find the extrema of $y_C$ $\endgroup$
    – Carl Witthoft
    Commented Feb 28, 2016 at 14:39
  • $\begingroup$ Yes, that is the tower uplift condition and it occurs when $x_c=0$ or $x_c=S$ depending on the side it happens. You can solve for it and find the constant $a$ that this happens for (numerically), or $h$ itself. The solution I got was $$ h = \pm a \left(\cosh\left(\frac{S}{a}\right)-1\right) $$ $\endgroup$
    – John Alexiou
    Commented Feb 28, 2016 at 22:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.