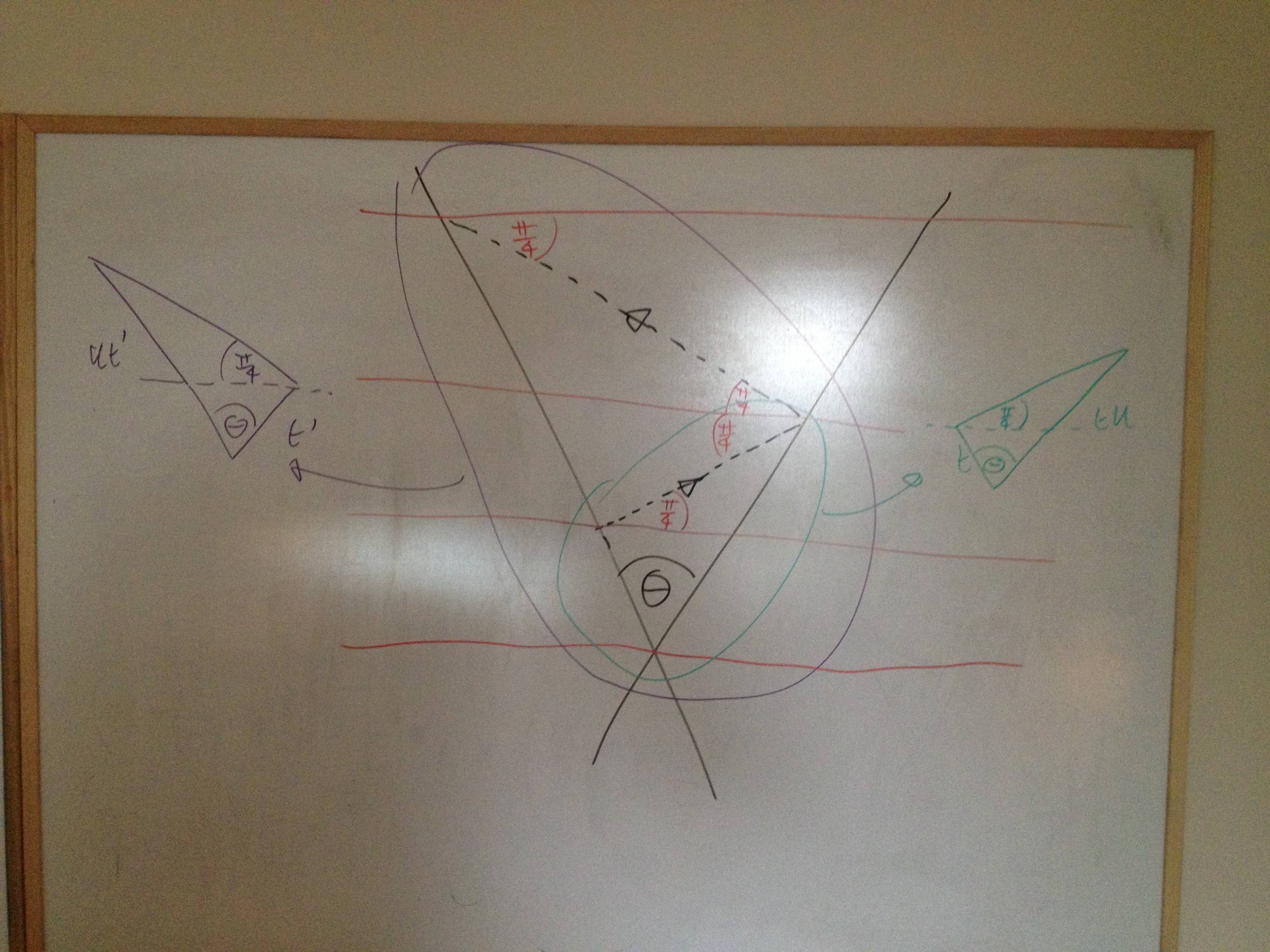
Your formula
$$ t' = \frac{t+t_2}{2} $$
implicitly uses the time measured by the observer with the vertical world line – which is the same thing as the vertical $y$-coordinate. However, the tilted/moving observer measures a shorter time $t'$ by the time dilation factor $\sqrt{1-v^2/c^2}$.
I know that my method of calculation isn't following the pedagogical goal of Bondi to convert everything to the Bondi's $k$-factor, the Doppler factor
$$ k = \sqrt{\frac{1+v/c}{1-v/c} }$$
but I haven't learned relativity in Bondi's way and the main goal is to fix the discrepancy which should be allowed to be done in any way. So let me say that the time dilation occurs by the factor $\sqrt{1-v^2/c^2}\lt 1$. So let's revert the relationships. For the $k$ defined above, we have
$$ \frac vc = \frac{k^2-1}{k^2+1},\quad \sqrt{1-\frac{v^2}{c^2}} = \frac{2k}{k^2+1} $$
This is the time dilation factor you have omitted so the right equation replacing
$$ t' = \frac{t+t_2}{2} $$
is
$$ t' = \frac{k}{k^2+1} (t+t_2) $$
This is right. Substitute $t_2=kt'$ and you get
$$ t' = \frac{kt}{k^2+1} + \frac{k^2}{k^2+1}t' $$
i.e., after multiplication by $k^2+1$,
$$ t' (k^2+1-k^2) = kt,\quad t'=kt$$
which you wanted to get.
Inverted calculation
For Freeman, let me also revert the logic and order of the calculation. By the definition of Bondi's $k$, we may assume $t'=kt$. We want to calculate the time dilation factor $1/\gamma \lt 1$ – I want to avoid new symbols. The time $t'=kt$ may also be expressed as
$$ t' = \frac{1}{\gamma} \frac{t+t_2}{2} $$
i.e.
$$ kt = \frac{1}{\gamma}\frac{t+k^2 t}{2} $$
where I used $t_2=kt'=k^2 t$. From the last equation, it is easy to calculate
$$\frac{1}{\gamma} = \frac{2k}{k^2+1},$$
just like expected.