Relativity and light speed communication

Question

Say Alice could instantly encode another human being, Bob, into photons.

Say Alice then beams those photons to a receiver on another planet, Vogon, one light year away. Charlie is controlling the receiver on Vogon and when Bob's photons arrive, he immediately sends the photons back to Earth. When they arrive Bob is reconstituted into flesh and bone.

Assume instant encoding and decoding times and that Charlie sent Bob's photons back the very instant they were received.

What are the timeframes observed to have passed for each of the participants?

Alice: 2 years would have passed?

Bob: No time would have passed?

Charlie: does it make sense to say anything about Charlie because he is in a different location?

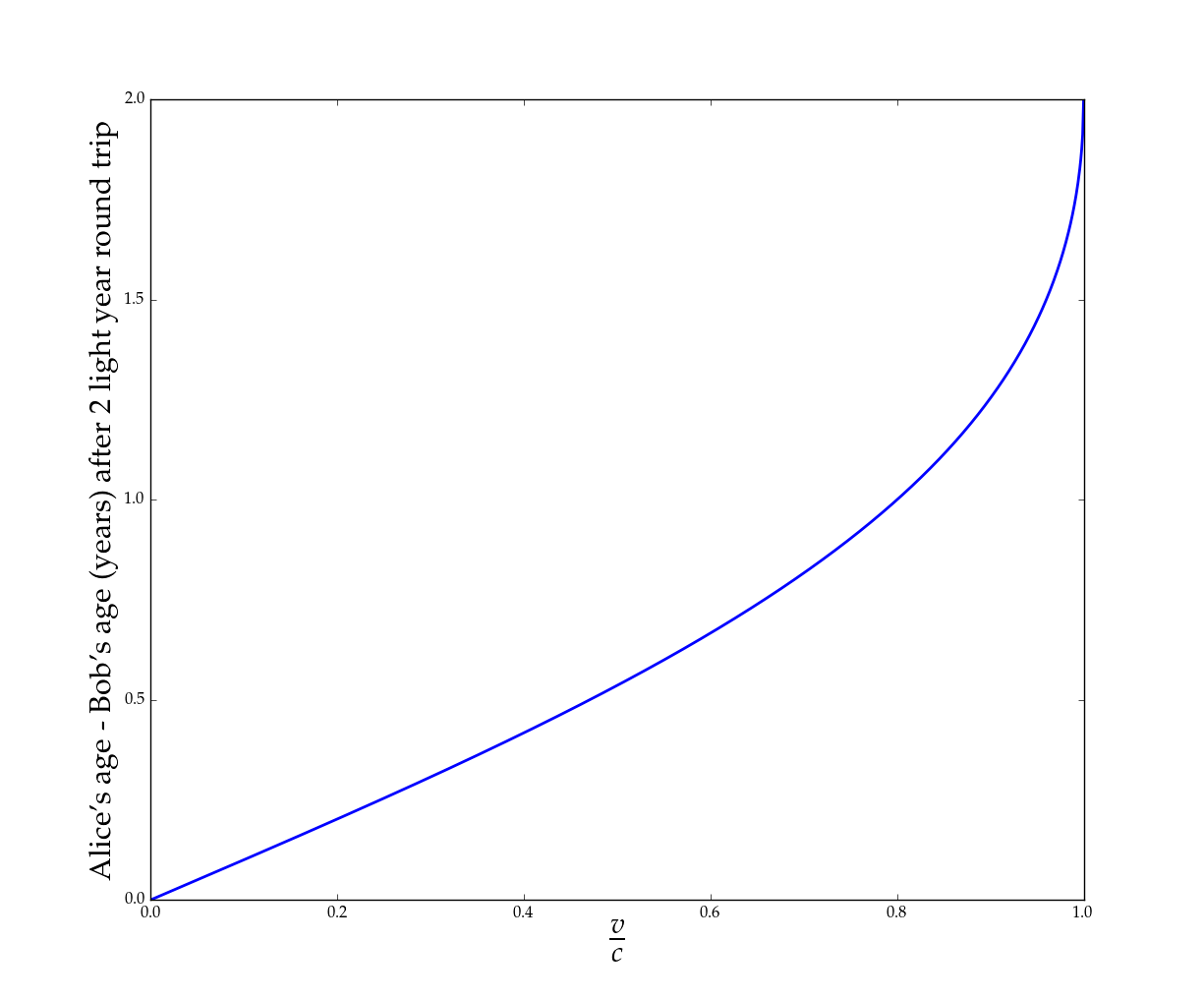
If this is true, is there a continuum of effect (i.e. differing progression of time for Alice and Bob) as speed of propagation of Bob changes, and if so what is the relationship?

Edit: Assume the two planets are stationary with respect to one another.

Answer 1

This is the answer for the "continuum" bit of your question or what happens if Bob leaves for Vogon on a sublight spaceship.

I've assumed that Alice and Charlie are one light year away from each in the rest frame of either and that they are at rest with respect to each other (ie the separation will remain one light year for the entire experiment).

In general if you send Bob encoded in massive objects $m \neq 0$ which move in Alice's frame at speed $v < c$ then Alice will see the (entire) journey taking $\frac{2c}{v}$years.

For Bob the journey seems a little different. On the outward leg he sees himself at rest and the planet Vogon move towards him at speed $-v$. However because the distance between the planets was measured to be one light year in Alice's frame Bob will see the distance contracted by a factor of $\gamma = \frac{1}{\sqrt{1 - \frac{v^2}{c^2}}}$ (note $\gamma \geq 1$) so from Bob's point of view the distance will be $d = \frac{1}{\gamma}$light years and he will see the journey take:

$$\frac{dc}{v} = \frac{c}{v\gamma}\text{years}$$

Note that as $v \to c$ $\gamma \to \infty$ and Bob sees the time taken go to $0$.

The same calculations apply on his return journey so when he gets back he will have aged by only:

$$\frac{2c}{v\gamma}\text{years}$$ whilst Alice will have aged $$\frac{2c}{v}\text{years}$$

I plotted the difference between their ages after the trip (assuming they're the same age before Bob leaves) in the attached picture.

Answer 2

Alice: 2 years

Bob: No time has passed

Charlie: 2 years

There are a couple of things to point out:

1. First two planets aren't likely to be stationary with respect to each other, they will be rotating at different rates around different suns that are themselves in a solar system with it's own movement. But for a realistic scenario (e.g. another planet in another solar system) then the time difference is probably not that much (e.g. still close to 2 years).

2. Technically Bob doesn't exist for the 2 years the signal is in transit. The photons aren't Bob. I'm assuming he is encoded and the original destroyed, so that's the end of the line for him (no more time will ever pass) and the recreation of Bob is a duplicate, not the original. However this starts to become a philosophical debate at this point (is the recreation the same as the original?) so let's not go there. The recreation will have Bob's memories and will not have noticed any time passing.

3. If you can encode Bob, then you could simply store the encoding on a computer then recreate 2 years from now (or any amount of time required). Much simpler and doesn't suffer from interference/attenuation of the signal being sent such a long distance.

Summary: In it's simplest form Bob simply has a 'gap' in his time line. E.g. he has skipped 2 years (or whatever amount of time) that has passed for the other two observers.