If we take a point on the ground $12$ m from the barn as the origin, then the position of the snowball at time $t$ seconds after it leaves the roof is
$(12 - 6 \cos (20^o) t, \space 15 - 6 \sin(20^o) t - \frac 1 2 g t^2)$
Since the man runs at constant speed $v$ starting at $t=0$, the position of the man's feet and head will be
$(vt, 0)\\(vt, 1.9)$
The minimum value for $v$ will occur when the snowball lands at the man's feet. To find this minimum $v$, find $t_0$ such that
$15 - 6 \sin(20^o) t_0 - \frac 1 2 g t_0^2 = 0$
then find $v_{min}$ such that
$12 - 6 \cos (20^o) t_0 = v_{min}t_0$
Similarly, the maximum value for $v$ occurs when the snowball hits the man on the top of his head, so find $t_1$ such that
$15 - 6 \sin(20^o) t_1 - \frac 1 2 g t_1^2 = 1.9$
then find $v_{max}$ such that
$12 - 6 \cos (20^o) t_1 = v_{max}t_1$
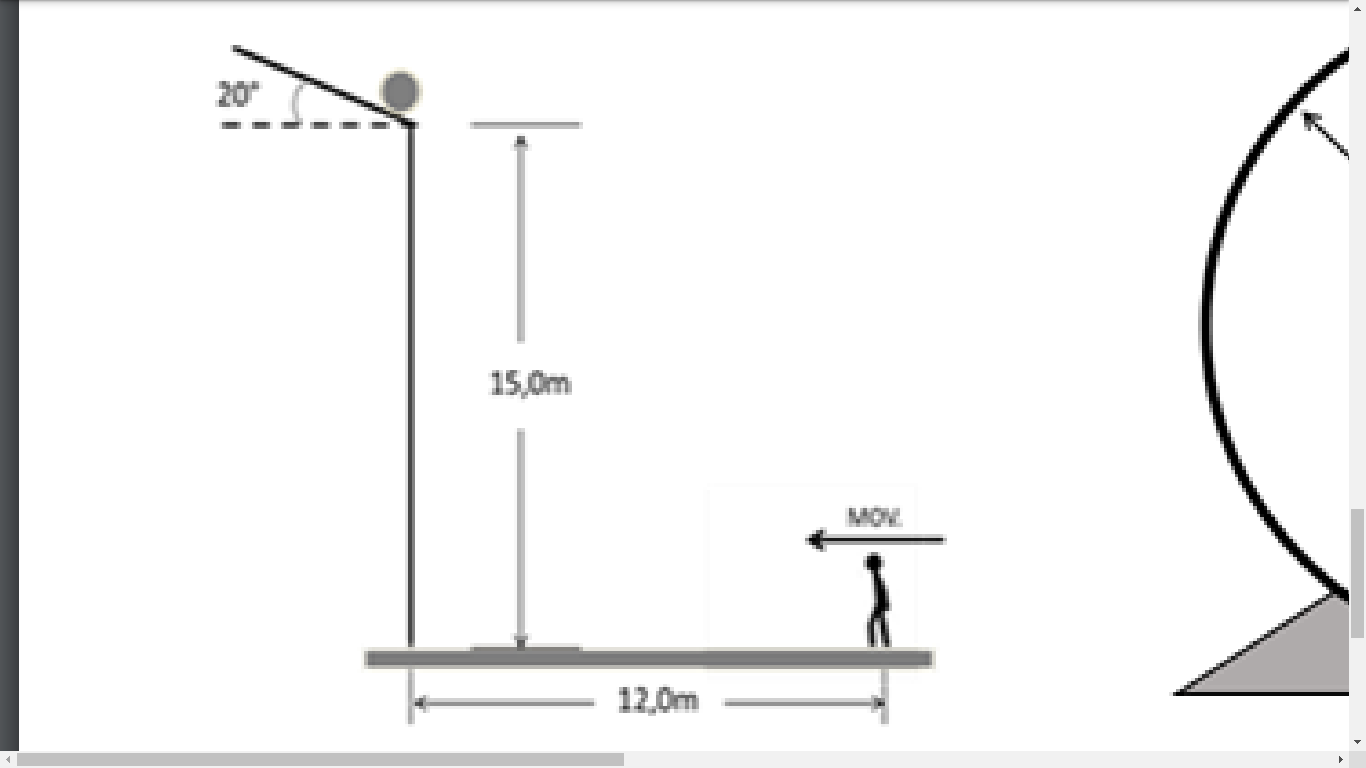 A snowball rolls from the roof of a large barn that has a downward slope of 20 °. The roof end is 15.0 m above the ground and the snowball has a speed of 6.00 m / s when abandoned from the roof. At the same moment, a man of 1.90 m tall is 12.0 m from the barn running towards it with speed v, of constant modulus. What must be:
A snowball rolls from the roof of a large barn that has a downward slope of 20 °. The roof end is 15.0 m above the ground and the snowball has a speed of 6.00 m / s when abandoned from the roof. At the same moment, a man of 1.90 m tall is 12.0 m from the barn running towards it with speed v, of constant modulus. What must be: