What does the Atomic Form Factor mean?

Question

I was reading Nuclear Physics and the author mentioned something about the atomic form factor, something in relation to the Fourier transform of the spatial distribution of the electric charge. but I don't know what it means. What is the physical interpretation of the atomic form factor?

Answer 1

There are a variety of ways one could answer this. As you note, the form factor is the Fourier transform of the spacial distribution of the electric charge density. The subtlety with this definition is that for a composite system such as a nucleus (or atom, or nucleon), defining a "net charge density" that is just a function of spacial coordinates, i.e. $\rho(x, y, z)$, is an approximation. The exact description of an atom or nucleus is given by a many-body wavefunction.

That aside, $\rho(x, y, z)$ for an atom or nucleus can be roughly interpreted as the charge density associated with an effective potential that is arrived at by averaging over the motion of the constituent particles. The form factor $f(Q)$ is Fourier transform of this.

Consequently, one should expect the form factor associated with an atom to deviate from zero around $1/(10^{-10} \textrm{m})$ and $1/(10^{-15} \textrm{m})$. The former corresponds to electronic degrees of freedom (e.g. the "wavefunction" of the electrons) and the latter corresponds to nuclear degrees of freedom.

The form factor $f(Q)$ will provide a measure of the interaction of between the atom and an incident photon with momentum $Q$. Consequently, photons with a wavelength $\sim 10^{-10} \textrm{m}$ (i.e. visible or UV light) or $\sim 10^{-15} \textrm{m}$ (i.e. gamma rays) will interact strongly with the atom by coupling to the electronic or nuclear degrees of freedom, respectively.

One can also define other kinds of form factors that correspond to different sorts of interactions (e.g. magnetic, the strong force, etc.).

Answer 2

The simplest way to think of a form factor is to interpret the meaning of the term. It is a multiplicative factor that modifies an expected area/crossection.

. One then can design an experiment to measure the area seen by this scattering. This area is the crossection of the target. In classical physics when hitting a target and measuring the recoils one will get for the crossection the effective area of the target. A form factor would be a factor needed for the calculated and the measured crossection to agree, if the target had peculiarities on the surface, for example , which effectively would make it seem larger in scattering. So a form factor would be necessary to explain the data within the model used.

In quantum mechanical situations one can have a simple theory, for example Rutherford scattering, just based on simple classical scattering theory and expect the experiment to provide a measure of the size of the target. In nuclear and particle physics the only way to measure a target is by scattering off it. The simple model, using a central potential for example, will give an expected crossection for this scattering. The real data will deviate, and a form factor function will be measured, which is the difference between the simple theory expectations for the crossection and the real data. Then more advanced theories of charge distributions enter the picture, and a form factor can be theoretically calculated and checked with the measured form factor for deviations or agreement.

In Rutherford scattering of an electron on an atom , the simple formula

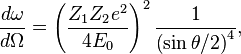
will be modulated by the square of the Form Factor. In other words, electron scattering off an extended source is equal to scattering off a point source modulated by the form factor (formula 13 in pdf link). This form factor integrates over charge distributions that are not point like, which is the assumption entering Rutherford scattering. Thus form factors can be used to explore the finer structures of the target.

Form factors were an important tool in studying the weak interactions with neutrino proton scattering, for example, but as the standard model has been successful in describing the existing data and predicting new ones, they have fallen out of fashion as a research tool.