I have a question following the derivation of the Klein-Gordon equation from a lagrangian. From
Eq. (13d), where does $\delta^\mu_\nu$ come from? I guess it's a conversion factor of some sort.
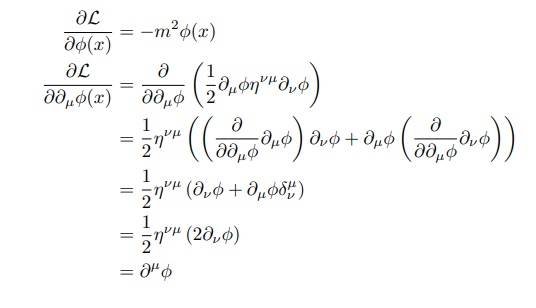
-
4$\begingroup$ I hope that scan didn’t come from a textbook, because it has terms in which there are three $\mu$’s. This is not allowed in tensor calculus, and it invalidates the derivation. $\endgroup$– G. SmithCommented Aug 18, 2020 at 17:09
-
2$\begingroup$ Here is a MathJax tutorial. Scans of math should not be posted on this site. $\endgroup$– G. SmithCommented Aug 18, 2020 at 17:16
2 Answers
Formally when you derive by the derivative of the field you have to choose a DIFFERENT index from the ones already used in the Lagrangian.
So for instance for the following massless Lagrangian you have
$$ \mathcal{L}=g^{\mu\nu}\partial_{\nu}\phi \partial_{\mu}\phi$$
And the derivative should be:
$$\frac{\partial \mathcal{L}}{\partial\left(\partial_{\alpha}\phi\right)}=... $$
Now when you consider $$ \frac{\partial \mathcal{L}}{\partial \partial_{\alpha}\phi}= g^{\mu\nu}\frac{\partial_{\mu} \phi}{\partial_{\alpha} \phi}\partial_{\nu} \phi \\ + g^{\mu\nu}\frac{\partial_{\nu} \phi}{\partial_{\alpha} \phi}\partial_{\mu} \phi $$
The $\frac{\partial_{\mu} \phi}{\partial_{\alpha} \phi}$ term amounts to the term $\delta^{\alpha}_{\mu}$.
So you get a term with one index which is $\alpha$, which you than have to derive with respect to $\alpha$ like so: $$ \partial_{\alpha}\left( \frac{\partial \mathcal{L}}{\partial \left(\partial_{\alpha}\phi\right)}\right)=\frac{\partial \mathcal{L}}{\partial \phi}$$
If you consider $\partial_\mu\phi$ as a coordinate $y_\mu$ then the second term on the third line reads as $$y_\mu\left(\frac{\partial}{\partial y_\mu}y_\nu\right).$$ By definition of (partial) derivatives this is just $$y_\mu\delta^\mu_\nu$$ with $\delta^\mu_\nu$ the Kronecker delta.
-
1$\begingroup$ @CarlF, think about what this would mean for the first term. Hint: $\delta_\mu^\mu=4$. $\endgroup$– G. SmithCommented Aug 18, 2020 at 17:30
