$E$ field boundary condition and Snell`s law

Question

So, for E field boundary condition, we know the vertical part of the incident field

$\varepsilon _{1}E_{1\perp } = \varepsilon _{2}E_{2\perp }$

and the tangential parts are same from both side.

That basically means a larger $\varepsilon$ leads to a smaller vertical part. put that into a figure as following 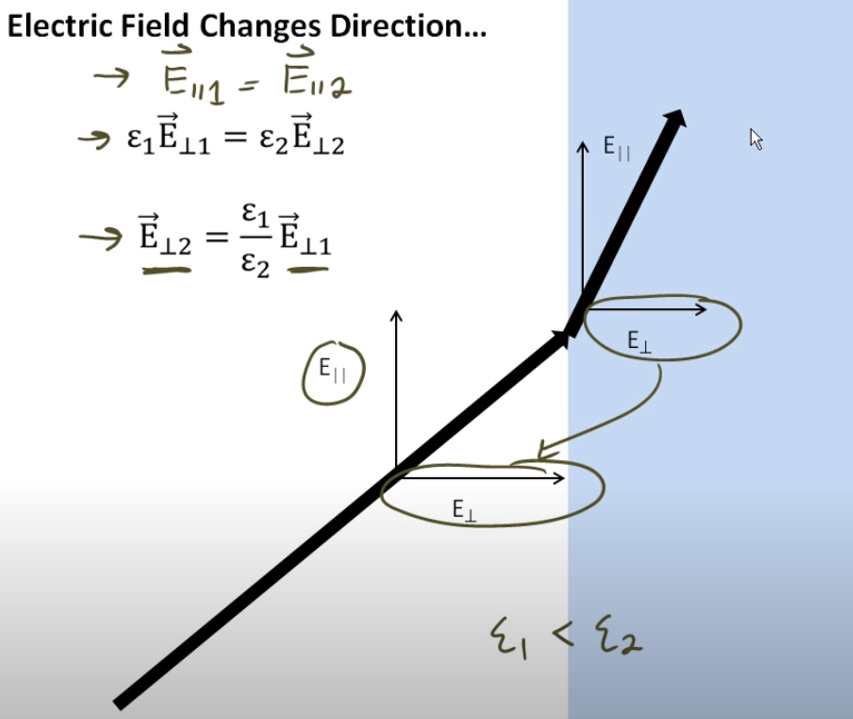As shown in this figure, the incident angle is smaller than transmitted angle. And this is directly opposite to Snells law, where $\beta {_{1}}sin(\Theta _{1}) = \beta {_{2}}sin(\Theta _{2})\\ \sqrt{\varepsilon _{1}}sin(\Theta _{1}) = \sqrt{\varepsilon _{2}}sin(\Theta _{2})$,

however, $sin(\Theta_{1})$ or $sin(\Theta_{2})$ leads to the parallel part of the field.

For example, say the a wave traveling from Air to Water. Since water has a higher $\varepsilon$, therefore the $\Theta_{water}$ is larger than $\Theta_{air}$ as shown on image above. But the Snell`s law shows the opposite.

I kind of know Snell`s Law is coming from the Electric field boundary condition, but I cannot get it through, where I got it wrong?

Answer 1

Snell's law refers to the direction of propagation of the wave, not to the direction of the electric field. If you apply your analysis to the wavevector $\mathbf k$ rather than $\mathbf E$, you should find the correct behavior.

Answer 2

You are being confused because often Snell's law would be shown in a diagram using the electromagnetic wave propagation direction as the lines. Since electromagnetic waves are transverse, the electric field direction is always perpendicular to the wave propagation direction.

Thus if your diagram represented the electric field (at some instant of time) either side of an interface, then the wave directions would be at right angles to the lines you have drawn and you will find Snell's law.