1
$\begingroup$

I was doing Kleppner-D.-Kolenkow-R.J. and I came across the following problem:-

A pendulum is tied vertically to a car at rest, the car suddenly accelerates at a rate A. Find the maximum angle of deflection $\phi$ through which the weight swings.

MY TRY: I saw the solution of this problem in the book which uses car's frame of reference, which was fairly simple.

I tried to do it in the ground frame of reference.

Deflection of the pendulum will be maximum when the angular velocity of the mass hung to pendulum relative to the hanged point will be zero, hence the velocity of mass relative to the car, perpendicular to the string is zero. But the constraint of a taut string doesn't allow velocity of mass relative to the car along the string also. So, velocity of mass relative to car is zero at the point of maximum deflection.

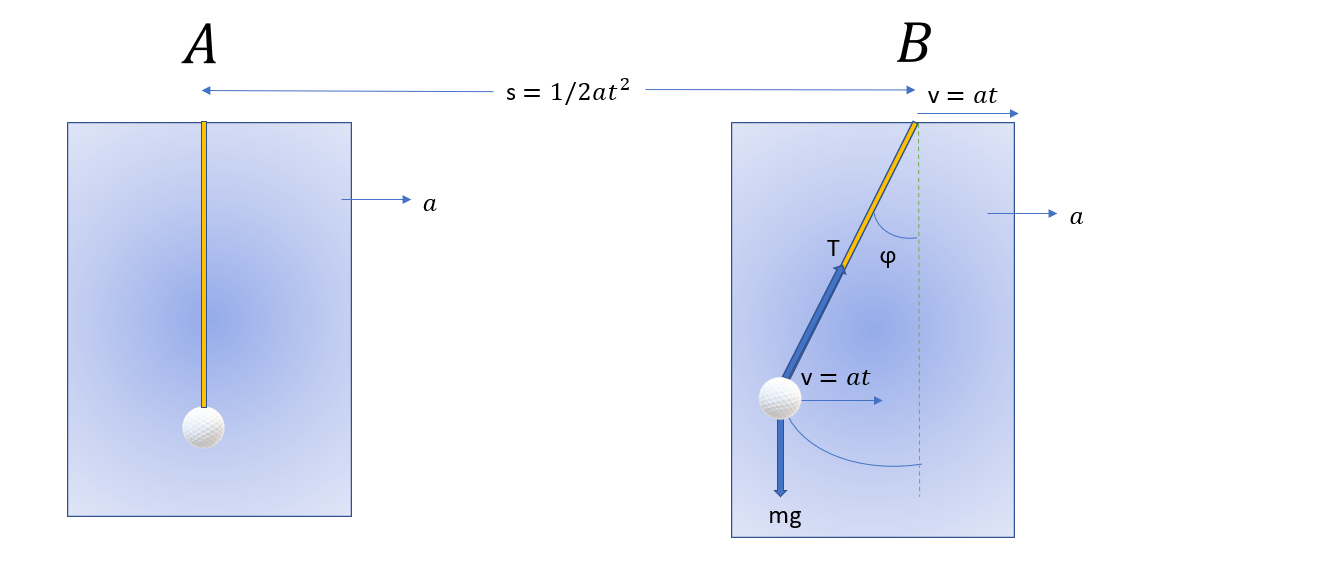enter image description here I have the following two tools to solve the problem:-

  1. Apply Work energy theorem to the mass.
  2. Use the string constraint i.e. the acceleration of the mass and the topmost point along the string will be equal at any instant i.e. $T-mgcos(\theta)=masin(\theta)$
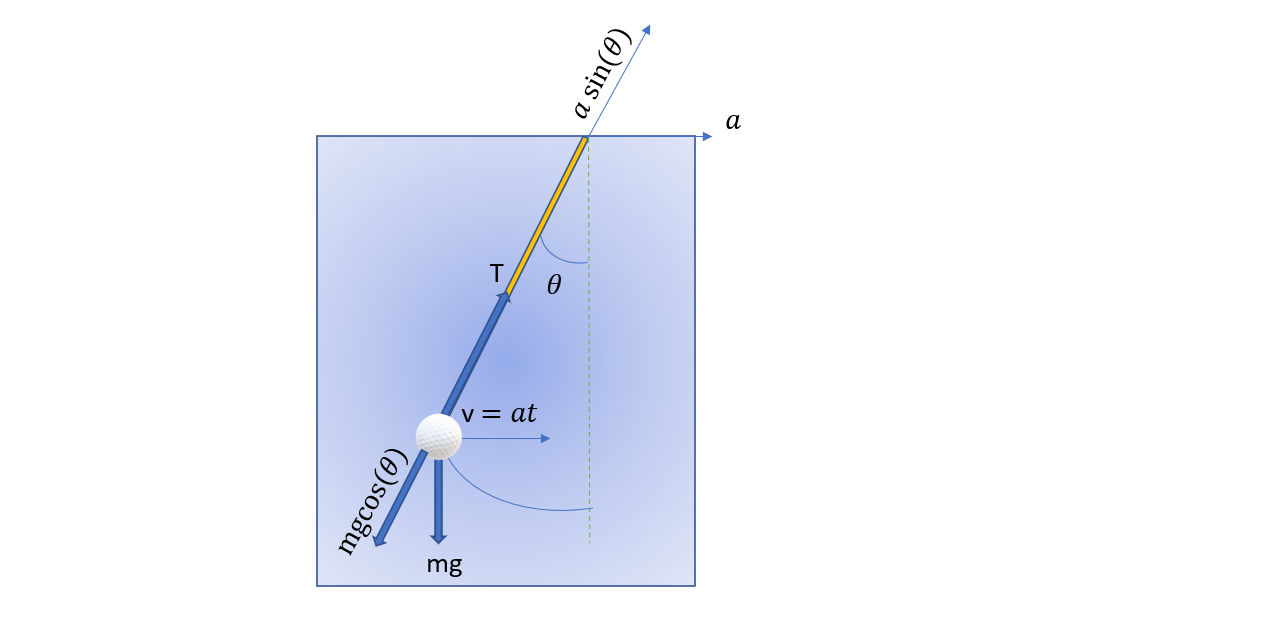
    enter image description here

The tension force and gravity are only two forces acting on the mass. But, how could one find the work done by tension on the mass in the journey from $A$ to $B$. Any hint would be a great help!

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
2
$\begingroup$

General relativity tells us that gravity is equivalent to acceleration. In this case the pendulum suddenly finds itself in a system where gravity has shifted backwards by an angle with a tangent of a/g. Being a pendulum it will swing toward this new equilibrium, and momentum will carry it an equal angle beyond.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Fine! But it is essentially the same method used in the car's frame of reference. Could you please give hints to the answer in ground's reference frame? Or how would we find the work done by tension force? $\endgroup$
    – ImBatman
    Commented Aug 3, 2020 at 18:24
  • $\begingroup$ When working with forces, you want to work with acceleration rather than velocity. Again, in the inertial frame, you look at the “equilibrium” position where the acceleration relative to the car is zero. Then T sin(θ) = ma and T cos(θ) – mg = 0 where a is the acceleration of the car. $\endgroup$
    – R.W. Bird
    Commented Aug 3, 2020 at 20:30
2
$\begingroup$

Work done by tension in car's frame of reference would be zero. Then we can apply conservation of energy where initial and final kinetic would be zero(since at start it didn't have any energy) then use pseudo force's work equal to work done by gravity. We get answer $$\theta = 2\arctan(\frac{a}{g})$$ (where $\arctan$ is $\tan$ inverse function).

Improve this answer
$\endgroup$
3
  • $\begingroup$ Nice to see such cool method of energy conservation in car's frame! But I just wanted to know whether we could solve the problem in ground frame entirely? Could you help in finding work done by tension force in ground frame? $\endgroup$
    – ImBatman
    Commented Aug 4, 2020 at 5:59
  • $\begingroup$ Could you add in your calculations with a bit more detail, since I'm getting a different answer from the same method? $\endgroup$
    – Box Box Box Box
    Commented Oct 27, 2020 at 12:45
  • $\begingroup$ @AshishAhuja The answer would seem to be different untill you apply some trigonometric half angle formullas. $\endgroup$
    – ImBatman
    Commented Nov 2, 2020 at 9:38

Not the answer you're looking for? Browse other questions tagged or ask your own question.