It is a rotational motion problem from Kleppner mechanics:
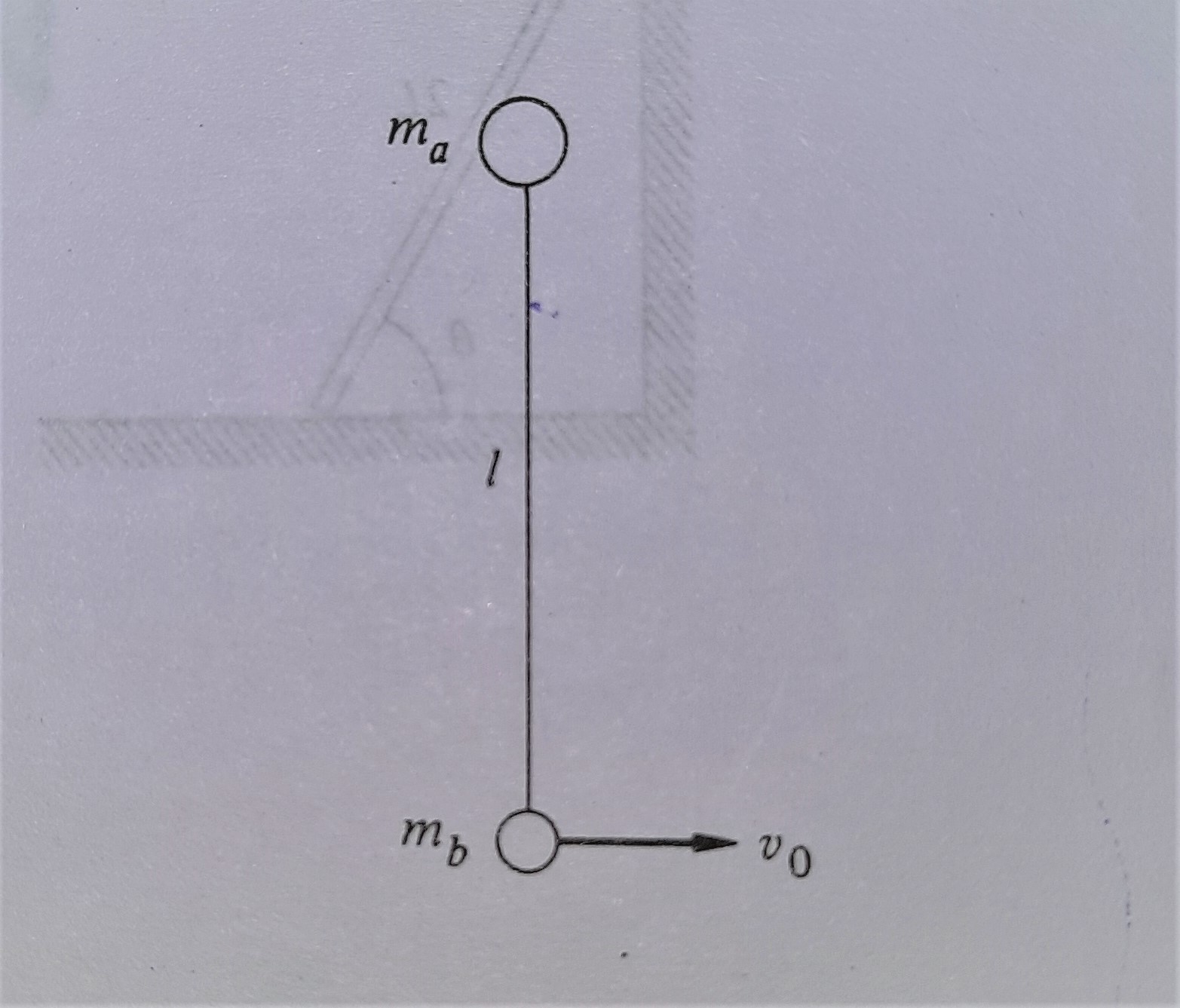
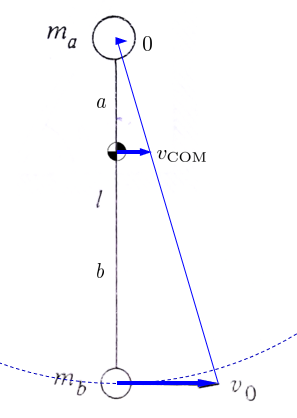
Two masses $m_a$ and $m_b$ are connected by a string of length $l$ and lie on a frictionless table. The system is twirled and released with $m_a$ instantaneously at rest and $m_b$ moving with instantaneous velocity $v_0$ at a right angle to the line of the centres as shown below. Find the subsequent motion of the system and tension in the string.
After I tried for two days I can find tension by polar coordinates system! (Here I assumed there is zero radial velocity of the reduced mass, according to central force problem.)
But my confusion is: As the tension is pulling the mass $m_a$ in direction along the line of the string but it has zero velocity initially; so the string should be collapsed after a certain amount of time but if I think about it the problem is invalid! At this point my mind blows up!
I am really confused. Where I am getting it wrong? 🤕🤕