The original question I was asking was Why does a body not rotate if force is applied on the centre of mass?.
The obvious answer is that the torque ${\bf r}\times{\bf F}$ is zero as ${\bf f}$ is zero at the centre of mass, but that defeats the purpose of my question. Why is the centre of mass so special that we take torque about it and force applied on it does not provide rotation?
I am looking for the intuition behind this.
Okay so let me give you the intuition.
Now you would've heard of cases of a pendulum made of a rod instead of a string.
(You can checkout this: http://hyperphysics.phy-astr.gsu.edu/hbase/penrod.html)
Okay, so in this case you can clearly see that the torque is taken about the hinge. And you apply gravitational force at the centre of mass and take its torque about the end of the rod and the torque is non zero! Eureka! You have an answer.
Now we usually take torque about a hinge so that the forces acting due to the hinge don't appear in torque. In the most common cases, the center of mass is itself the hinge, so we take torque about center of mass.
When you talk about rolling, you again take the torque about center of mass, however you really don't have to. You could choose any point and write the torque and define parameters about that point. However center of mass, often gives us a very easy and intuitive way of calculating the physical parameters and that's why we often write torque about the centre of mass.
I hope I have answered your question. Please feel free to comment if there is any doubt!
Why a body does not rotate if force is applied on the centre of mass?
The question is: Why does it rotate if it is not applied to the center of a mass?
First we look at two rules that students of mechanical engineering (I don't know about physics) learn at university:
In university, these two rules are used to prove that the formula of momentum ($M = r\times F$) is valid...
However, we can use the same methods used in that proof to prove that a body will rotate if the force is not applied to the center of gravity:
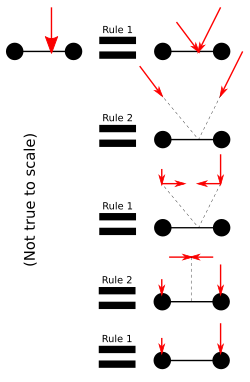
We do that for a very simple body because otherwise we would require integral calculation: We have a body like a dumbbell: Some bar that has nearly no mass in the middle but all mass is concentrated in the two endpoints. (Pos. 3 and 4 of my image)
We do some vector calculations (shown in my second drawing) to prove that one force applied to some point of the body has the same effect as two forces applied to the ends of the body (where the mass is).
If the single force is applied to the center of the bar (if both masses at the ends are equal, this is the center of the mass), the two forces having the same effect as the single force have the same magnitude. (Pos. 3)
However, if the single force is not applied to the center of the bar, the two forces do not have the same magnitude. (Pos. 4)
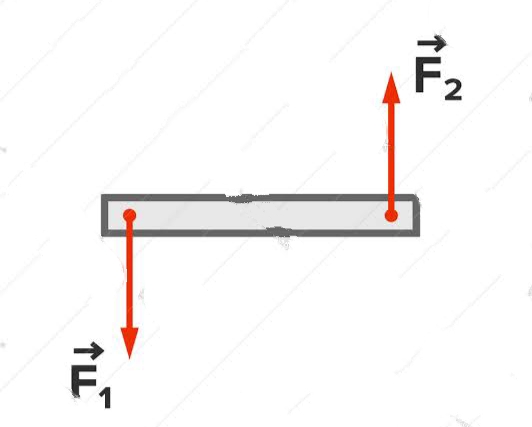
You may say that the left force accelerates the mass on the left end of the bar while the right force accelerates the mass at the right end of the bar.
Because the forces have a different magnitude (but the masses are equal), the acceleration is different and therefore the velocity will be different after a short time.
If two points of a solid body move with a different velocity, this means that the solid body is rotating.
I am looking for intuition behind this.
Most intuitive would be applying a single force at one end of the bar...
Drawings
Positions 1-4 in my answer:
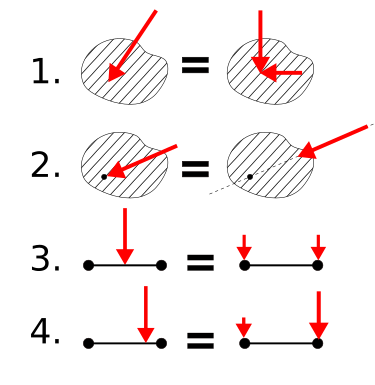
Vector operations required for the proof:
The rotation of a body depends upon the point at which it is hinged. So if a force applied at the center of mass (COM) then it may cause rotation about the axis (if it's not the one through COM) at which it is hinged by causing a torque to act.
But what about the case when it isn't hinged at any point with the force still acting on the COM? A torque still acts about the axes other than that of the COM. Let me answer this question.
I'll simplify the case by taking an example of a rigid rod. The force causes a translation acceleration. And lets say the rod rotates about an arbitrary axis not through the COM. Thus an angular acceleration exists about that axis. But this angular acceleration must add another translation acceleration (from $\vec{a} = \vec{r} \times \vec{\alpha}$) which is not what we observe and hence the rod will not rotate about any other axis even if a torque would act about it.
I hope this clear your doubt!
Other than the specified reasons in previous answers, the major reasons I choose to use the centre of mass frame of reference is because
It is easier to visualize the motion of the object, as it performs pure rotation about the centre of mass.
You do not need to bother about pseudo forces. You can see for yourself that torques due to all the pseudo forces cancel out.
So you can just see pure rotation and no bother of pseudo forces!
How could any other frame be more beautiful than that? (;p pardon special relativity fans)
Center of mass is a point in space, that is it doesn't have a length. Also note that a point is not a circle, it doesn't have a circumference nor area.
If you replace a collection of particles with centre of mass you will end up having a point with same mass.
Now just try to imagine applying a force to the point . The moment you apply a force to it , if you are able to imagine , you will see the object can only translate .
This can be explained with a simple case - torque with zero net force.
Consider a rod in free space being acted upon by two equal and opposite forces at the ends.
The subsequent motion of the rod is dictated by -
No net force on the COM
The rod is a rigid structure
What it means is individual particles of rod must move without changing their relative positions (the rod is rigid) and also the COM of the rod must not accelerate (the net force is zero).
That leaves a single way - rotation about the COM.
Why does a body not rotate when a force is applied at the COM?
It's just like you apply a force on particle and it goes into rotation about some random point.
