Are two waves being in phase the same as saying that the two waves are coherent?

Question

If two waves are coherent, is it the same as them being in phase? Please correct if I'm wrong.

Answer 1

They are two different but closely related concepts

Given two sine waves of equal frequency once can ask what is their relative phase, and are they in-phase or out of phase. So $\sin(\omega t)$ and $sin(\omega t+\pi)$ have a relative phase $\pi$ or 180 degrees and so we would say they are out of phase.

The question of coherence is: how stable is the phase between the two waves? Does it change quickly with time. With the two perfect sine waves above the relative phase never changes? It's always $\pi$. But no real wave is perfectly sinusoidal. Instead think of a signal that looks sinusoidal in any small piece but the phase is slowly drifting. Symbolically we can write $f(t)\equiv sin(\omega t + \phi(t))$ where $\phi(t)$ is a slowly changing function of t. If we have two of these signals we can ask how is the relative phase changing. If it does not change significantly (like if we measure the light on two parts of a laser beam) then we would say that the signals are highly coherent. If the relative is not stable, if they go quickly from being in phase to out phase like the light from a light bulb, than we would say they are incoherent. More quantitatively we can give a coherence time, the time it takes for the phases to become unmoored from each other.

Answer 2

For two waves to be 'perfectly' coherent (I'm assuming electromagnetic, transverse waves) they have to have the same wavelength, polarization and phase.

To go a bit deeper, the coherence can never be perfect.

Only an infinitely long wave has a wavelength that is 'fully defined', ie. has no uncertainty (or width) associated with it. One therefore defines a coherence length (or equivalently a coherence time) over which the phase is likely to be the same. So if you split a realistic wave (say the beam of a HeNe Laser) in two, then delay one of them, and reunite them (e.g. Michelson or Mach-Zehnder Interferometer), the coherence decays as a function of the delay.

Answer 3

Let's place your question in the frame of a Mach-Zehnder interferometer so we can define some important variables. Here is a hastily drawn Mach-Zehnder from MS Paint.

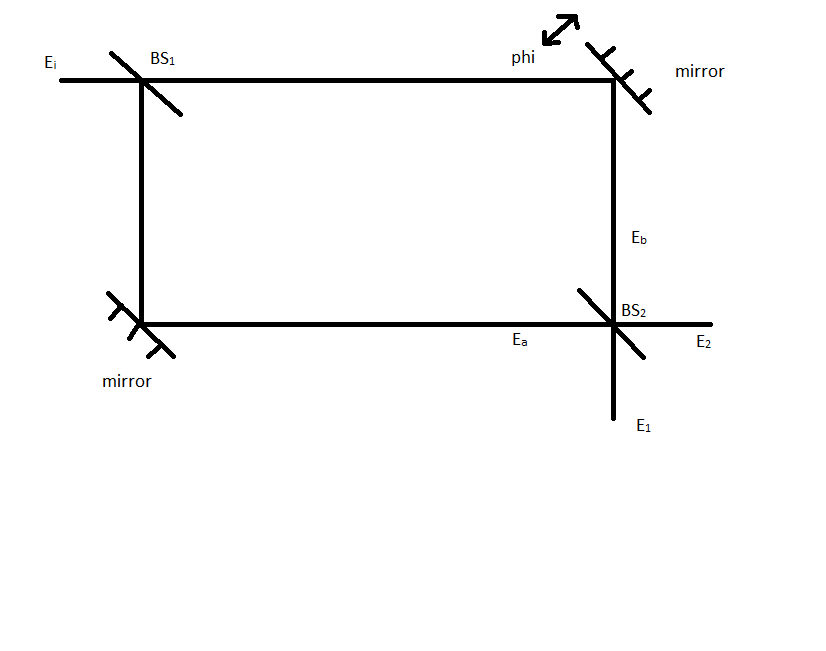
The electric field $E_{i}$ incident on the first beam splitter $BS_{1}$ is split into electric fields $E_{a}$ and $E_{b}$. If we assume the mirrors are fully reflective, no energy is lost, and the fields recombine at $BS_{2}$. By displacing one of the mirrors, we can adjust the path-length difference and thus the relative phase of the two waves when they are incident on $BS_{2}$. The equation governing this phase shift is $\phi=\frac{2\pi\Delta l}{\lambda}$. Now, without loss of generality, let's consider just $E_{1}$. The equation for this beam is $E_{1}=r_{2}E_{a}+t_{2}E_{b}e^{i\phi}$ where $r_{2}$ and $t_{2}$ are the reflection and transmission coefficients of $BS_{2}$ respectively and the factor if $e^{i\phi}$ accounts for the relative phase shift. For simplicity's sake, let's assume the transmission and reflection coefficients for both beam splitters are equal to $\frac{1}{\sqrt{2}}$. Then $E_{a}=\frac{1}{\sqrt{2}}E_{i}=E_{b}$. Though electric field is not conserved, the energy is, and we can represent the energy by the intensity (or power) of the beam. The intensity of $E_{1}$ is given as $I_{1}=|E_{1}|^{2}=\frac{|E_{a}|^{2}}{4}(1+e^{i\phi})(1+e^{-i\phi})=\frac{I_{i}}{2}(1+\cos\phi)$, where $I_{i}$ is the input intensity corresponding to $E_{i}$. Note that if $\phi$ is a multiple of $2\pi$, the waves interfere constructively and all of the input intensity is reflected through $E_{1}$.

Now let's talk about coherence. Let the path length difference between the two arms of our interferometer be nearly zero, and assume the mirrors are stably constructed and not prone to vibrations. The phase difference between the beams is then constant, and we say the beams are coherent. However, let's say that we vibrate one of the mirrors rapidly, with a vibration amplitude greater than the wavelength of the wave. If the vibrations are extremely fast, aka faster than our detector can register, the detector will average out the vibration. The average intensity $I_{1}$ is then equal to $\frac{I_{i}}{2}\langle(1+\cos\phi)\rangle=\frac{I_{i}}{2}(1+\langle\cos\phi\rangle)$. Since the amplitude of the fluctuations is greater than a wavelength, the cosine term will average to zero, and the average intensity emerging from $E_{1}$ is $\frac{I_{i}}{2}$. The same process can be done for $E_{2}$ with the same result. We do not observe interference, and the beams are called incoherent. The last possibility is that the fluctuations are just as fast, but the amplitude of the fluctuations is less than a wavelength. This causes the $\cos\phi$ term to not average to zero, but the interference is preserved, though its visibility is lowered. These beams are said to be partially coherent.

I hope that by defining each term specifically I have answered your question.

Source: "Quantum Mechanics: Theory and Experiment" by Mark Beck

Answer 4

While I agree with BebopButUnsteady, there is a simpler way to summarize what he said. Coherence and relative phase difference are not the same. Take two signals,

$$s_1 = \sin(\omega_1t + \theta_1)\\ s_2 = \sin(\omega_2t + \theta_2)$$

Two waves can be coherent and out of phase. But they cannot have a constant relative phase difference and be incoherent. The crux of the issue is the phase delay, theta, and frequencies, omega, of the two waves. If the two waves have the same frequency $\omega_1 = \omega_2$ and are phase aligned, $\theta_1 = \theta_2$, they are coherent and in phase. If the two have different phase offsets $\theta_1 \neq \theta_2$, but are of the same frequency, $\omega_1 = \omega_2$, then they are coherent and out of phase. If they have different frequencies, $\omega_1 \neq \omega_2$, they are incoherent regardless of the phase shift.

Answer 5

I believe that "in phase" means that the phase difference of the two waves is zero (and out-of-phase means that this difference is equal to pi). But more general if the phase difference of two waves remains the same during a time that is called "Coherence time", the fields are coherent during that time. In reality the fields do not remain coherent for infinite interval and after the coherence time the phase difference drifts.