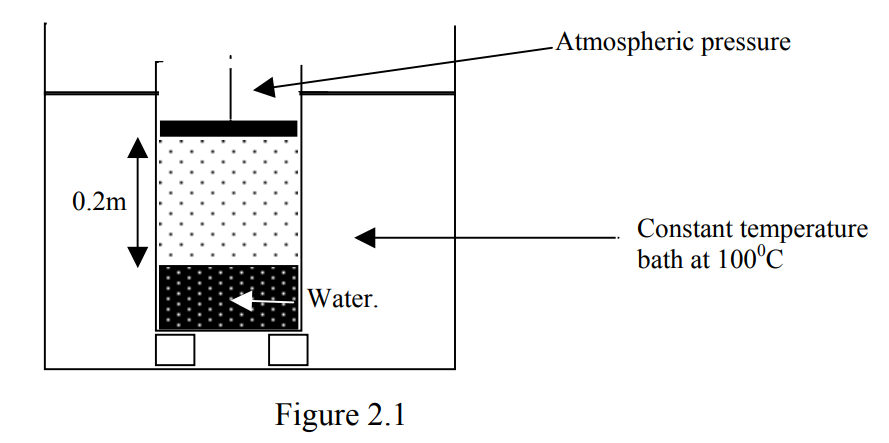
A cylinder, with a weightless piston, has an internal diameter of $0.24 m$. The cylinder contains water and steam at $100 C$. It is situated in a constant temperature bath at $100 C$, Figure $2.1$. Atmospheric pressure is $1.01 × 10^5 Pa$. The steam in the cylinder occupies a length of $0.20 m$ and has a mass of $0.37 g$.(see diagram below).
I'd like to have a better understanding of the setup:
Why is the ideal gas equation, $pV=nRT$ not applicable to this system, does the fact that the steam is not isolated from the water below it (so that condensation occurs) violates the assumption that there are no molecular forces between ideal gas molecules?
The pressure also seems to remain constant at $p=p_{atm}$ as the piston is slowly pushed downwards/ pulled upwards, because the steam condenses/evaporates into water so there are less/more frequent collisions between the steam molecules and the cylinder, is my explanation satisfactory?
The diagram: