15
$\begingroup$

If I have a beaker with pure water in it that has a piston right above the surface of the water that maintains a pressure of 1 atm then below 100 °C I would not expect to have any water vapor. In other words, the piston will keep touching the surface of the water. Above 100 °C the piston will go up a lot and I will have only vapor inside the beaker.

The only coexistence region of water+vapor is at 100 °C. This is all in the thermodynamic limit so I am not considering fluctuations.

However, now consider the following. Suppose I have a special gas that does not interact or dissolve in water. I introduce some of this special gas between the water surface and the piston and still maintain 1 atm pressure. Now let's consider the system at 50 °C.

I cannot see any difference between the two configurations (with and without the special gas) as far as the water is concerned and thus at 50 °C I would not expect any of the water to be in vapor form. And yet my gut/experience tells me that there is some vapor in the gap between the water surface and the piston. To see this imagine putting some ice cubes on the piston. One would expect droplets to condense on the piston.

What am I missing?

Edit 1 Adding phase diagrams for clarification and discussion. It's from James Sethna's book on statistical mechanics.

enter image description here

Edit 2 I am adding a diagram of the setup. The point is that I start with the setup at 50 °C and 1 atm with water at the bottom and an ideal gas that does not dissolve in water at the top with the two separated by a partition. What will happen when I remove the partition. Will some of the water in liquid phase convert into vapor phase? If so, how does one figure out how much of the water will convert into vapor? Assume we know the mass of the water and that of the gas.

enter image description here

Improve this question
$\endgroup$
11
  • $\begingroup$ Are you familiar with Psychrometrics, the study of water vapor in atmospheric air and the Psychrometric chart? $\endgroup$
    – Bob D
    Commented Dec 31, 2019 at 13:09
  • $\begingroup$ No I am not but I do think that this is a simple thermodynamics questions that should be answerable without the specific details of the properties of water and air. I mean I can ask this question about any two fictitious substances where one of them supports a liquid-vapor 1st order phase transition. $\endgroup$
    – Borun Chowdhury
    Commented Dec 31, 2019 at 13:19
  • $\begingroup$ I am concerned that your assumption of a special gas which does not interact in any way with the water is too artificial to produce an answer which meshes with intuition. Assuming a set temperature and pressure for your gas, the fact that it cannot dissolve or condense simply means that it makes a set volume of your cylinder inaccessible to the water. In that sense, how is it different from a solid block of inert material? $\endgroup$
    – J. Murray
    Commented Dec 31, 2019 at 20:23
  • $\begingroup$ @J.Murray The difference between an inert gas and an inert solid is that other gases, including water vapor, can occupy the spaces between the gas's molecules much more easily than between the solid's molecules. $\endgroup$
    – Bob Jacobsen
    Commented Dec 31, 2019 at 22:25
  • 4
    $\begingroup$ What you're missing is that the pressure in the phase diagram corresponds to the partial pressure of the water in the atmosphere, not the absolute pressure. You can be at 1 atm absolute pressure and have very little water vapor in the air, so a very small partial pressure. $\endgroup$
    – Vincent Fourmond
    Commented Jan 1, 2020 at 9:49

5 Answers 5

Reset to default
21
$\begingroup$

As others have said, here's the difference:

Case I: At 50 C and 1 atm, in a system that contains only pure water, all the water will be in a liquid state. That's what phase diagram (a) would show you if the axes were numbered -- you're not on a coexistence line; rather, you're squarely within the liquid region.

Case II: At 50 C and 1 atm, in a system that contains water and air, some of the water will be in the gaseous state.

The natural question is then: Why?

The answer: There's no entropy of mixing in Case I, but there is in Case II.

Specifically: Water molecules will move between phases from regions where their chemical potential is higher, to where it's lower, until the chemical potential is uniform, at which point you've reached equilibrium (with respect to the water).

At 50 C & 1 atm, the chemical potential of pure water in the liquid state is lower than that of pure water in the gas state. Thus, in Case I, where we only have the possibility of pure liquid water and pure gaseous water, all the water will stay in the liquid state.

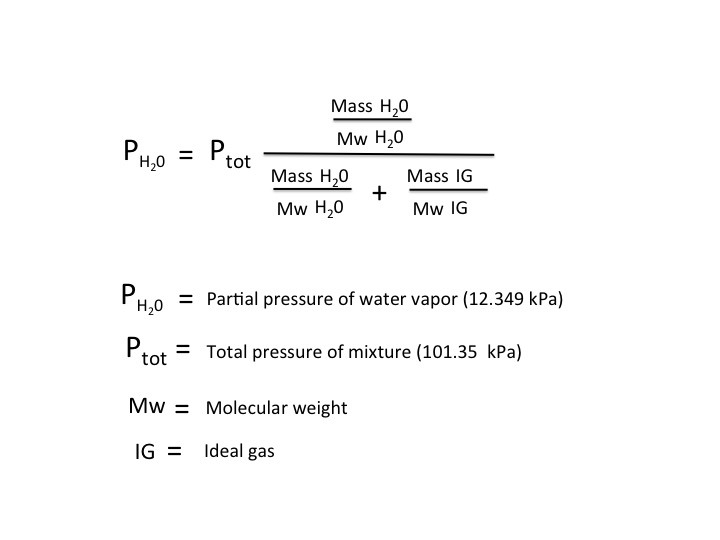
However, let's now consider Case II. Suppose we have some water in the gaseous state. Why doesn't all that gaseous water move to the liquid state, as in Case I? The reason is that the water in the gaseous state is not pure water! It is water mixed with your inert gas. And its chemical potential is lowered (relative to that of pure water vapor) as a result of the increase in entropy associated with this mixing. If the partial pressure of the water is lower than its vapor pressure, it will move from the liquid phase to the gas phase. As this happens, the chemical potential of the water in the gas phase will increase. When the chemical potential of the water in the gas phase (which includes the entropy of mixing) reaches the chemical potential of the liquid water, you have reached equilibrium. At this point, the partial pressure of the water vapor will be equal to its vapor pressure:

Defintion of terms:

$\mu_{H_2O(g)}^{mix}(T, P_{H_2O(g)})$ is the chemical potential of water vapor in a gaseous mixture at temperature $T$ and partial pressure $P_{H_2O(g)}$

$\mu_{H_2O(g)}^o(T)$ and $\mu_{H_2O(l)}^o(T)$ are the chemical potentials of pure gaseous and liquid water, respectively, at temperature $T$ and standard pressure $P^o$ (where $P^o$ = 1 bar; 1 bar is about 1 atm).

Then:

$$\mu_{H_2O(g)}^{mix}(T, P_{H_2O(g)}) = \mu_{H_2O(g)}^o(T) + RT ln \frac{P_{H_2O(g)}}{P^o},$$

where the logarithmic term is the contribution of the entropy of mixing to the chemical potential. You can see that the lower the partial pressure of the water vapor, the lower the resulting chemical potential.

At equilibrium, the chemical potentials of the water in the pure liquid and mixed gaseous states are equal:

$$\mu_{H_2O(g)}^{mix}(T, P_{H_2O(g)}) = \mu_{H_2O(l)}^o(T)$$

and thus:

$$\mu_{H_2O(g)}^o(T) + RT ln \frac{P_{H_2O(g)}}{P^o} = \mu_{H_2O(l)}^o(T)$$

Consequently:

$$RT ln \frac{P_{H_2O(g)}}{P^o} = \mu_{H_2O(l)}^o(T) - \mu_{H_2O(g)}^o(T)$$

At 50 C and 1 atm, the chemical potential of pure liquid water is less than that of pure gaseous water. Hence the RHS is negative. Equilibrium occurs when $P_{H_2O(g)}$ is sufficiently low that the LHS is equally negative. This is the vapor pressure of water at that temperature.

N.B.: You specified that your gas didn't dissolve in liquid water. But what if it were a real gas, and did? In that case, wouldn't the presence of the real gas (say, air) also lower the chemical potential of the liquid water? The answer is yes, but the amount by which the chemical potential of the liquid water is lowered by this effect is relatively small.

Improve this answer
$\endgroup$
12
  • $\begingroup$ Yes I was thinking along these lines as well. I was thinking that the sheer presence of the extra space due to the special gas between the liquid and piston would mean a more entropic configuration when some of the vapor goes up there but again I am trying to get a quantitative handle here. Where can I see the results for chemical potential of vapor lowering by entropy of mixing? I should be able to derive it with some effort but if there is a reference handy please let me know. $\endgroup$
    – Borun Chowdhury
    Commented Jan 1, 2020 at 11:28
  • $\begingroup$ @BorunChowdhury I've added a set of equations that show how the chemical potential of the water vapor is lowered by the entropy of mixing $\endgroup$
    – theorist
    Commented Jan 1, 2020 at 23:56
  • $\begingroup$ Thanks a lot. Could you please explain the derivation of the first equation or alternatively point me to the right source and that should tie up loose ends. I will try to derive it myself and if I do so before you respond I will update the answer. $\endgroup$
    – Borun Chowdhury
    Commented Jan 2, 2020 at 9:27
  • $\begingroup$ Any physical chemistry book will do. For instance, Google Books has the 3rd ed. of Castellan at books.google.com/…. Go to eqn 10.47, which shows the derivation of the free energy of mixing. In an ideal gas, the free energy of mixing is entirely due to the entropy of mixing, because there are no interactions. $\endgroup$
    – theorist
    Commented Jan 3, 2020 at 2:29
  • $\begingroup$ @BorunChowdhury It can also be derived using statistical mechanics. See the answers here: chemistry.stackexchange.com/questions/39524/entropy-of-mixing and chemistry.stackexchange.com/questions/61307/…. Note that $x_i$ is the mole fraction of component $i$ so, in a gas mixture, $x_i = \frac{P_i}{P}$ $\endgroup$
    – theorist
    Commented Jan 3, 2020 at 8:02
16
$\begingroup$

If I have a beaker with pure water in it that has a piston right above the surface of the water that maintains a pressure of 1 ATM then below 100° C I would not expect to have any water vapor.

The only reason you would have no water vapor is because you have no air between the piston and water surface for the vapor to be in.

The only coexistence region of water+vapor is at 100° C. This is all in the thermodynamic limit so I am not considering fluctuations.

That is not true. If it were, there would not be any water vapor in the atmosphere at normal temperature and pressures. Water vapor in air is simply gaseous H2O molecules mixed together with the dry air components (primarily nitrogen and oxygen). You are thinking the only way to get water vapor in air is to boil liquid water. Water vapor is also produced by evaporation, which occurs at temperatures below boiling point.

However, now consider the following. Suppose I have a special gas that does not interact or dissolve in water. I introduce some of this special gas between the water surface and the piston and still maintain 1 ATM pressure. Now lets consider the system at 50° C.

I cannot see any difference between the two configurations (with and without the special gas) as far as the water is concerned and thus at 50° C I would not expect any of the water to be in vapor form.

Again, not true. The amount of water vapor in the "special gas" may be different at a given temperature and pressure than the amount in air, but it can still exist nonetheless.

And yet my gut/experience tells me that there is some vapor in the gap between the water surface and the piston.

Trust your gut. If you want to know how much water vapor there is in air without doing calculations, you can check the Psychrometric chart. For example, for a dry bulb temperature of 25° C and a relative humidity of 20%, the amount of water in the air is about 0.002 kilograms H2O per kilogram of dry air.

As for your evaporation comment, I agree there is evaporation and I am asking exactly how it happens at 50° C and 1 ATM and hence what the flaw in my reasoning is.

At the molecular level, evaporation can occur at 50° C and 1 atmosphere because there is a distribution of kinetic energy of the water molecules around the average value that determines temperature. Some have energies (velocities) above the average, others below. Those with high velocity at the surface of the water may have sufficient energy to escape intermolecular attraction forces and enter the air if the air is not saturated with water vapor (e.g, 100% relative humidity). Simultaneously, those water molecules in the air with lower energy condense and fall back to the surface. The process continues until the rate of evaporation equals the rate of condensation, which is when the air is saturated with water vapor.

You know that water boils at less than 100° C at high altitudes, because the air pressure is lower. At 12.3 kPa the boiling point of water is 50° C. That's the partial pressure of the water vapor in air corresponding to the saturation.

Improve this answer
$\endgroup$
16
  • 1
    $\begingroup$ @BorunChowdhury There is always a liquid-vapor equilibrium present at temp. below the critical temperature of gas. Note the definition of boiling point, which is the temperature at which vapor pressure of liquid equals atmospheric pressure. Vapor is always present at temperatures even below the boiling point $\endgroup$
    – aditya_stack
    Commented Dec 31, 2019 at 19:11
  • 9
    $\begingroup$ @BorunChowdhury - Remember, all models (and this includes phase diagrams) are wrong but some are useful. Models are just that - they are not reality nor explanations of reality but simulations (models) of real-world phenomena. Phase diagrams just happens to be a good model for the behavior of bulk quantities of material but what happens at the surfaces/boundaries are not explained by the phase diagram. We had to create another model/theory to explain that phenomena and that is vapor pressure (which is also as wrong as phase diagrams but also useful) $\endgroup$
    – slebetman
    Commented Dec 31, 2019 at 21:27
  • 4
    $\begingroup$ “... enter the air if the air is not saturated with water vapor...”: more precisely, the energetic water molecule will enter the air anyway, regardless of saturation. It’s just that at 100% saturation, about the same number of (slower than average for vapor molecules) molecules in the air will be captured by the liquid surface, so no net molecule transfer occurs in either direction. $\endgroup$
    – Euro Micelli
    Commented Jan 1, 2020 at 0:43
  • 2
    $\begingroup$ @slebetman A generic statement like "all models are wrong and some are more wrong" are always correct and hence meaningless. The first step is to understand what a model even means before judging how right (or how wrong) it is vis-a-vis reality. $\endgroup$
    – Borun Chowdhury
    Commented Jan 1, 2020 at 11:19
  • 2
    $\begingroup$ @slebetman The phase diagrams are reality -- they are based on experimental measurement. You're obfuscating this by throwing out cliched philosophical statements. $\endgroup$
    – theorist
    Commented Jan 1, 2020 at 19:26
6
$\begingroup$

At a fixed temperature and pressure, as in this situation, water has a particular vapor pressure. Below 100C, that's vapor pressure is less than 1atm and we'll restrict ourselves to that case.

"Vapor pressure" means that, in equilibrium, that will be the partial pressure of water vapor in a gas that's in contact with liquid water.

So in the second case, with the special gas present, there will be a small amount of water vapor in with that special gas. The amount will be determined by the vapor pressure, which is determined by the temperature.

What happens in the first case? The vapor pressure is less than the system pressure. Therefore, the liquid water is pushed "up" with more pressure than the gas above (which is all water vapor by construction) pushes "down": The water will rise up to meet the piston with no space between.

Improve this answer
$\endgroup$
1
  • $\begingroup$ See the answer by Chris. I am basically arguing along the lines of your argument and his and cannot make up my mind. $\endgroup$
    – Borun Chowdhury
    Commented Dec 31, 2019 at 21:33
6
$\begingroup$

The phase diagrams you refer to make a number of simplifying assumptions in their construction. In particular, they assume you are dealing with pure substances. In other words, the phase diagram for water is correct only if the piston is filled with water and nothing else.

With an ordinary gas, there would be some degree of gas dissolving into the water and some degree of water vapor in the gas.

With your special gas, it's easy to see there could be no water vapor: by construction, the special gas always has a partial pressure of 1 atm and a total pressure of 1 atm, so the partial pressure of water vapor is zero. That said, such a gas certainly does not exist in nature.

Improve this answer
$\endgroup$
7
  • $\begingroup$ That's what I was thinking but all I assumed was that the special gas does not dissolve in water. Is it sufficient to show that water vapor will not evaporate and mix into the gas as well? I'd rather see a proof of this using Free energies than hand wave but if I get a good argument I can try to make a formal one. $\endgroup$
    – Borun Chowdhury
    Commented Dec 31, 2019 at 21:27
  • $\begingroup$ See the answer by Bob Jacobson. I am basically arguing along the lines of your argument and his and cannot make up my mind. $\endgroup$
    – Borun Chowdhury
    Commented Dec 31, 2019 at 21:32
  • 1
    $\begingroup$ There is no “special gas” that can exclude other molecules. $\endgroup$
    – Bob Jacobsen
    Commented Dec 31, 2019 at 21:53
  • 1
    $\begingroup$ @BorunChowdhury Basically what Bob says. There is no such special gas- any real gas will mix into the water to some degree, and vice-versa. $\endgroup$
    – Chris ♦
    Commented Dec 31, 2019 at 22:20
  • $\begingroup$ So @Chris you guys are saying (and if so then I agree) that applying a pressure of 1 ATM by a piston directly and via the 'special gas that does not dissolve in water' are two different things. In the first case at 50 C and 1 ATM there will be no vapor and in the second there will be. Fine but in the second case how much water will convert into vapor? What is the criteria for finding that? I keep hearing/reading partial pressure of water vapor will be equal to the vapor pressure at 50 C. But why is that the case (assuming that's the answer you were gonna give)? $\endgroup$
    – Borun Chowdhury
    Commented Jan 1, 2020 at 11:16
2
$\begingroup$

Since your edit and new diagram significantly changes the question, I am providing this additional answer to specifically address the two questions in your Edit 2.

What will happen when I remove the partition. Will some of the water in liquid phase convert into vapor phase?

Yes it will. Since the water temperature is below the boiling point at 1 atmosphere, it will convert by evaporation and not vaporization. Vaporization of the water is a phase transition that occurs at the boiling point at 1 atm, which is 100 C. It occurs throughout the liquid as evidenced by the formation of bubbles within the liquid during boiling. Evaporation, on the other hand, occurs at below the boiling temperature at 1 atm. It occurs only at the surface of the liquid. I have already explained the mechanism for evaporation at the molecular level in my previous answer.

If so, how does one figure out how much of the water will convert into vapor?

First, consider when evaporation will cease.

Evaporation will continue until the rate of evaporation at the surface of the liquid equals the rate of condensation of the vapor above the surface, which occurs when the liquid and vapor are in thermodynamic equilibrium. That, in turn, occurs when the vapor pressure of the water vapor above the surface equals the its saturation pressure. The saturation pressure is the pressure that would correspond to the boiling pressure of water at 50 C. From the steam tables, that would be a pressure of the vapor equal to 12.349 kPa absolute. This pressure would then be the partial pressure of gaseous H$_2$0 above the surface in thermodynamic equilibrium.

In case you don't know, the partial pressure of a gaseous component of a mixture of gases is the pressure that that component would exert if it alone occupied the total volume (all other gases removed). Per Dalton's law of partial pressures the total pressure of the gas mix is the sum of the partial pressures. So in this case, evaporation will cease when the partial pressure of the water vapor is 12.2349 kPa. Since total atmospheric pressure at sea level is taken on average to be 101.35 kPa, that means the partial pressure of the ideal gas would be 89.101 kPa in order to total 1 atm.

Now, calculating the mass of the water vapor.

Knowing the partial pressure of the water vapor and the total pressure, we can determine the mass of the water in the gaseous state if we know the mass of the ideal gas and its molecular weight.

First we know that the partial pressure of each gas is the product of the total pressure and the mole fraction of that gas.

The mole fraction of each gas (gaseous H$_2$0 and ideal gas) equals the moles of the gas divided by the total moles of the mixture.

The moles of an individual gas is, in turn, the mass of the gas divided by its molecular weight.

Putting it all together you get the equation shown in the diagram below. From the equation, knowing the required partial pressure of gaseous H$_2$0 at equilibrium, its molecular weight, and the total pressure (1 atm) you can calculate the mass of the water vapor if you have the mass of the ideal gas and its molecular weight.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.