0
$\begingroup$

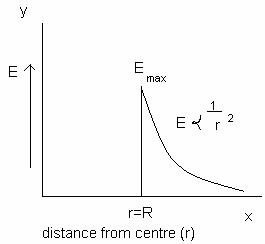
I had studied a lot of Newtonian mechanics and other concepts such as SHMs, Waves and even Thermodynamics but one thing i always noticed was things were continuous and differentiable. All the functions I calculated as answers could be integrated or differentiated to find other parameters however once i started studying Electrostatics and other related concepts i noticed many discontinuous graphs as well. For instance the electric field versus distance graph for a charged spherical shell is discontinuous as you can see in the graph below

enter image description here

Since i had been dealing with only continuous stuff without any breaks it seemed to paint a very smooth picture of the observable nature around me as you don't suddenly see abrupt breaks in the velocity of a particle where it goes from like 0 to 10 without any time lag however these notions tend to start breaking when I dealt with electrostatics.

I know the phrasing of this question might seem a bit weird or philosophical even but my simple question is what does it mean for nature to be discontinuous? I know there a phenomenon which explain why they happen but do these abrupt breaks and non differentiable points in curves reflect some intrinsic properties of the system we are dealing with?

Also in my very short duration of studying actual physics I saw many examples so i reckon there must be much more of these in higher studies. Hence I think they might reflect some fundamental properties of nature or the system but is there any explanation or good reasoning for it being so? Also if it is so prevalent in such systems why is it so that we don't see any of such cases in our day to day life in our immediate surroundings?

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ These discontinuities are not fundamental, but an artifact of the oversimplification. You simply don't have a perfect spherical shell of charge. And insisting that you do have one is exactly the cause of the discontinuity. $\endgroup$
    – Trebor
    Commented Jun 22, 2020 at 6:51
  • $\begingroup$ @Trebor So you mean to say our idealization are the cause of the discontinuities which will be theoretical and cannot be achieved or noticed practically? But aren't these theoretically a part of nature itself us observing them or not is due to our in-capabilities to form ideal systems $\endgroup$
    – FoundABetterName
    Commented Jun 22, 2020 at 7:05
  • $\begingroup$ Indeed. You can try calculating the electric field if the shell is "blurred" slightly (e.g. if the distribution of charge as a function of radius is a gaussian instead of a spike). $\endgroup$
    – Trebor
    Commented Jun 22, 2020 at 7:10
  • $\begingroup$ A spherical shell of charge means the existence of infinitely thin objects, which is impossible since this breaks down at elementary particle level. $\endgroup$
    – Trebor
    Commented Jun 22, 2020 at 7:11
  • $\begingroup$ Oh i got it now also if you'd like to paste these comments into an answer i'll accept it $\endgroup$
    – FoundABetterName
    Commented Jun 22, 2020 at 7:19

1 Answer 1

Reset to default
2
$\begingroup$

These discontinuities are not fundamental, but an artifact of the oversimplification. You simply don't have a perfect spherical shell of charge. And insisting that you do have one is exactly the cause of the discontinuity.

To put it more physically, the theory you are currently learning assumes that charge is continuous, which is true at macroscopic level, because the charge of elementary particles is extremely small. This breaks down when you consider an infinitely thin shell of charge.

As a bonus, you can try calculating the electric field if the shell is "blurred" slightly (e.g. if the distribution of charge as a function of radius is a gaussian instead of a spike). Although I don't think this is doable symbolically, but you can surely use computer software to get a numerical (and graphical) solution. You will see that the discontinuities are smoothed down.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Just one more clarification is needed so does this mean if i get a numerical solution there will be electric field all inside the shell as well and i presume not at the centre due to symmetry or will it be there at the centre too? also what will be the expected deviations (in terms of magnitude of field) from the Gaussian results? $\endgroup$
    – FoundABetterName
    Commented Jun 22, 2020 at 7:31
  • $\begingroup$ @DinoManPhyLab If the whole thing is still spherical, you can still calculate the electric field with the Gaussian theorem, which I suppose you have already learnt. Go ahead and try it! $\endgroup$
    – Trebor
    Commented Jun 22, 2020 at 7:33
  • $\begingroup$ Yes i've learnt to calculate the field with Gauss Law but what i was trying to ask is that are the results obtained by Gauss Law and the other method you mentioned quite similar and if they differ is there a significant value of electric field inside the shell? $\endgroup$
    – FoundABetterName
    Commented Jun 22, 2020 at 9:30
  • $\begingroup$ @DinoManPhyLab Well, Gauss Law is a law, so if you use it correctly the result will be correct. $\endgroup$
    – Trebor
    Commented Jun 22, 2020 at 15:21
  • $\begingroup$ No i mean that Gauss Law assumes symmetry and idealistic conditions which you said aren't possible like infinite point charges making a sphere and proposed a different method which included blurring the shell a bit so i meant to ask do the results from these 2 methods differ? $\endgroup$
    – FoundABetterName
    Commented Jun 22, 2020 at 15:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.