10
$\begingroup$

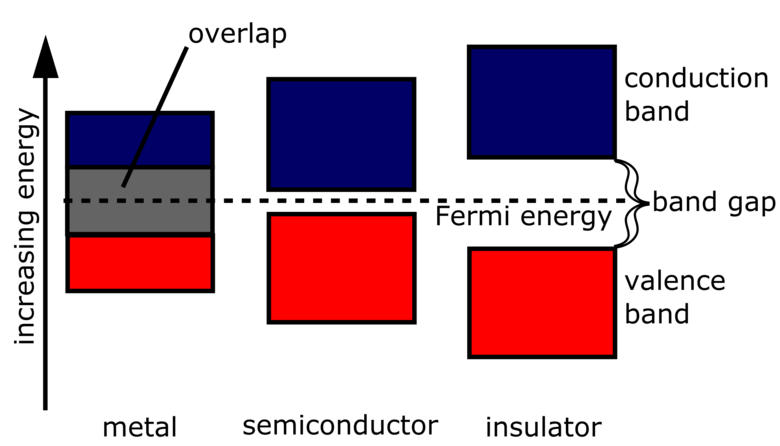
Looking at the periodic table, group II elements like magnesium are known to be metallic, and yet they have full outer shells. So this means they should have full (valence) bands.

Now, last time I checked a completely full band does not conduct.

In a similar way, group IV elements like silicon, germanium, and tin (which is practically a metal!) are actually semiconductors. But, diamond is an insulator.

I am an undergraduate of physics (and a newcomer to this site). I would love to understand the resolution to this problem. I have just started learning solid state physics so my knowledge is somewhat limited.

Why do group II elements conduct and group IV elements not act like semiconductors?

Edit:

Why do people keep using "Fermi energy" and "Fermi level" interchangeably?

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ Potassium is not a group two element. And K and Mg have respectively 1,2 electrons in their outermost shells which act as free electrons allowing conduction. $\endgroup$
    – Archimedesprinciple
    Commented Jun 12, 2020 at 2:54
  • 2
    $\begingroup$ @Archimedesprinciple [Mg has...] "2 electrons in their outermost shells which act as free electrons allowing conduction". This is not true. The shell is full, so conduction should not be possible. Unless the valence and conduction bands overlap as explained below. $\endgroup$
    – N. Gin labs
    Commented Jun 12, 2020 at 5:54

2 Answers 2

Reset to default
16
$\begingroup$

Even when an isolated atom has a filled shell, the electron bands in a solid crystal may be partially filled. The reason is that bands that originate from different atomic orbitals may actually overlap in energy. This is shown on the left in the figure below.

Energy Bands

When the bands overlap, the lowest-energy state has some electrons displaced from the top of the lower band to the bottom of the upper band, so that the Fermi energy in both bands is the same. This leaves partially filled bands, enabling conduction to occur.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Many thanks for your answer, could you show me the website where you got this image? I would like to learn more. $\endgroup$
    – N. Gin labs
    Commented Jun 12, 2020 at 5:21
  • 1
    $\begingroup$ @N.Ginlabs Google search gives this page as a container of this image. Dunno where Buzz actually took it from. $\endgroup$
    – Ruslan
    Commented Jun 12, 2020 at 7:43
  • 1
    $\begingroup$ @Buzz Are you sure the-fermi energy is the right word here? $\endgroup$
    – N. Gin labs
    Commented Jun 18, 2020 at 10:50
  • $\begingroup$ @N.Ginlabs Yes. $\endgroup$
    – Buzz ♦
    Commented Jun 18, 2020 at 17:09
  • 1
    $\begingroup$ @Buzz Well then, let me ask you this: What is the difference between "Fermi energy" and "Fermi level"? $\endgroup$
    – N. Gin labs
    Commented Jul 5, 2020 at 19:52
6
$\begingroup$

Your intuition is probably biased by one-dimensional periodic potential examples of band structures, where it is impossible to have a band level crossing (it would imply the existence of more than 2 independent solutions of a second ordere ordinary differential equation).

In more than 1D things are different and energy levels corresponding to more than two k-points are possible. This is the case of second column elements and also explains the possibility of a range of behaviors for the elements of group IV (Carbon is an insulator in the normal pressure diamond lattice, while Lead is a metal).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.