According to this, the size of the focal spot of sunlight through a lens is f/110, where f is the focal length. A 61" fresnel lens with 86 cm focal length then has a spot which is 7.8mm high, which is quite large. How much smaller can the spot get if you add another lens at the focal spot to focus it further and then maybe another lens in the focal spot of that lens and so on. How small can it theoretically get and how small is it be feasible to get it in practice? Can the spot be focused down to 100 microns, 1 micron?
-
1$\begingroup$ Possibly relevant: en.wikipedia.org/wiki/Etendue Remember that any "spot" of sunlight that you can make using geometric optics will, in fact, be a real image of the Sun. $\endgroup$– Solomon SlowCommented Jun 4, 2020 at 16:35
-
2$\begingroup$ Please note, if you like to focus rays of light onto a "small" spot, you do not use several lenses which are in each another focal plane. $\endgroup$– NotMeCommented Jun 4, 2020 at 20:21
-
$\begingroup$ possibly useful: en.wikipedia.org/wiki/Nonimaging_optics#Theory "Imaging optics can concentrate sunlight to, at most, the same flux found at the surface of the Sun. Nonimaging optics have been demonstrated to concentrate sunlight to 84,000 times the ambient intensity of sunlight, exceeding the flux found at the surface of the Sun, and approaching the theoretical (2nd law of thermodynamics) limit of heating objects to the temperature of the Sun's surface." $\endgroup$– robphyCommented Aug 23 at 16:59
3 Answers
How much smaller can the spot get if you add another lens at the focal spot to focus it further and then maybe another lens in the focal spot of that lens and so on.
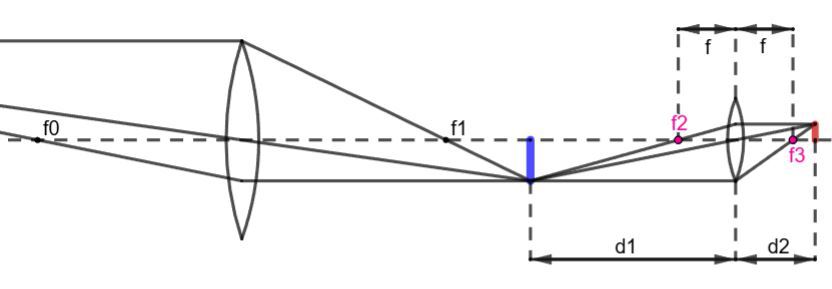
In the diagram above, the Sun is on the left, and rays from it are focused on a smaller real image (blue), and then a second lens uses that real image as the source image and focuses it on an even smaller spot (red).
For a spherical lens the magnification is given by $$ M = \frac{d_2}{d_1},$$
where $d_1$ is the distance from the lens to the source, and $d_2$ is the distance from the lens to the created image.
Using the thin lens approximation for the focal length of a simple lens: $$\frac{1}{f} = \frac{1}{d_1}+\frac{1}{d_2}. $$
This equation can be rearranged to give us $d_2$ in terms of focal length:
$$d_2 = \frac{f \times d_1}{f-d_1}.$$
Substituting this value for $d_2$ into the equation for magnification gives us: $$ M = \frac{f}{f - d_1}. $$
To make the spot as small as possible, we want $M$ to be as small as possible, i.e. de-magnify the source image. To achieve this, $f$ should be as small as possible, and $d_1$ should be as large as possible relative to the focal length. Because the image is real, we can even add another lens to reduce the spot even further, but the returns are probably diminishing.
There are several real-world limitations to this process. Spherical lenses do not focus rays to an exact focus, but they are commonly used as they are simple to manufacture. This is known as spherical aberration.
It is well known that light from the Sun forms a rainbow when passing through a prism and that this separation is caused by different wavelengths of light being refracted by different amounts when passing from one medium to another. This means that a source like the Sun, with multiple wavelengths, cannot have all the light focused to exactly the same spot by a normal simple lens. This is known as chromatic aberration. It is also well known that there is a limit to how much a microscope using visible light can magnify an image without losing resolution due to the wavelengths used. This is related to aperture diffraction. Another form of aberration is Petzval field curvature, which is due to the inability of a basic lens to focus a flat source object to a flat image plane.
Most of the aberrations mentioned above can be compensated for, to a certain extent, by sophisticated lens curvature designs, compound lens designs, special optical materials and special lens coatings. However, for a given wavelength, aperture diffraction is pretty much an unavoidable limitation that ultimately limits how small the spot can be focused.
How small can it theoretically get and how small is it be feasible to get it in practice?
This is determined by the conservation* of etendue. The product $G=A\theta$ is constant where $G$ is the etendue, $A$ is the area, and $\theta$ is the solid angle. Since the maximum solid angle is $4\pi$ and since the solid angle of the sun as seen from earth is $6.8 \ 10^{-5}$ it follows that the smallest area is $$\frac{A_{spot}}{A_{pupil}}=\frac{6.8 \ 10^{-5}}{4\pi}=5.4 \ 10^{-6}$$
This would require focusing from all directions, so is not feasible. But it would be the theoretical minimum. So for a $61"$ lens, $A_{pupil}=2922 \mathrm{\ in^2}$ so the minimum spot would be $A_{spot}=0.016\mathrm{\ in^2}$
*etendue is conserved for refraction and reflection, but not for scattering or diffraction. Those increase etendue such that the area becomes larger.
I've read elsewhere that the minimum is equal to the light's wavelength, due to interference scrambling all the light beams. Visible light's wavelength (according to google) is up to 700nm, so that, I think, is the theoretical limit of how small it can get.
-
$\begingroup$ Your answer could be improved with additional supporting information. Please edit to add further details, such as citations or documentation, so that others can confirm that your answer is correct. You can find more information on how to write good answers in the help center. $\endgroup$– Community BotCommented Mar 19 at 1:45