1
$\begingroup$

I have a conceptual doubt in ideal fluid flow. I have expressed my doubt with the help of the following example.

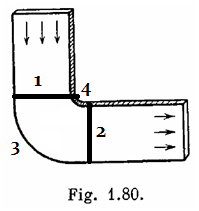
Suppose an ideal fluid flows along a flat tube of constant cross section, in a horizontal plane and bent as shown in the figure below. The flow is ideal and steady.

The question is - All the fluid elements which reach at layer 1, will they reach the layer 2 at the same time or different times?

The doubt comes because, as the fluid enters the layer 1, different fluid elements across a cross section will begin to have different speeds, though before layer 1, they all had the same speed. For example - when the fluid is passing through the bending the pressure at 3 will be greater than the pressure at 4 and velocity at 3 will be smaller than the velocity at 4. The fluid which is near point 3 will first deaccelerate and then accelerate till it reaches layer 2 and the fluid which is near point 4, will first accelerate and then deaccelerate till it reaches layer 2. All this happens because for the fluid to take a turn the necessary centripetal force will be given by the pressure difference which arises between points 3 and 4.

Now, the conceptual question comes, all the fluid elements which reach at layer 1, will they reach the layer 2 at the same time or different times? How do we verify this idea?

Kindly help.

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ According to the Bernoulli equation, the pressure coming into the elbow is equal to the pressure leaving the elbow (assuming it is horizontal). The thing that causes the force on the elbow is the change in momentum (direction) of the fluid. To bring about this change in momentum, the pressure at 3 is higher than at 1 and 2. $\endgroup$
    – Chet Miller
    Commented May 24, 2020 at 12:19
  • $\begingroup$ @ChetMiller - Thank you for your response. I understand that. That is not the original question. My question is, all the fluid elements which reach at layer 1, will they reach the layer 2 at the same time or different times? How do we verify this idea? I request you to Kindly read the question for more details. $\endgroup$
    – Devansh Mittal
    Commented May 25, 2020 at 2:57
  • 2
    $\begingroup$ If they are traveling more slowly near 3 than near 4, and the distance along streamline 3 is longer than along. streamline 4, the time for. particles to transition along 3 will be higher than along 4. You can quantify all this by solving Laplace's equation for the stream function (assuming 2D geometry), and then using it to determine the velocity along the streamlines. $\endgroup$
    – Chet Miller
    Commented May 25, 2020 at 10:59

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.