0
$\begingroup$

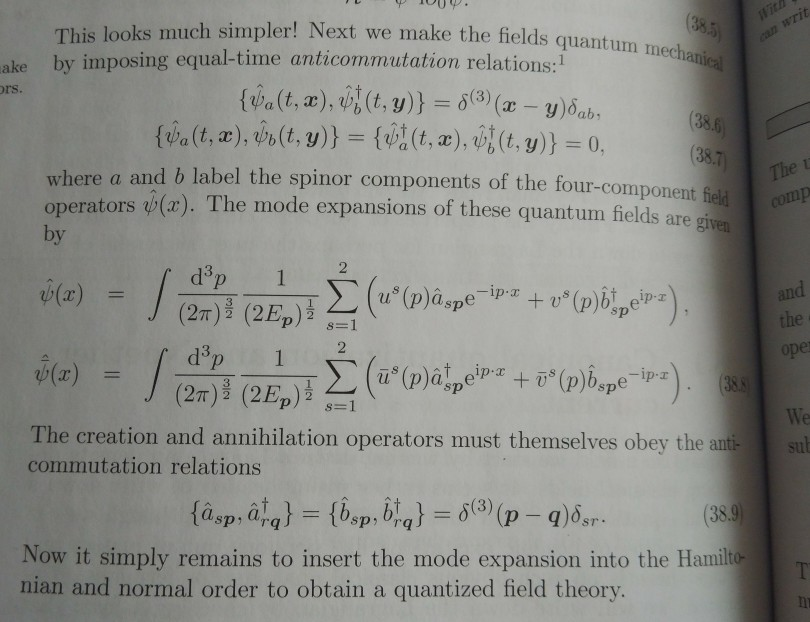
In the attached Quantum Field image we have the mode expansions (for a Dirac field), with annihilation operators (for both particle and antiparticle) attached to an exponential with a (-ikt) while the creation operators are attached to a positive (+ikt).

If we switch these so that the annihilation operators are attached to an exponential with a (+ikt) and the creation operators are attached to a negative (-ikt), we wind up with the following commutation relations:

$$\boxed{[a(k),a^{\dagger}(p)]_{}=[b(k),b^{\dagger}(p)]_{} = -\delta^{3} (k-p),} $$

which varies from the standard commutation relations only by the negative Dirac delta function: $${{-\delta^{3} (k-p).}}$$

Is this fundamentally objectionable? Are there any specific reasons (physical or theoretical) that this wouldn't work.

The same question applies to the use of a complex scalar quantum field instead of the Dirac quantum field.

Quantum Field Operators

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ "In the attached Quantum Field image" - I was quite disappointed when I realized that the image was not of a Quantum Field. $\endgroup$
    – Alfred Centauri
    Commented May 20, 2020 at 16:06
  • $\begingroup$ Does the exponent $(ikt)$ look right to you? $\endgroup$
    – Alfred Centauri
    Commented May 20, 2020 at 17:13
  • $\begingroup$ Also, the time dependence of the operators are determined by the Hamiltonian and the Heisenberg equation of motion: $\dot{\hat{O}} = -i[\hat{O},\hat{H}]$. Is it valid to just switch which operator is associated with which exponential? $\endgroup$
    – Alfred Centauri
    Commented May 20, 2020 at 17:16

1 Answer 1

Reset to default
2
$\begingroup$

Ah, these conventions.

Reminds me of an exam, where I put the wrong sign in the Schrödinger equation. After a long discussion, the professor agreed that you can always replace every $i$ with $-i$. The physics stays the same.

To be consistent, you should then also change the $i$s in $u$ and $v$, and $\bar{\psi}$, probably resulting in positive $\delta$ again.

Think of $i$ not simply as a number, rather as a generator of a phase-transformation $U(1)$. So if you change the sign, it has to be consistent.

Maybe there is yet another convention/choice for the plane wave solutions you use. Could be independent.

Improve this answer
$\endgroup$
2
  • $\begingroup$ DoctorNuu, when you say, "also change the "i" in the a and b," do you mean change the sing of the "k" and "p" such as in a(k) and b(p) in the commutation relation I give above? That is what I presumed. Thanks. $\endgroup$
    – user131744
    Commented May 20, 2020 at 16:20
  • $\begingroup$ Sorry, got (of course) confused again. I meant the spinors $u$ and $v$. And obviously in the inverse transformations from $\psi$ to $a$ and $b$. Also there is a hidden $i$ in $\bar{\psi}$. Only mess with these things if you're really ready for it ;-) And, no, no need to touch the signs of the c-numbers $p$ and $q$ $\endgroup$
    – user257090
    Commented May 20, 2020 at 17:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.