Tight-binding in a semi-infinite square lattice

Question

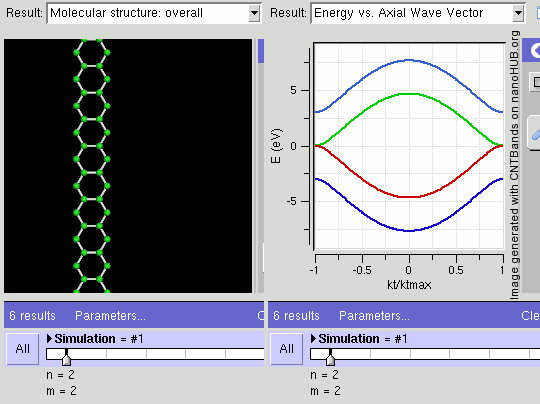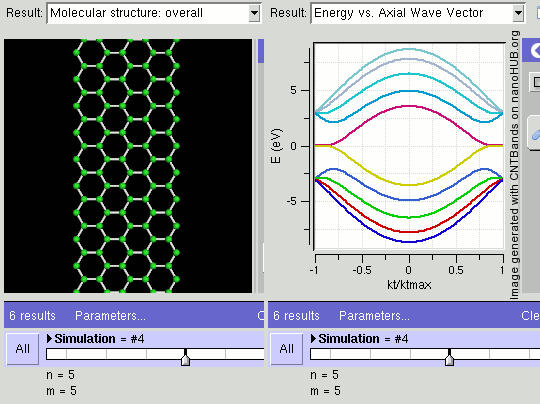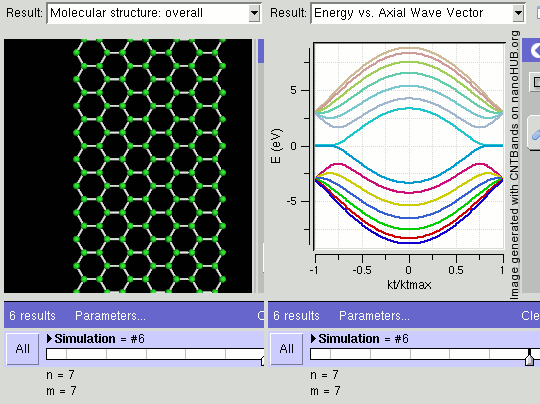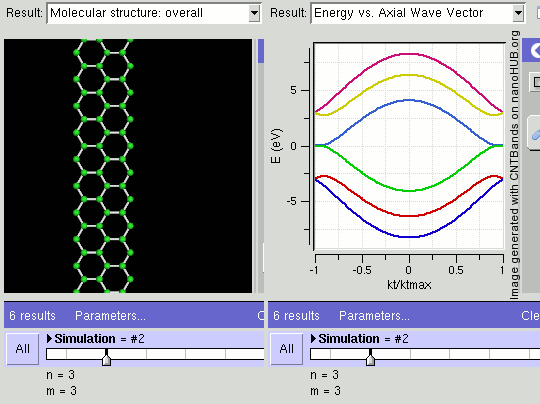
I have a problem understanding how changing the boundaries from a periodic lattice to a finite lattice. For example, if we have a 2D square lattice of lattice constant $a$ whose $x$ axis has only $N_x$ cells with one atom each and no spin degeneracy, and periodic boundary conditions on $y$ with $N_y$ cells, how can we even solve the corresponding Hamiltonian?

Normally, if we had a periodic system in both directions, we would simply use Bloch's theorem to transform our Hamiltonian into momentum space. Nevertheless, since we don't have translation symmetry in the $x$ direction, we can't do that. What other option do we have? Can we use the periodicity of the $y$ direction to use Bloch's theorem somehow?

Answer 1

One way to go about it is, assuming the Hamiltonian is variable separable using Bloch waves for $y$ and doing a brute force $N_x \times N_x$ diagonalisation for $x$. And since tight binding often assumes nearest neighbour hopping, the resulting tridiagonal matrix is easily diagonalisable.

I personally came across this when studying graphene nanoribbon whose treatment can be seen in many books, Chapter 7 of Bernevig’s Topological Insulators and Topological Superconductors for instance.

Gif source: Wikipedia

Answer 2

In the case that you described you still can use the periodicity in $y$ direction. In general, exploiting symmetries of the system is very helpful in quantum mechanical problems: point symmetries, transnational symmetries, timer-reversal symmetry, etc. In order to be really good in this one has to learn the group theory.

The way your problem is formulated is too general to propose a specific approach. If the Schrödinger equation is separable for $x$ and $y$, it will significantly simplify things. Some of one-dimensional problems are known to be exactly solvable, such as a finite Krönig-Penny model, tight-binding Hamiltonian, finite Ising chain or XY model. Finally, if you are interested in the processes happening far from the boundary, you may still assume the periodicity. On the other hand, boundary states in realistic materials is a notoriously hard problem.

Another interesting case is half-infinite periodic medium. I suspect that one could design a solution using periodic symmetry and, e.g., reflection symmetry along the planes parallel to the boundary. I asked a question about it some time ago, but received no clear answer.