0
$\begingroup$

Einstein imagined a man in a moving train who observed lightning which were on the same side of the track and at some distance from each other.

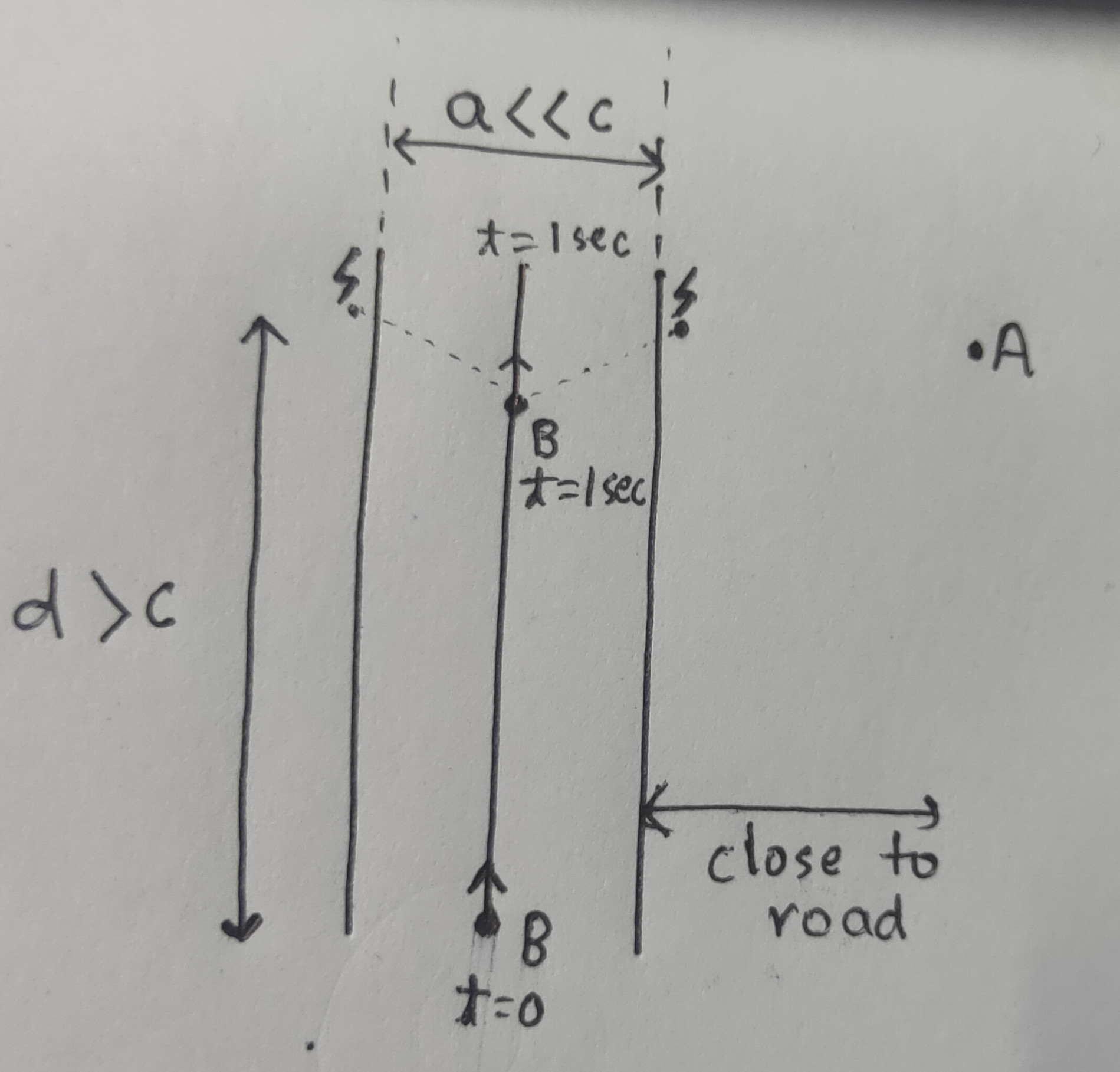
But try to modify the experiment. Let us imagine two men, say A and B. A is standing still on a platform and B is on a bike (whose speed can reach very close to the speed of light instantly). Both of them have their own stopwatch and both start it at the same time (which was the time when B started his ride). But imagine the lightning bolts on opposite side of the road. Since the road is not much broad, it would take same time for both the lights to reach A. But for B the situation might be different. Now let us say that B is driving the bike on the line passing through the midpoint of the road and towards the spot where lightning is about to happen. Suppose he started his journey and at $t = 1s$ the lightning event took place. Since the distance of B from both the lights is same (and he is very close to lightning spot at $t = 1s$), so he will also receive the lights of both the bolts simultaneously. And for both of them this event of lightning is simultaneous.

Note: take the distance of B from the lightning spot to be greater than c i.e. $3×10^8 m$.

So how can we say or prove time dilation in a moving frame or disprove simultaneity using this thought experiment? Am I wrong somewhere?

Avoid mathematical terms and give practical reasons only.

Picture is given below,

enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Could you draw a picture its hard to visualize the situation $\endgroup$
    – seVenVo1d
    Commented May 7, 2020 at 14:56
  • 1
    $\begingroup$ After you have received answers you should never make edits that change the question in a way that invalidates the answers. I have reverted the edit. $\endgroup$
    – Dale
    Commented May 8, 2020 at 4:31
  • 1
    $\begingroup$ To observe the effects of relative simultaneity, the events have to occur in the direction of your motion. In this case, the events are directed along the breadth of the road, which is not in the direction of motion of the bike. That is why you cannot disprove simultaneity using this thought experiment. $\endgroup$
    – PNS
    Commented May 8, 2020 at 4:38
  • $\begingroup$ Do you mean that the lightning should take place on same side of the road? If that is the case then why is it so?? $\endgroup$
    – user262060
    Commented May 8, 2020 at 6:30
  • $\begingroup$ Lightnings are not simultaneous in A: relativity must hold exactly, at least in these kind of thought experiments, so the approximation that : "Since the road is not much broad, it would take same time for both the lights to reach A" is not valid. $\endgroup$
    – Luca M
    Commented May 8, 2020 at 7:42

1 Answer 1

Reset to default
2
$\begingroup$

So how can we say or prove time dilation in a moving frame or disprove simultaneity using this thought experiment? Am I wrong somewhere?

Let’s step away from thought experiments for a moment and look directly at the Lorentz transform. In the usual configuration the x direction is the direction of relative movement and the x’ axis is parallel to the x axis and similarly for the other axes. So the Lorentz transform is:

$$\Delta t’ = \gamma \left(\Delta t -\frac{v \Delta x}{c^2}\right)$$ $$\Delta x’ = \gamma ( \Delta x - v \Delta t)$$ $$\Delta y’ = \Delta y$$ $$\Delta z’ = \Delta z$$

In your scenario x is the direction along the road and y is the direction across the road. Since in your scenario $\Delta t=0$ and $\Delta x=0$ we can plug into the first formula and find $\Delta t’=0$.

So it is expected that both frames agree that they happened simultaneously. This thought experiment is not capable of studying the relativity of simultaneity.

Similarly, for time dilation since $\Delta t’$, $\Delta t$, and $\Delta x$ are all zero then the first equation reduces to $0=\gamma 0$ which is true regardless of $\gamma$ and therefore provides no information about time dilation.

This scenario is set up in a way that it is not sensitive to either time dilation or the relativity of simultaneity. $\Delta x=0$ makes the relativity of simultaneity irrelevant and $\Delta t =0$ makes time dilation irrelevant

Improve this answer
$\endgroup$
6
  • $\begingroup$ So , why is it unable to prove time dilation? From STR time gets slower in moving frame. But here it doesn't happen. Why? $\endgroup$
    – user262060
    Commented May 7, 2020 at 11:35
  • $\begingroup$ @Aakanksha sharma I added another paragraph explaining why it is unable to investigate time dilation also $\endgroup$
    – Dale
    Commented May 7, 2020 at 14:22
  • $\begingroup$ But in all moving frame time should slow down . Why not in this one? $\endgroup$
    – user262060
    Commented May 7, 2020 at 14:29
  • $\begingroup$ This has nothing to do with the frame. It is about the geometry of your setup. Time slows down in this frame and simultaneity is relative in this frame, but your setup is chosen so that neither of those effects matter. They exist, they just don’t impact this setup, as shown $\endgroup$
    – Dale
    Commented May 7, 2020 at 18:42
  • 1
    $\begingroup$ Yes, of course. To get time dilation you need $\Delta t \ne 0$ and to get only time dilation you need $\Delta x = 0$. Comments are not for extended discussion and not for additional questions. If you want to discuss then you should go to a site like physicsforums.com. Your question has been answered $\endgroup$
    – Dale
    Commented May 8, 2020 at 4:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.