Consider the following setup and problem:
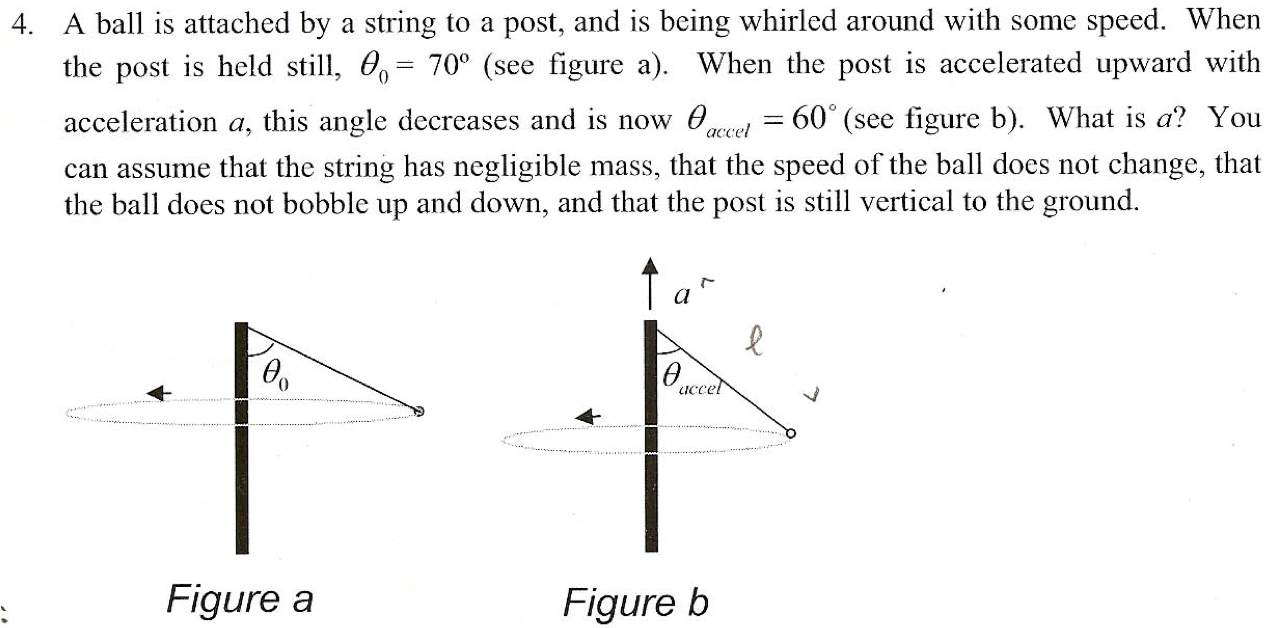
When solving this problem, I had the intuition that the string tension $T_A$ would be equal to $T_B$, which does not agree with the solutions.
What would lead you to know the string tensions in both cases are, in fact, different?
Let's assume that the question is telling us to keep the component of the speed in the plane perpendicular to the central rod constant. Then in the case with upward acceleration, Newton's second law in the $x$ (horizontal) and $y$ (vertical) directions reads \begin{align} T_a\sin\theta_a &= m\frac{v^2}{\ell\sin\theta_a}\\ T_a\cos\theta_a &= m(g+a) \end{align} where $T_a$ is the magnitude of the tension for given upward acceleration $a$ and $\theta_a$ is the corresponding angle. This is two equations in two unknowns $T_a$ and $\theta_a$. Solving for $T_a$ (I used Mathematica out of laziness fyi) $$ T_a = \frac{m v^2}{2\ell}\sqrt{1+\frac{4(g+a)^2\ell^2}{v^2}} $$ Note that for fixed $v, \ell, m$ the tension necessarily increases.
Note. I had previously only analyzed the vertical component of the tension which OP pointed out was not sufficient to answer the question.
Originally the tension in the string only has to offset one vertical force: $mg$. When the post is accelerated, it now has to offset two vertical forces: $mg$ and $ma$.
If you look at the balance of forces on the mass you will notice that $T\,\cos\theta = m\,g$ and since the angle is different, the tension must be different.
