2
$\begingroup$

I'm trying to understand the concept of angular velocity. I read this paragraph on Wikipedia, which asserts that if a point $p$ has angular velocity $u$ within a coordinate frame $F_1$ which itself has angular velocity $v$ within some other frame $F_2$, then the point has angular velocity $u+v$ with respect to the second frame.

I want to set aside the specific formula $u+v$ and just focus on the abstract form $f(u, v)$ - in other words, just the fact that the angular velocity of $p$ with respect to $F_2$ can be deduced from the angular velocities of $p$ with respect to $F_1$ and of $F_1$ with respect to $F_2$. That seems like it ought to be impossible to me. In the diagram below, $C$ has the same angular velocity $u$ with respect to $B$ in both figures and $B$ has the same angular velocity $v$ with respect to $A$ in both figures, yet in the first figure $C$ has angular velocity $u+v$ with respect to $A$, whereas in the second figure $C$ has angular velocity $u-v$, zero if we set $u=v$.

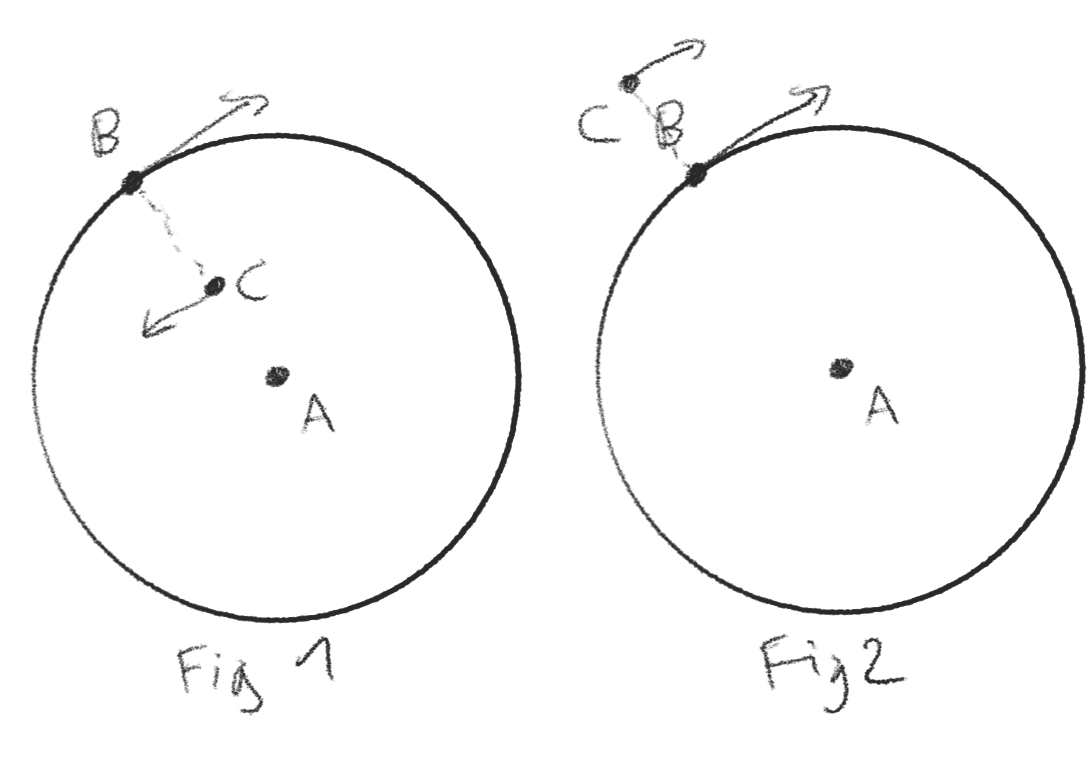
enter image description here

What am I failing to understand?

Improve this question
$\endgroup$
13
  • $\begingroup$ Just note that angular velocity applies to all points on a rigid body and that a single point does not have angular velocity. Also, note that learning from Wikipedia is tricky because it is very inconsistent and sometimes erroneous. $\endgroup$
    – John Alexiou
    Commented Apr 29, 2020 at 12:26
  • $\begingroup$ Can you please explain what the figures represent. There are circles with points and arrows which appear tangential but could indicate out of plane. Where is $u$ and $v$ in the figures? $\endgroup$
    – John Alexiou
    Commented Apr 29, 2020 at 12:28
  • $\begingroup$ @ja72 Everything is happening in a flat plane. $C$ is rotating around $B$, $B$ is rotating around $A$. The arrows attached to $B$ and $C$ represent their velocity vectors and are $u$ and $v$ respectively. $\endgroup$
    – Jack M
    Commented Apr 29, 2020 at 13:06
  • 1
    $\begingroup$ The vectors $u$ and $v$ are described as rotational velocity in the question, but drawn as translational velocities in the diagram. Please clarify. Your question is still not clear to me. I want to help, but I don't know what exactly are you asking. $\endgroup$
    – John Alexiou
    Commented Apr 29, 2020 at 14:38
  • $\begingroup$ @ja72 "Also, note that learning from Wikipedia is tricky because it is very inconsistent and sometimes erroneous." Wiki does contains some errors but compared to other encyclopedic resources it does quite well. $\endgroup$
    – Gert
    Commented Apr 29, 2020 at 14:41

1 Answer 1

Reset to default
4
$\begingroup$

You are asking about the algebra of angular velocities, especially as it relates to the area of kinematics.

Consider the tea-cup ride at Disney. You have a platter (orange) rotating about A with angular speed $\Omega$. At some radius $R$ away form the center a 2nd object (green) is pinned at point B with relative speed $\dot \theta$. This is to denote that $\theta$ is an orientation angle. On this object there are two attached points, C and D, that at some instance in time are located as shown below:

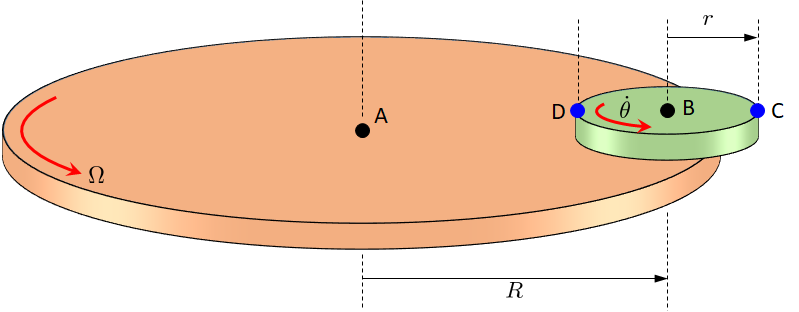
teacups

  • What is the rotational velocity of A?

    Every particle attached to the platter moves with translational velocity except the center of rotation. Nevertheless we can state that the body rotates with $\Omega$ and the translational speed of each particles is given by $$ v= R\, \Omega $$ where $R$ is the radial distance from the center of rotation. You can say that B rotates about A, but in reality the entire body is rotating about A. A more accurate description would be that B orbits A.

  • What is the rotational velocity of B?

    This depends on which body is B belong to. The bottom of the pin connecting the two bodies rotates with $\Omega$ and the top of the pin with $$\omega = \Omega + \dot\theta$$ since $\dot \theta$ is the relative motion between the two bodies.

  • What is the translational velocity of points A, B, C and D.

    • $v_A = 0$

      since it is on a fixed point

    • $v_B = R\,\Omega$

      as we saw before

    • $v_C = v_B + \omega \, r = \Omega \,R + (\Omega + \dot \theta) \, r = \Omega ( R+r) + \dot \theta \, r $

      To be interpreted as the velocity of the platter at C plus the relative velocity of the second body.

    • $v_D = v_B - \omega \, r = \Omega \,R - (\Omega + \dot \theta) \, r = \Omega ( R-r) - \dot \theta \, r $

      To be interpreted as the velocity of the platter at C minus the relative velocity of the second body.

As you can see, relative translational velocities switch signs between C and D. This is because the position of the point is either radially out or radially in from the reference point B.

On the contrary rotational velocity of the second body is only added the motion of the platter, because the location of the pin at B bears no significance in the rotational kinematics.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thanks very much. I don't understand the equation $\omega=\Omega+\dot\theta$. What exactly is $\omega$? I don't understand how it can be the rotational velocity of the top of the pin, which is surely just $\Omega$. $\endgroup$
    – Jack M
    Commented Apr 29, 2020 at 21:56
  • $\begingroup$ Ok we are getting somewhere now. $\omega$ is the rotational velocity of the green body. That is if you want to find the translational velocity of C relative to B you need to do $$ v_C - v_B = \omega \, r $$ Rotational velocities add vectorially, and in this case you add the green body rot. velocity to the relative rot. velocity to get the rot. velocity of the green body. Or otherwise the relative rot. velocity is $$ \dot \theta = \omega - \Omega$$ $\endgroup$
    – John Alexiou
    Commented Apr 29, 2020 at 22:55
  • $\begingroup$ So just to make sure I understand,are $\omega$, $\Omega$ and $\dot\theta$ all vectors? And the expression $\omega r$ in your previous comment is to be interpreted as a cross product, where $r=C-B$? $\endgroup$
    – Jack M
    Commented May 1, 2020 at 11:36
  • $\begingroup$ Yes, The true 3D kinematics of a pin joint where $B^\star$ is the bottom of the joint (riding on orange body) is $$ \vec{\omega} = \vec{\Omega} + \hat{z} \dot \theta $$ and $$\vec{v}_B = \vec{v}_{B^\star}$$ $\endgroup$
    – John Alexiou
    Commented May 1, 2020 at 12:49
  • $\begingroup$ But then surely the correct equation should be $v_C-v_B=\dot\theta\hat z\vec r$, no? Isn't the point $C$ orbiting the point $B$ at $\dot\theta$ radians per second? $\endgroup$
    – Jack M
    Commented May 1, 2020 at 13:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.