Angular velocity calculation

Question

The statement of the exercise is as follows: The system in the figure consists of a disk of radius R and a bar AB of length 4R, with no slipping between them. The disk rolls and slides on the floor, while the bar AB is hinged at the fixed point A with OA = R and rests on the disk. Use θ as a coordinate.

I understand that $$ w_{bar}= \dot{\theta} $$ however the alleged result of the angular velocity of the disk is $$w_{d} = \dot{\theta} tan^2{\theta}$$ and I don't understand how you get that result.

Answer 1

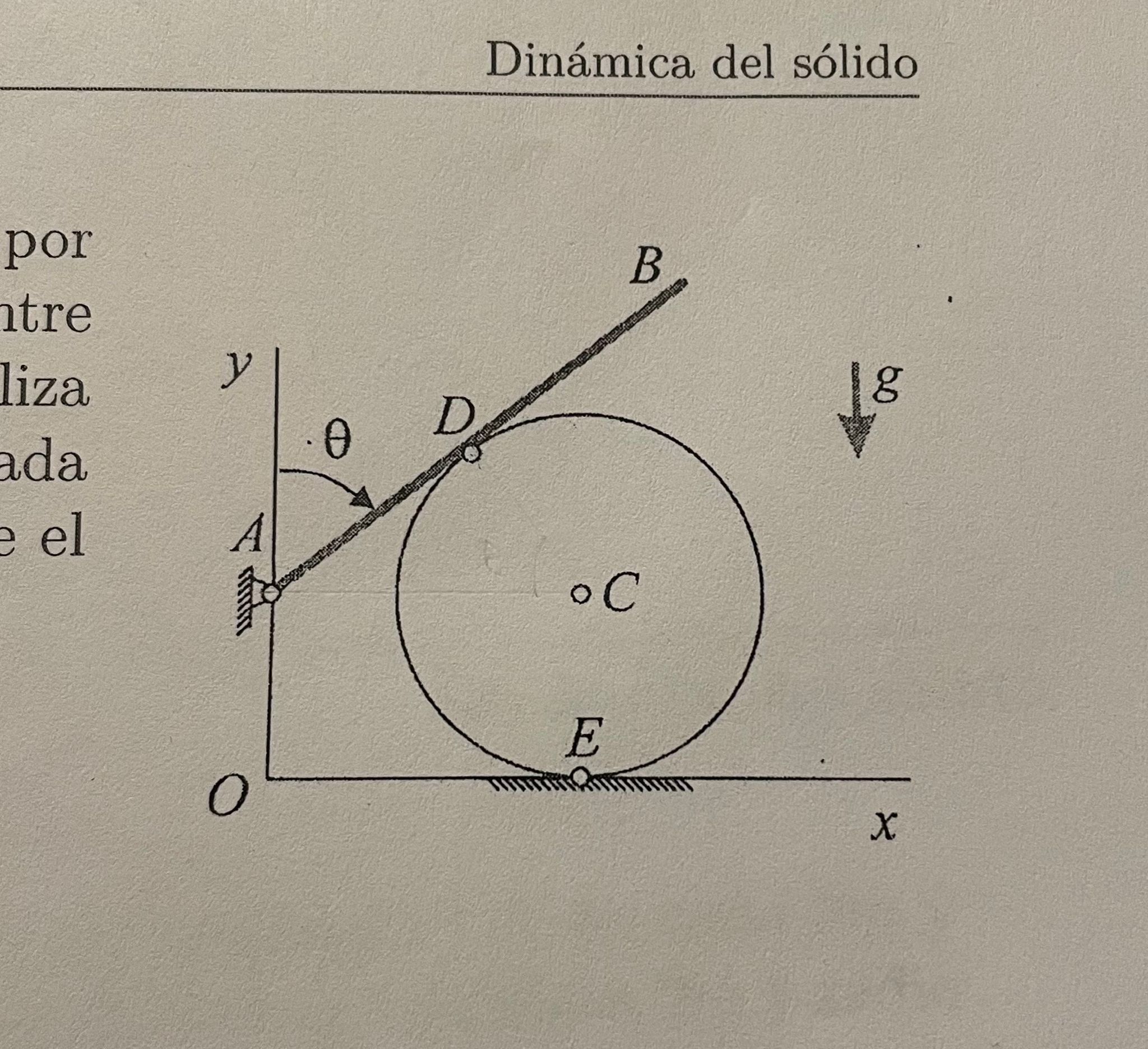
The kinematics of this system at this instant are as follows:

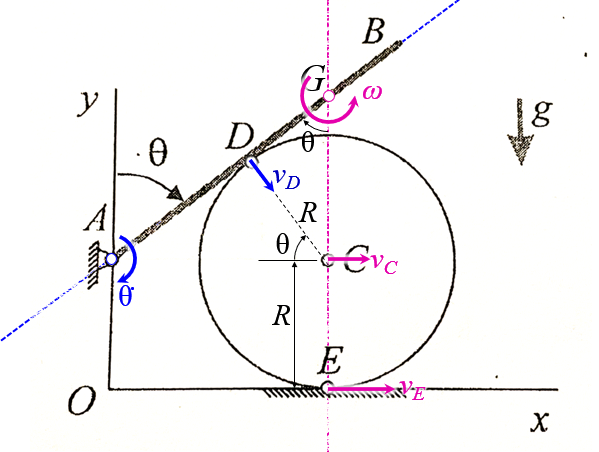
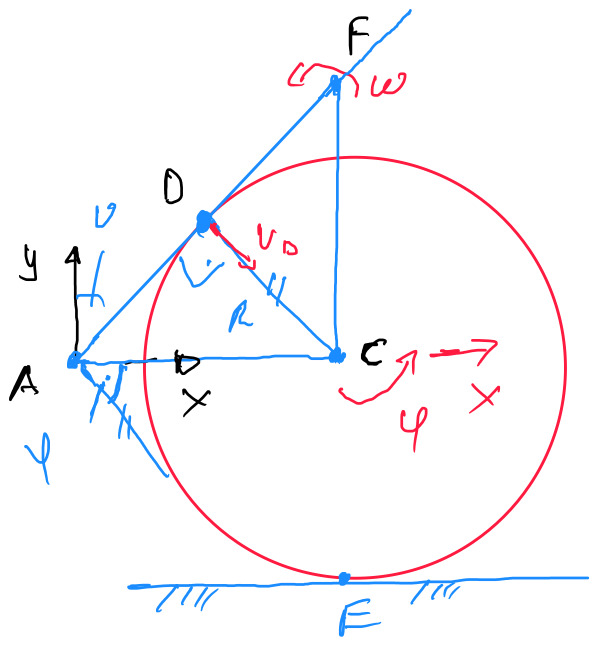
As the bar angle $\theta$ is moving clockwise with speed $\dot{\theta}$, the point of contact D moves with speed $v_D$ as indicated above with the blue arrow towards the center of the circle (since there is no slipping).

The circle translates to the right and rotates counter-clockwise with the instant centre of rotation at the point indicated above as G. This is because the rod has center of rotation at A and the relative rotation center between the rod and the circle is at D, and thus the center of rotation of the circle is constrained along the blue dashed line that connects the two. At the same time, the floor imposes a constraint that velocities at the contact point E must be horizontal only (slip condition) which forces the center of rotation to be somewhere on the vertical magenta line.

Where the dashed blue and dashed magenta lines intersect is where the center of rotation of the circular body is, and I designated this point as G.

With the above geometry, you have the following kinematic relationships

• $v_D = d_{\rm AD} \dot{\theta}$
• $v_D = d_{\rm DG} \omega$
• $v_C = d_{\rm GC} \omega$
• $v_E = v_C + R \omega$

where $d_{\rm AD} = R \tan \theta$ is the distance between A and D, $d_{\rm DG}=R \cot \theta$ is the distance between D and G, and $d_{\rm GC} = \frac{R}{\sin \theta}$ the distance between G and C.

The rotation $\omega$ of the circular object is thus found from the two first equations for $v_D$ by substituting the distances and finding that $v_D = R \tan (\theta) \dot{\theta} = R \cot (\theta) \omega$ which is solved for $$\omega = (\tan^2 \theta) \dot{\theta}$$

and the resulting velocities

$$v_D = \tfrac{R}{\tan \theta} \omega $$ $$v_C = \tfrac{R}{\sin \theta} \omega $$ $$v_E = \left( \tfrac{R}{\sin \theta} + R \right) \omega$$

Answer 2

Point F is the Instant center of rotation this means that $~v_D=\omega\,FD~$

starting with

$$\vec R_{AD}=AD\,\begin{pmatrix} \sin(\theta) \\ \cos(\theta) \\ \end{pmatrix}$$ $$\vec R_{DC}=R\,\begin{pmatrix} \cos(\phi) \\ -\sin(\phi) \\ \end{pmatrix}$$

from the condition $~\vec R_{AD}\perp\vec R_{DC}~$ you obtain that $~\phi=\theta~$. from the rolling condition you obtain that $~x=R\,\phi~$

with

$$AC=\frac{R}{\sin(\pi/2-\theta)}$$ $$FC=AC\tan(\pi/2-\phi)$$ $$AF=\frac{AC}{\cos(\pi/2-\phi)}$$ $$AD=\frac{R}{\tan(\pi/2-\phi)}$$ $\Rightarrow$ $$v_D=\omega\,FD\\ AD \dot\theta=\omega\,(AF-AD)\\ \frac{R}{\cot(\theta)}\,\dot\theta=\omega\,\cot(\theta)\,R$$

$$\omega=\tan^2(\theta)\dot\theta$$