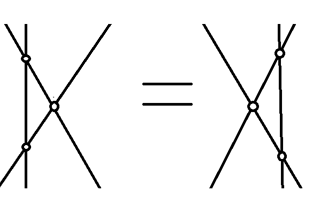
I'm a graduate student in mathematics, and I have lately been interested in the relation between knot theory and statistical mechanics. As I understood, the Yang-Baxter equation (shown below) is the equivalent of the Reidemeister III move (RIII), and it appeared as a convenient hypothesis to solve lattice models such as the Ising model.
What I can't find anywhere though is any kind of convincing physical interpretation of the Yang-Baxter equation. As I have little physics education, I'm not looking for the whole technical story, but rather some convincing argument that it is a sensible relation to assume. Here is one I came up with:
Thinking of the three strands of RIII as the trajectories of three particles $p_1$, $p_2$ and $p_3$, the RIII move means that It does not matter in which order particles interact: either in the order $(p_1,p_2)$, $(p_1,p_3)$ and $(p_3,p_2)$ or in the order $(p_2,p_3)$, $(p_1,p_3)$ and $(p_1,p_2)$.
This is highly unconvincing: why is there a relation between this orders, and not other orders? How is the topology of the crossings (whether crossings are positive or negative) taken into account? So my question is: do you have a better motivation for assuming the Yang-Baxter equation?
NB: as an example of a simple motivation, I convinced myself that the $q$-Potts model ($E\sim\sum_{(i,j)} \delta(s_i,s_j)$, where $s_i$ is the state of vertex $i$ and the sum is over all edges in the lattice) was sensible by thinking of magnets whose positions are fixed at some points: they lowest energy state is the one where all poles are in opposite direction, justifying the term $\delta(s_i,s_j)$.
Question following Yu-v answer. (Sorry to keep asking, but I feel I haven't get the point yet.) The part I don't get is
As each of this orderings must have a well-defined amplitude, different ways to relate them to each other must agree.
Here is my understanding of the situation : the system is the superposition of the solutions of each ordering. Moreover, we have a way to relate the solutions of an ordering $S_{12}$ to the solution of the ordering $S_{21}$ obtained from $S_{12}$ by switching the particles 1 and 2. Call this relation $R$ and write $S_{12}\cong_R S_{21}$ (here I just mean that there is a one-to-one correspondence between the solutions). $S_{21}$ is not defined from $S_{21}$ by $R$: all solutions exist on their own, and it just so happened that there is a relation between them.
Now we can define relations $R_1$ and $R_2$ between $S_{123}$ and $S_{321}$ according to the two ways of reordering the particles 1, 2 and 3: $S_{123}\cong_{R_1} S_{321}$ and $S_{123}\cong_{R_2} S_{321}$. Only considering what I just said, there is no reason to think that $R_1=R_2$. For example, you can have different isomorphisms between two vector spaces: having different isomorphisms does not prevent the vector spaces from being well-defined. I deduce that I'm missing an essential point.