I'm confused about the scattering mechanism in the Integer Quantum Hall effect. I often read the statement, that at a hall plateaux, the particles can't scatter, since an integer number of Hall plateaux is occupied, so the particles have no available state to scatter into. So then I don't understand the origin of the hall resistivity. Why do we always have non-zero hall resistivity, even if there is no scattering allowed. The origin of the hall resistivity is scattering, right?
Greetings
In short
The origin of the hall resistivity is scattering, right?
No. The origin of the plateaux in the Hall resistivity/conductance is due to the lack of scattering because of dissipationless flow. Otherwise you'd have the normal (non quantum) Hall effect. $R_H$ just arises from a transverse voltage because of charge accumulation in the direction $\perp$ to the current flow.
Why do we always have non-zero hall resistivity, even if there is no scattering allowed.
Because the Hall resistance is defined as $R_H = V_H/I$, where $V_H$ is the transverse voltage but $I$ is the "normal" current. Hall resistance along $y$ does not impede the current to flow along $x$.
In depth
As a reminder, here is the plot of the Hall (transverse) resistance $R_H$ and the longitudinal resistance $R_x$.
The battery leads are along the $x$ (longitudinal) direction. So if there are free carriers, the current $I$ will be along $x$. The flow is said to be dissipationless if $R_x = 0$.
The transverse resistance $R_H$ is just defined as $V_H/I$, where $V_H$ is the transverse voltage. The larger the current, the more charge accumulation in the transverse direction and hence the larger $V_H$, which in turns increases the value of $R_H$.
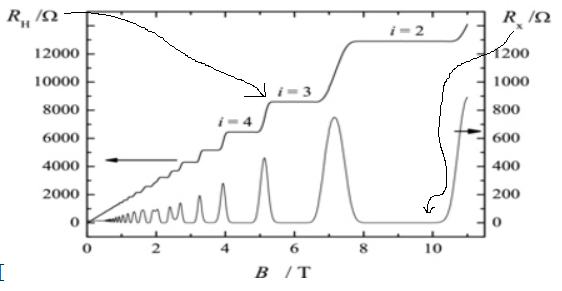
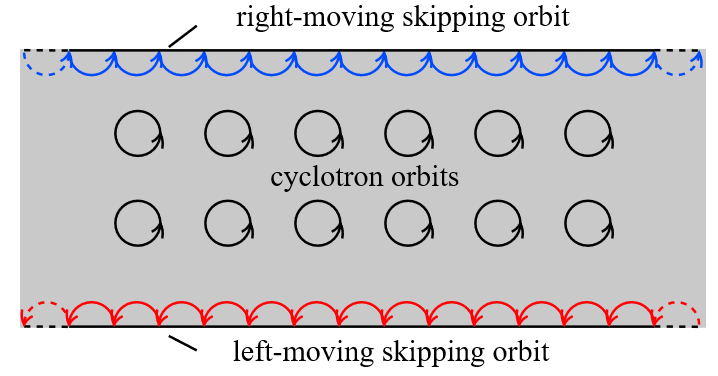
At the plateaux of $R_H$, the Landau levels are filled. There are no available states for particles to scatter into. They can only do the skipping orbit at the edge, which is hence dissipationless, hence why $R_x = 0$.
At the jump, Landau levels are not full. More final states are available for the electrons, so they can scatter in random directions. This introduces dissipation in the "usual" ohmic way. Hence why $R_x \neq 0$ at the jumps.
