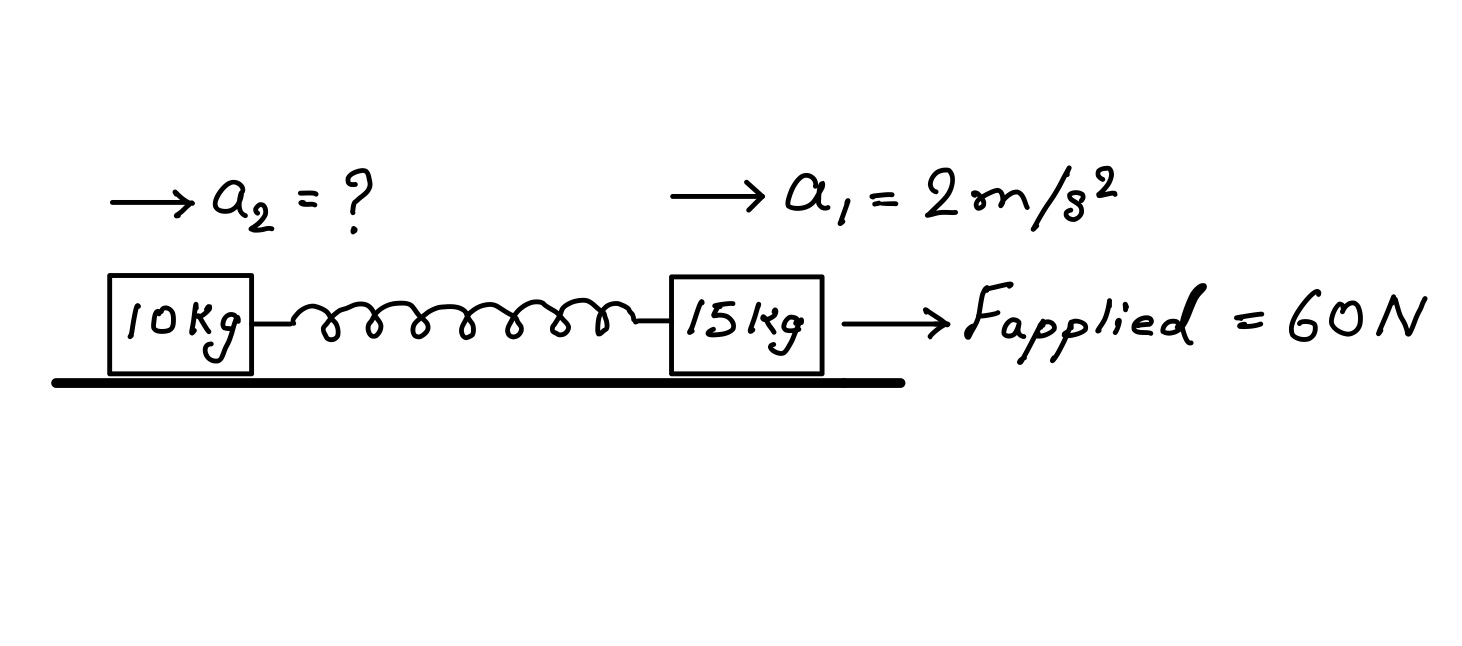
Two masses connected by a massless spring, on a frictionless surface , and a force of $60$N is applied to the 15kg mass such that it accelerates at 2 $\frac{m}{s^2}$. What is the acceleration of the $10kg$ mass?
I came across this question. I first thought that that the $10$kg was constrained to move at the same acceleration. But when I work it out, I get $a_2$ = 3 $\frac{m}{s^2}$. And it is the correct answer according to the book.
What I am unable to understand is, isn’t the $10$kg mass constrained to move at the same acceleration as the $15$kg mass? I thought we could replace the massless spring by (or treat it as) a massless string and results would be the same. Am I making a fundamental mistake?